Question

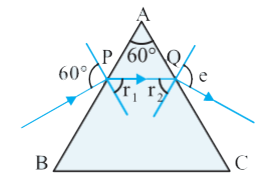
A ray of light incident on an equilateral triangular glass prism of $\mu =\sqrt{3}$ moves parallel to the base of the prism inside it. What is the angle of incidence for this ray ?

Explanation

To solve the problem, we need to find the angle of incidence for a ray of light striking an equilateral triangular prism with a refractive index (μ) of √3, such that the ray moves parallel to the base of the prism inside it.

1. Understand the Geometry of the Prism:
- An equilateral triangle has all angles equal to 60 degrees. Therefore, each angle of the prism is 60 degrees.

2. Identify the Incident Ray and Refraction:
- Let the incident ray strike the prism at point A and make an angle of incidence \( i \) with the normal at that point.
- After refraction, the ray inside the prism is parallel to the base BC of the triangle.

3. Determine the Angle of Refraction:
- Since the ray inside the prism is parallel to the base BC, the angle of refraction \( r \) at point A must be 30 degrees. This is because the angle at the base (60 degrees) minus the angle of refraction (30 degrees) gives us the angle with the normal (90 degrees).

4. Apply Snell's Law:
- According to Snell's law:
\( n_1 \sin(i) = n_2 \sin(r) \)
- Here, \( n_1 \) (the refractive index of air) is 1, \( n_2 \) (the refractive index of the prism) is √3, and \( r = 30^\circ \).

5. Substitute the Values into Snell's Law:
- Substitute the known values into Snell's law:
\( 1 \cdot \sin(i) = \sqrt{3} \cdot \sin(30^\circ) \)
- Since \( \sin(30^\circ) = \frac{1}{2} \), we have:
\( \sin(i) = \sqrt{3} \cdot \frac{1}{2} \)
- This simplifies to:
\( \sin(i) = \frac{\sqrt{3}}{2} \)

6. Find the Angle of Incidence:
- The angle \( i \) for which \( \sin(i) = \frac{\sqrt{3}}{2} \) is:
\( i = 60^\circ \)

Final Answer:
The angle of incidence \( i \) is \( 60^\circ \).

---