How is a wavefront defined? Using Huygen's construction , draw a figure showing the propagation of a plane wave refracting at a plane surface separating two media. Hence verify Snell's law of refraction.
Step-by-Step Solution:
Step 1: Define Wavefront
A wavefront is defined as the locus of all points in a medium that are vibrating in the same phase of a wave. In simpler terms, it is an imaginary surface that connects all the points that are at the same stage of their oscillation.
Step 2: Huygens' Principle
According to Huygens' principle, every point on a wavefront can be considered as a source of secondary wavelets. The new wavefront at a later time can be constructed by drawing a tangent to these wavelets.
Step 3: Draw the Initial Wavefront
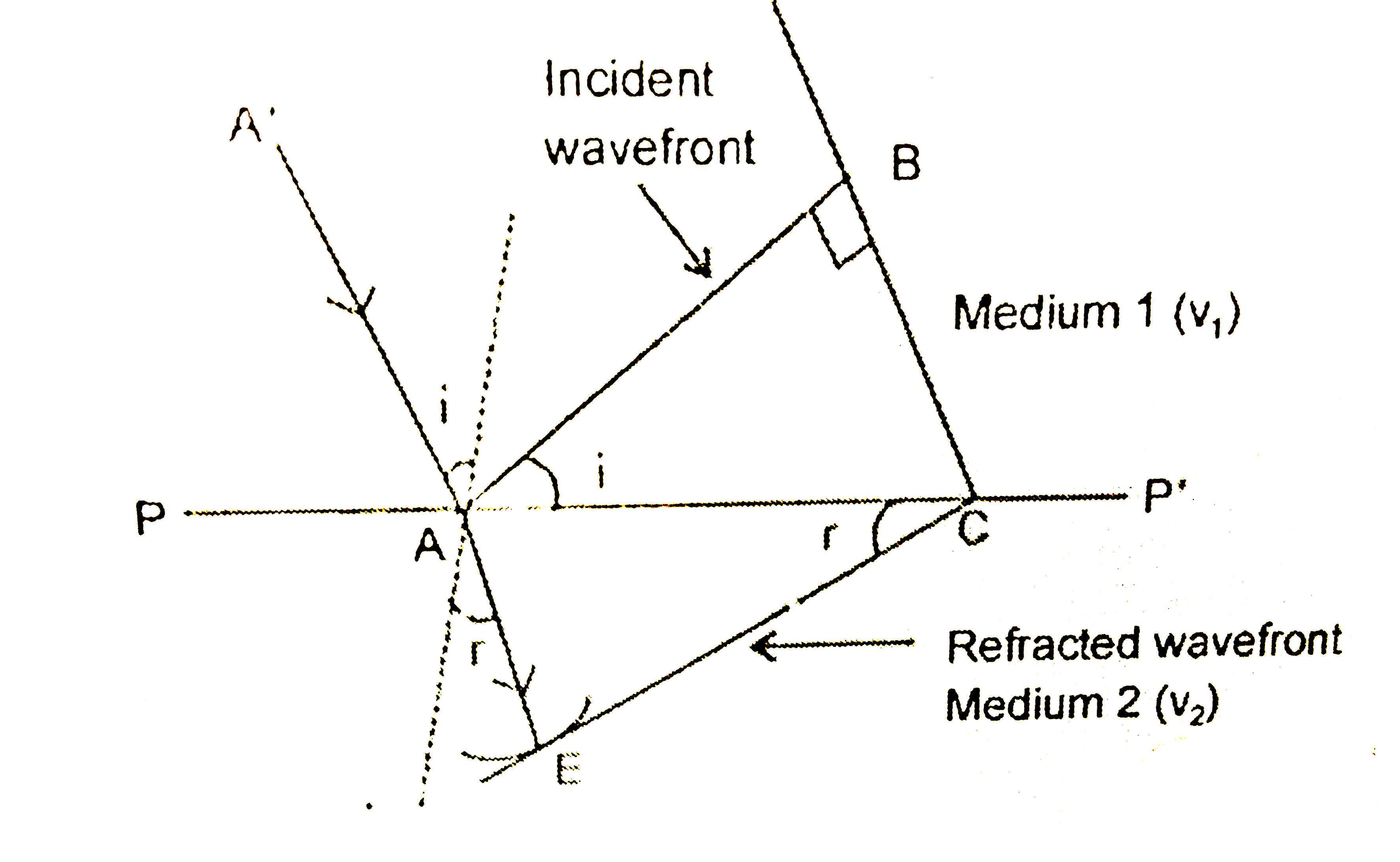
Consider a plane wavefront AB traveling from medium 1 (with speed V1) into medium 2 (with speed V2). At time t=0, the wavefront is represented by a straight line AB.
Step 4: Propagation of the Wavefront
After a time t, point A moves to point A' in medium 1 and point B moves to point B' in medium 2. The distances traveled are:
- AA′=V1t in medium 1
- BB′=V2t in medium 2
Step 5: Construct Right Triangles
From the points A, A', B, and B', we can form two right triangles:
1. Triangle ABB' where:
- AB′ is the hypotenuse
- ∠I is the angle of incidence
2. Triangle AA'B where:
- AA′ is the opposite side
- ∠R is the angle of refraction
Step 6: Apply Trigonometric Ratios
Using the definitions of sine in the right triangles:
- For triangle ABB':
sinI=BB′AB′
where BB′=V1t.
- For triangle AA'B:
sinR=AA′AB′
where AA′=V2t.
Step 7: Relate the Two Equations
From the two equations, we can express:
sinIsinR=BB′AA′=V1tV2t
The t cancels out, leading to:
sinIsinR=V1V2
Step 8: Conclusion
This relationship is known as Snell's Law of Refraction, which states:
sinI/sinR=V1/V2
Thus, we have verified Snell's law using Huygens' construction.
---
A wavefront is defined as the locus of all points in a medium that are vibrating in the same phase of a wave. In simpler terms, it is an imaginary surface that connects all the points that are at the same stage of their oscillation.
According to Huygens' principle, every point on a wavefront can be considered as a source of secondary wavelets. The new wavefront at a later time can be constructed by drawing a tangent to these wavelets.
Consider a plane wavefront AB traveling from medium 1 (with speed V1) into medium 2 (with speed V2). At time t=0, the wavefront is represented by a straight line AB.
After a time t, point A moves to point A' in medium 1 and point B moves to point B' in medium 2. The distances traveled are:
- AA′=V1t in medium 1
- BB′=V2t in medium 2
From the points A, A', B, and B', we can form two right triangles:
1. Triangle ABB' where:
- AB′ is the hypotenuse
- ∠I is the angle of incidence
2. Triangle AA'B where:
- AA′ is the opposite side
- ∠R is the angle of refraction
Using the definitions of sine in the right triangles:
- For triangle ABB':
sinI=BB′AB′
where BB′=V1t.
sinR=AA′AB′
where AA′=V2t.
From the two equations, we can express:
sinIsinR=BB′AA′=V1tV2t
The t cancels out, leading to:
sinIsinR=V1V2
This relationship is known as Snell's Law of Refraction, which states:
sinI/sinR=V1/V2
Thus, we have verified Snell's law using Huygens' construction.
Topper's Solved these Questions
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SET I
Explore 54 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SET II
Explore 8 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
Using Huygen's construction, draw a figure showing the propagation of a plane wave reflecting at the interface of the two media. Show that the angle of incidene is equal to the angle of reflection.
View SolutionUsing Huygen's construction, draw a figure showing the propagation of a plane wave reflecting at the interface of the two media. Show that the angle of incidence is equal to the angle of reflection.
View SolutionUse Huygen's principle to show how a plane wavefront propagates from a denser to rarer medium. Hence verift snell's law of refreaction.
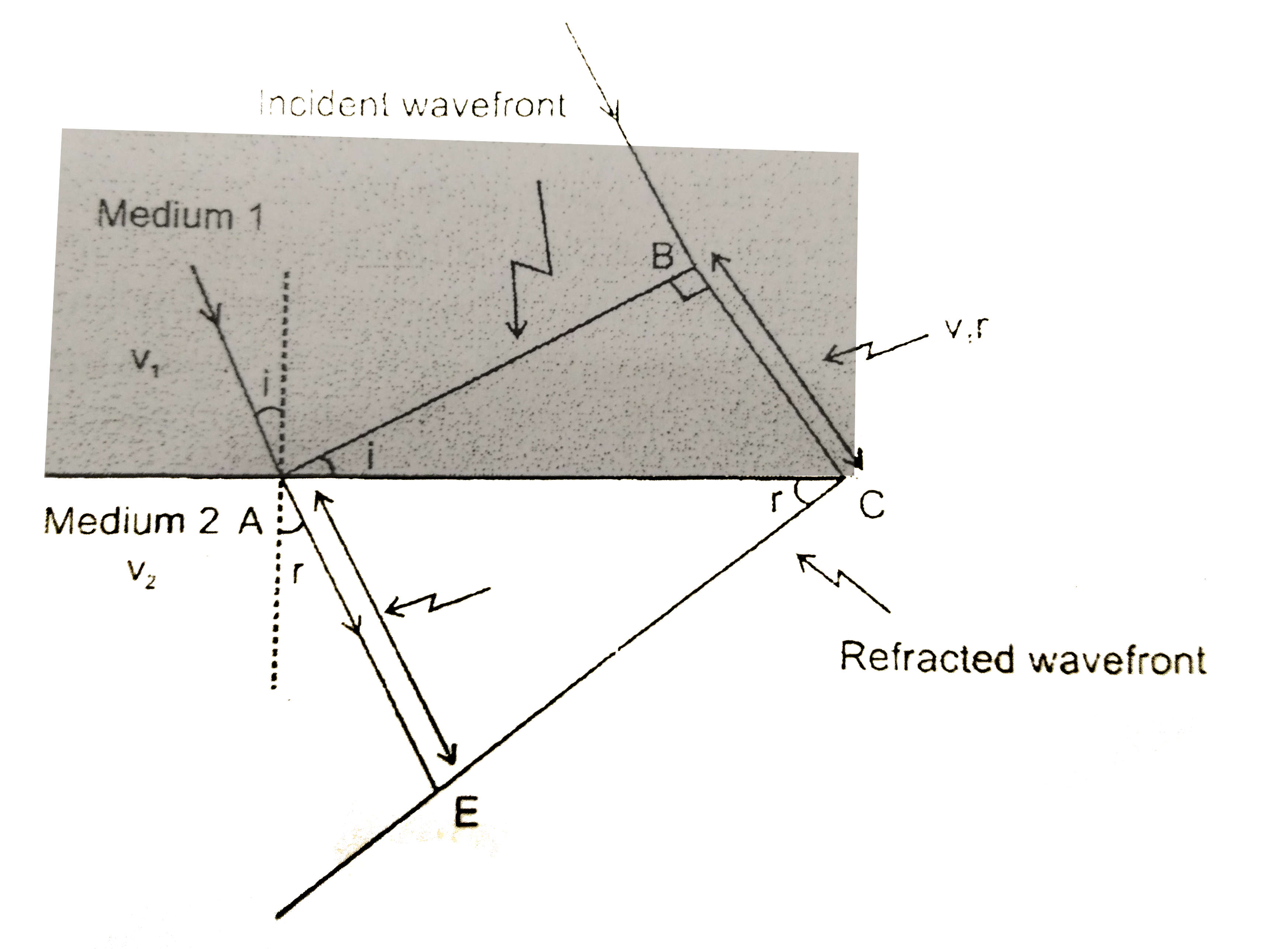
View SolutionDefine the term wavefront. Using Huygens' wave theory, verify the laws of reflection.
View SolutionA plane wavefront propagating from a rarer into a denser medium is incident at an angle of incidence i on a refracting surface. Draw a diagram showing incident wavefront and refracted wavefront . Hence verify Snell's laws of refraction.
View SolutionThe diagram, given below, shows the refracton of a plane wavefront, incident on the surface PP' separating two media, (1)and(2). Which of the media is the rarer one ?
View Solution(a) State Huygen's principle . Using this principle draw a diagram to show how a plane wave front incident at the interface of the media gets refracted when it propagates from a rarer to a denser medium. Hence verify Snell's law of refraction.
(b) When monochromatic light travels from a rarer to a denser medium, explain the following, giving reasons-
(i) Is the frequency of reflected and refracted light same as the frequency of incident light?
(ii) Does the decrease in speed imply a reduction in the energy carried by light wave?
View SolutionDraw a diagram to show refraction of a plane wave front incident in a convex lens and hence draw the refracted wave front
View SolutionAnswer the following:
(a) What is a wave front? How does it propagate? Using Huygens' principle, explain reflection of a plane wavefront from a surface and verify the laws of reflection.
(b) A parallel beam of light of wavelength 500nm falls on a narrow slit and the resulting diffraction pattern is obtained on a screen 1m away. If the first minimum is formed at a distance of 2.5mm from the centre of screen, find the
(i) width of the slit, and
(ii) distance of first secondary maximum from the centre of the screen.
View SolutionUsing Huygen.s principle, verify Snell.s law of refraction of plane wave propagating from denser medium to rarer medium.
View Solution