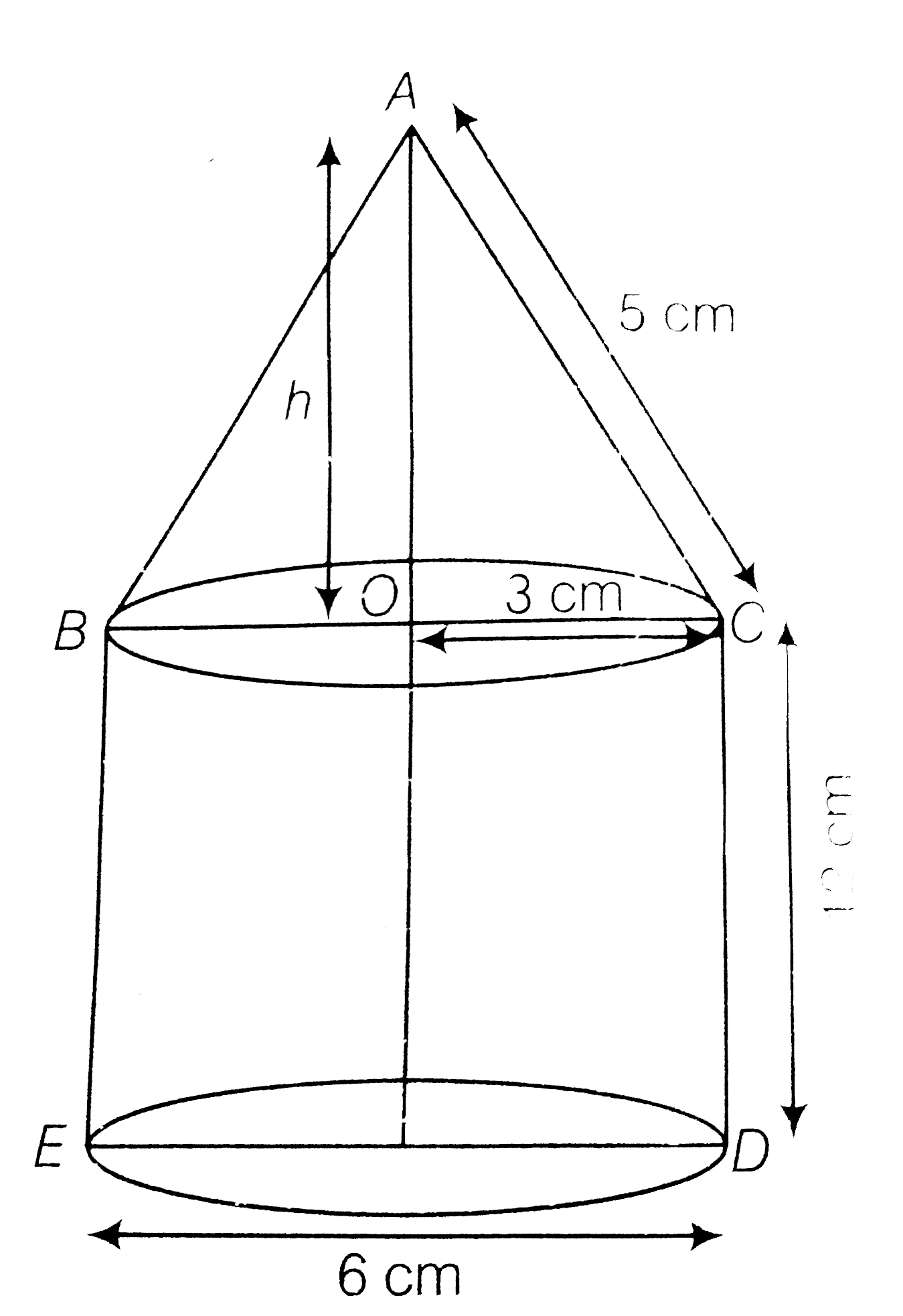
A rocket is in the form of a right circular cylinder closed at the lower end and surmounted by a cone with the same radius as that of the cylinder. The diamter and height of the cylinder are 6 cm and 12 cm , respectively. If the slant height of the conical portion is 5 cm, the find the total surface area and volume of the rocket. ( use π=3.14).
Since, rocket is the combination of a right circular cylinder and a cone.
Given, diameter of the cylinder = 6 cm
∴ Radius of the cylinder = 12 cm
and height of the cylinder =πr2h=3.14×(3)3×12
=339.12cm3
and height of the cylinder = 12 cm
∴ Volume of the cylinder =πr2h=3.14×(3)3×12
=339.12cm3
and curved surface area =2πrh
=2×3.14×3×12=226.08
Now, in right angled ΔAOc
h=√52−32=√25−9=√16=4
∴ Height of the cone h = 4 cm
Radius of the cone ,r= 3cm
Now, volume of the cone
=13πr2h=13×3.14×(3)2×4
=113.043=37.68cm3
and curved surface area =πrl=3.14×3×5=47.1
Hence, total volume of the rocket=339.12+37.68=376.8cm3
and total surface area of the rocket = CSA of cone + CSA of cylinder + Area of base of cylinder
=47.1+226.08+28.26
=301.44cm2
Topper's Solved these Questions
- SURFACE AREAS AND VOLUMESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - SHORT ANSWER TYOE QUESTIONS
14 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ASWERS QUESTIONS
14 Videos
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - Long Answer Type Questions
18 Videos
Similar Questions
A rocket is in the form of a circular cylinder closed at the lower end with a cone of the same radius attached to the top. The cylinder is of radius 2.5 m and height 21 m and the cone has the slant height 8 m. Calculate the total surface area and the volume of the rocket.
View SolutionThe height of a right circular cone is 7 cm and radius is 24 cm. Find the slant height of the cone.
View SolutionA solid is in the form of a right circular cylinder, with a hemisphere at one end and a cone at the other end. The radius of the common base is 3.5 cm and the heights of the cylindrical and conical portions are 10 cm and 6 cm, respectively. Find the total surface area of the solid. (Use π=22/7 )
View SolutionA solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm. and the height of the cylindrical and conical portions are 12 cm and 7 cm respectively. Find the volume of the solid toy. (Use π=22/7 )
View SolutionA cone of height 15 cm is melted and reacast in to cylinder of same base .Find the height of the cylinder.
View SolutionA toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The height and radius of the cylindrical par are 13cm and 5cm respectively. The radii of the hemispherical and conical parts re the same as that of the cylindrical part. Calculate the surface area of the toy if height of the conical part is 12 cm.
View SolutionA toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The height and radius of the cylindrical part are 13cm and 5cm respectively. The radii of the hemispherical and conical parts are the same as that of the cylindrical part. Calculate the surface area of the toy if height of the conical part is 12 cm.
View SolutionFind the volume of a right circular cylinder, if the radius (r) of its base and height (h) are 7cm and 15cm respectively.
View SolutionFind the volume of a right circular cylinder, if the radius (r) of its base and height (h) are 7cm and 15cm respectively.
View SolutionA right circular cylinder and a right circular cone have the same radius and the same volume. The ratio of the height of the cylinder to that of the cone is (a) 3:5 (b) 2:5 (c) 3:1 (d) 1:3
View Solution
