AB is a diameter of a circle and C is any point on the circle. Show that the area of ABC is maximum, when it is isosceles.
To show that the area of triangle ABC is maximum when it is isosceles, we can follow these steps:
Step 1: Draw the Diagram
- Draw a circle with diameter AB.
- Let A and B be the endpoints of the diameter, and C be any point on the circumference of the circle.
Step 2: Identify the Properties of Triangle ABC
- Since AB is the diameter, angle C (∠ACB) is a right angle (90 degrees) by the inscribed angle theorem.
Step 3: Set Up the Area Formula
- The area A of triangle ABC can be given by the formula:
A=12×AC×BC
- Let AC=x and BC=y.
Step 4: Use Pythagorean Theorem
- Since triangle ABC is a right triangle, we can apply the Pythagorean theorem:
AB2=AC2+BC2⟹(2r)2=x2+y2⟹4r2=x2+y2
- Rearranging gives:
y2=4r2−x2
Step 5: Substitute in Area Formula
- Substitute y2 into the area formula:
A=12×x×y=12×x×√4r2−x2
Step 6: Maximize the Area
- To find the maximum area, we can differentiate A with respect to x and set the derivative to zero:
A=12x√4r2−x2
- Let A2=s:
s=14x2(4r2−x2)
Step 7: Differentiate and Set to Zero
- Differentiate s with respect to x:
dsdx=14(8r2x−4x3)
- Set the derivative to zero:
8r2x−4x3=0
4x(2r2−x2)=0
- This gives x=0 or x2=2r2 (i.e., x=r√2).
Step 8: Find y
- Substitute x=r√2 back into the equation for y:
y2=4r2−(r√2)2=4r2−2r2=2r2⟹y=r√2
Step 9: Show Isosceles Condition
- Since x=y=r√2, triangle ABC is isosceles when AC=BC.
Step 10: Verify Maximum Area Condition
- To confirm it is a maximum, check the second derivative:
d2sdx2<0
- This indicates that the area is maximized at x=r√2.
Conclusion
- The area of triangle ABC is maximum when it is isosceles, confirming that AC=BC.
- Draw a circle with diameter AB.
- Let A and B be the endpoints of the diameter, and C be any point on the circumference of the circle.
- Since AB is the diameter, angle C (∠ACB) is a right angle (90 degrees) by the inscribed angle theorem.
- The area A of triangle ABC can be given by the formula:
A=12×AC×BC
- Let AC=x and BC=y.
- Since triangle ABC is a right triangle, we can apply the Pythagorean theorem:
AB2=AC2+BC2⟹(2r)2=x2+y2⟹4r2=x2+y2
- Rearranging gives:
y2=4r2−x2
- Substitute y2 into the area formula:
A=12×x×y=12×x×√4r2−x2
- To find the maximum area, we can differentiate A with respect to x and set the derivative to zero:
A=12x√4r2−x2
- Let A2=s:
s=14x2(4r2−x2)
- Differentiate s with respect to x:
dsdx=14(8r2x−4x3)
- Set the derivative to zero:
8r2x−4x3=0
4x(2r2−x2)=0
- This gives x=0 or x2=2r2 (i.e., x=r√2).
- Substitute x=r√2 back into the equation for y:
y2=4r2−(r√2)2=4r2−2r2=2r2⟹y=r√2
- Since x=y=r√2, triangle ABC is isosceles when AC=BC.
- To confirm it is a maximum, check the second derivative:
d2sdx2<0
- This indicates that the area is maximized at x=r√2.
- The area of triangle ABC is maximum when it is isosceles, confirming that AC=BC.
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - OBJECTIVE TYPES QUESTIONS
25 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers
5 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers
5 Videos
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions
22 Videos
Similar Questions
AB is a diameter of a circle and C is any point on the circumference of the circle. Then a) the area of ABC is maximum when it is isosceles b) the area of ABC is minimum when it is isosceles c) the perimeter of ABC is minimum when it is isosceles d) none of these
View SolutionAB is a diameter of a circle and C is any point on the circumference of the circle. Then (a) the area of ΔABC is maximum when it is isosceles (b) the area of ΔABC is minimum when it is isosceles (c) the perimeter of ΔABC is minimum when it is isosceles (d) none of these
View Solution
Knowledge Check
In the figure, A, B, C are points on the circle. What is the value of x?
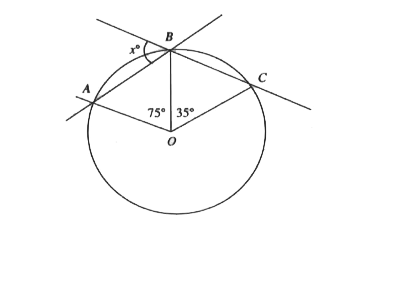 A45B55C60D65
A45B55C60D65
If AOB is a diameter of a circle and C is a point on the circle, then AC2+BC2=AB2.
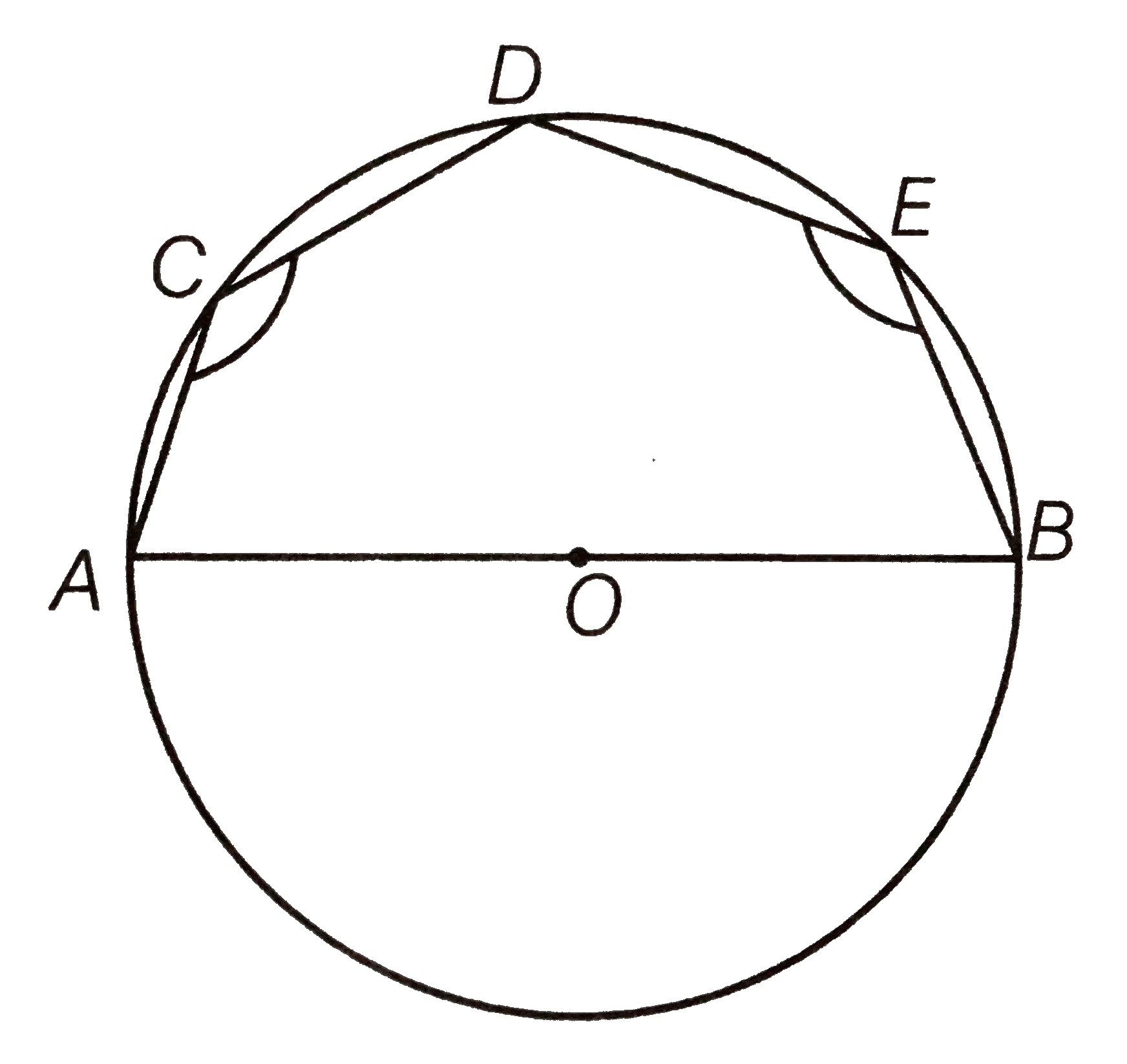
View SolutionIn figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD+∠BED.
View SolutionAn isosceles triangle of vertical angle 2θ is inscribed in a circle of radius a . Show that the area of the triangle is maximum when θ=π6 .
View SolutionThe sum of the perimeters of a square and a circle is given. Show that the sum of their areas is minimum when the length of a side of the square is equal to the diameter of the circle.
View Solution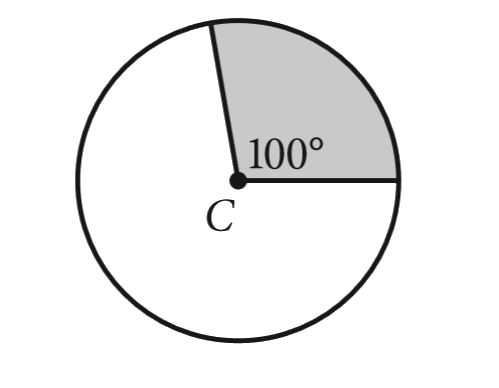
Point C is the center of the circle above. What fraction of the area of the circle is the area of the shaded region?
View SolutionIf ABC is an isosceles triangle and D is a point on BC such that AD⊥BC then,
View SolutionIn Figure, P, is any point on the chord BC of a circle such that AB=AP. Prove that CP=CQ.
View Solution
