To ensure almost 100% transmittivity, photographic lenses are often coated with a thin layer of dielectric material, like MgF2(μ=1.38) . The minimum thickness of the film to be used so that at the centre of visible spectrum (λ=5500Å) there is maximum transmission.
To find the minimum thickness of the dielectric film (MgF₂) that ensures maximum transmission of light at the center of the visible spectrum (λ = 5500 Å), we can follow these steps:
Step 1: Understand the Condition for Maximum Transmission
For maximum transmission through a thin film, we need to ensure that the path difference between the two rays reflected from the top and bottom surfaces of the film leads to destructive interference. This occurs when the optical path difference is equal to λ/2.
Step 2: Calculate the Optical Path Difference
The optical path difference (OPD) for the two rays can be expressed as:
OPD=2ndcosr
where:
- n is the refractive index of the film (for MgF₂, n=1.38),
- d is the thickness of the film,
- r is the angle of refraction.
Step 3: Set the Condition for Destructive Interference
For destructive interference, we set the optical path difference equal to λ/2:
2ndcosr=λ2
Step 4: Simplify the Equation
Rearranging the equation gives:
ndcosr=λ4
Thus, the thickness d can be expressed as:
d=λ4ncosr
Step 5: Consider the Angle of Incidence and Refraction
For normal incidence (when light strikes the surface perpendicularly), the angle of incidence I and angle of refraction R are both zero. Therefore, cosr=1.
Step 6: Substitute Values
Substituting the values into the equation:
d=5500Å4×1.38×1
Calculating this gives:
d=55005.52≈997.1Å
Step 7: Round to Significant Figures
Rounding to significant figures, we find:
d≈1000Å
Final Answer
The minimum thickness of the film required for maximum transmission is approximately 1000 Å.
---
For maximum transmission through a thin film, we need to ensure that the path difference between the two rays reflected from the top and bottom surfaces of the film leads to destructive interference. This occurs when the optical path difference is equal to λ/2.
The optical path difference (OPD) for the two rays can be expressed as:
OPD=2ndcosr
where:
- n is the refractive index of the film (for MgF₂, n=1.38),
- d is the thickness of the film,
- r is the angle of refraction.
For destructive interference, we set the optical path difference equal to λ/2:
2ndcosr=λ2
Rearranging the equation gives:
ndcosr=λ4
Thus, the thickness d can be expressed as:
d=λ4ncosr
For normal incidence (when light strikes the surface perpendicularly), the angle of incidence I and angle of refraction R are both zero. Therefore, cosr=1.
Substituting the values into the equation:
d=5500Å4×1.38×1
Calculating this gives:
d=55005.52≈997.1Å
Rounding to significant figures, we find:
d≈1000Å
The minimum thickness of the film required for maximum transmission is approximately 1000 Å.
Topper's Solved these Questions
- WAVE OPTICSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Short answer type Questions
3 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIAL,DEVICES AND SIMPLE CIRCUITEXERCISE - Multiplel choice questions
26 Videos
Similar Questions
On face of a glass (μ=1.50) lens is coated with a thin film of magnesium fluoride MgF2(μ=1.38) to reduce reflection from the lens surface. Assuming the incident light to be perpendicular to the lens surface. The least coating thickness that eliminates the reflection at the centre of the visible spectrum (λ=550nm) is about
View SolutionA glass lens is coated on one side with a thin film of magnesium fluoride (MgF2)to reduce reflection from the lens surface (Fig. 2.26). The Index of refraction of MgF2 is 1.38, that of the glass is 1.50. What is the least coating thickness that eliminates (via interference) the reflections at the middle of the visible specturm (λ=550nm)? Assume that the light is approxmately perpendicular to the lens surface.
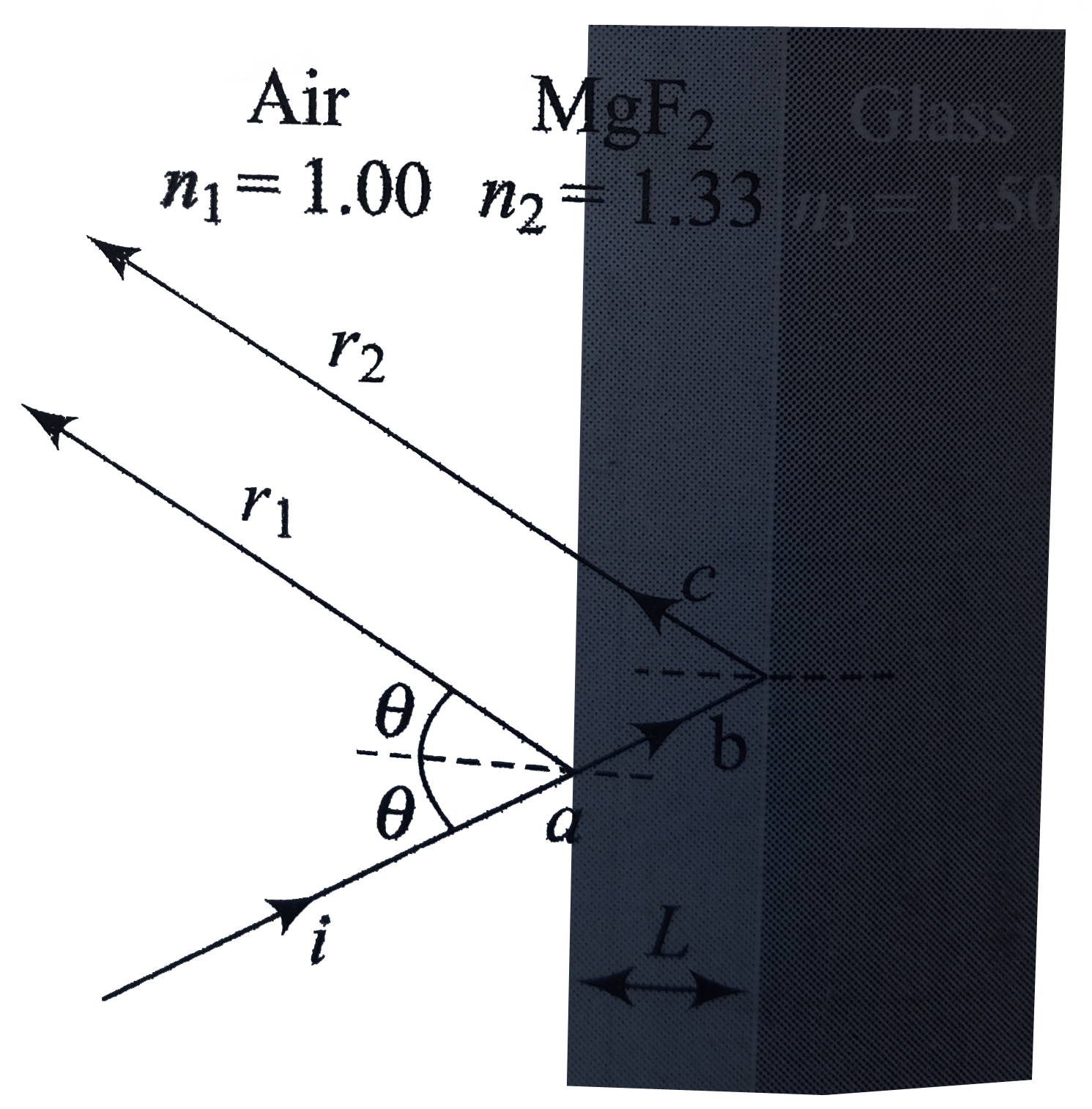
View SolutionIn the YDSE, the monochromatic source of wavelength λ is placed at a distance d2 from the central axis (as shown in the figure), where d is the separation between the two slits S1andS2 .
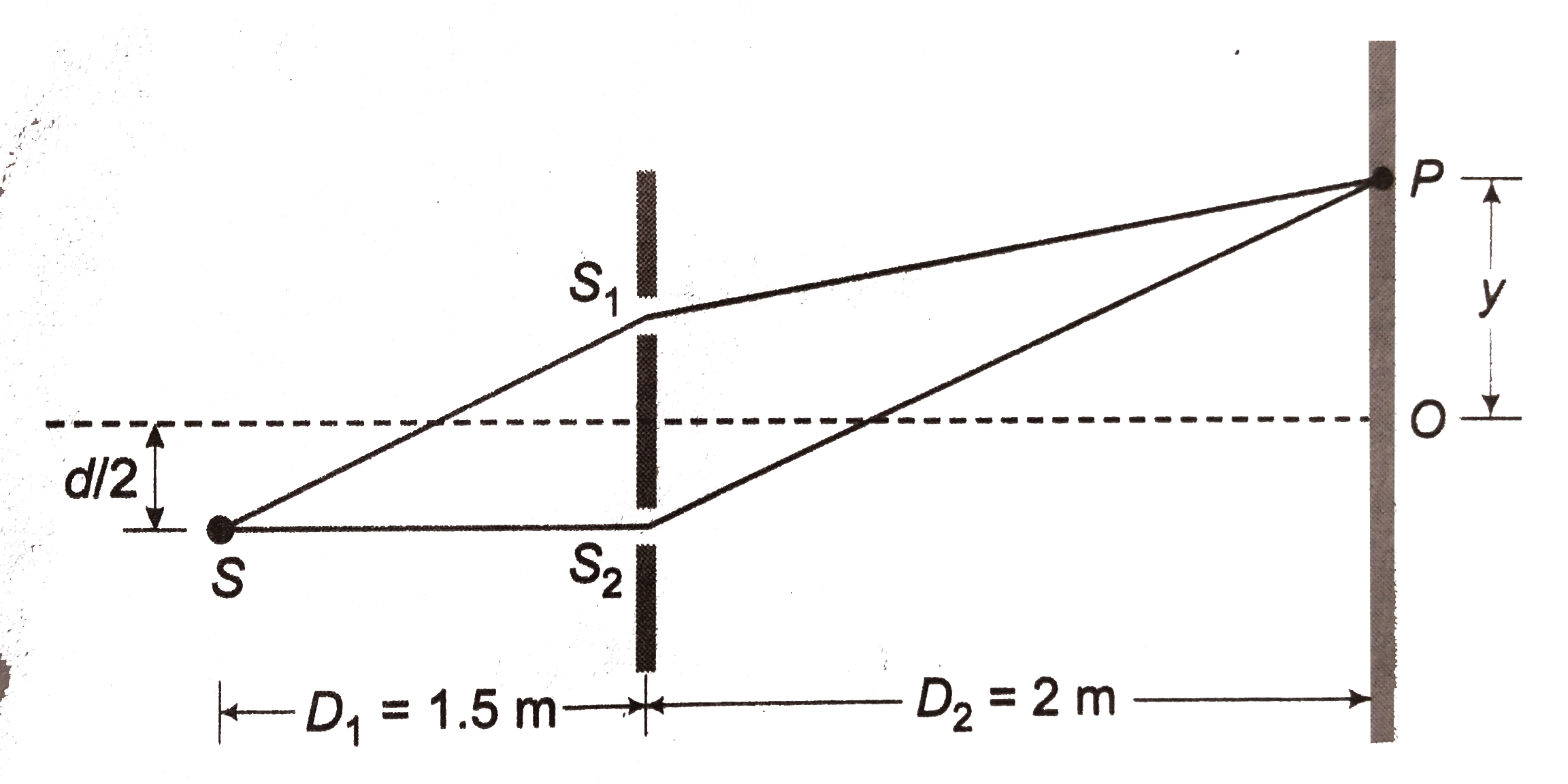
(a)Find the position of the central maxima.
(b) Find the order of interference formed at O.
(c)Now, S is placed on centre dotted line. Find the minimum thickness of the film of refractive indes μ=1.5 to be placed in front of S2 so that intensity at O becomes 34th of the maximum intensity.
(Take λ=6000Å,d=6mm.)
View SolutionIn solar cells, a silicon solar cell (μ=3.5) is coated with a thin film
of silicon monoxide SiO(μ=1.45) to minimize reflective losses from the surface.
Determine the minimum thickness of SiO that produces the least reflection at a
wavelength of 550nm, near the centre of the visible spectrum. Assume
approximately normal incidence .
View SolutionIn solar cells, a silicon solar cell (μ=3.5) is coated with a thin film
of silicon monoxide SiO(μ=1.45) to minimize reflective losses from the surface.
Determine the minimum thickness of SiO that produces the least reflection at a
wavelength of 550nm, near the centre of the visible spectrum. Assume
approximately normal incidence .
View SolutionA thin film of a specific meterial can be used to decrease the intensity of reflected light. There is destrucive inteference of wave reflected from upper and lower surface of the film. These films are called non-reflecting or anti-reflecting coatings. The process of coating the lens or surface with non-reflecting film is called blooming as shown in figure The refracting index of coating (n1) is less than that of the glass (n2).

5. magnesium fluoride (MgF2) is generally use as anti-reflection coating. If refractive index of MgF2 is 1.25, then minimum thickness of film required is (Take λ=500nm)
View SolutionTo produce a minimum reflection of wavelength near the middle of visible spectrum (550 nm), how thick should a coating of MgF2(μ=1.38) be vaccum-coated on a glass surface?
View SolutionA thick glass slab (μ=1.5) is to be viewed in reflected white light. It is proposed to coat the slab with a thin layer of a material having refractive index 1.3 so that the wavelength 6000 Å os suppressed. Find the minimum thickness of the coating required.
View SolutionIn YDSE, both slits produce equal intensities on the screen. A 100% transparent thin film is placed in front of one of the slits. Now, the intensity on the centre becomes 75% of the previous intensity. The wavelength of light is 6000Å and refractive index of glass is 1.5. Thus, minimum thickness of the glass slab is
View Solution
