If the coordinates of the middle point of the portion of a line interceptecd between the coordinate axes is (3,2), then the equation of the line will be
2x+3y=12
3x+2y=12
4x−3y=6
5x−2y=10
The correct Answer is:A
Since, the coordinate of the middle point are P (3,2)
∴3=1.0+1.a1+1
⇒3=a2⇒a=6
Similarly, b=4
∴ Equation of the line is x6+y4=1
⇒2x+3y=12
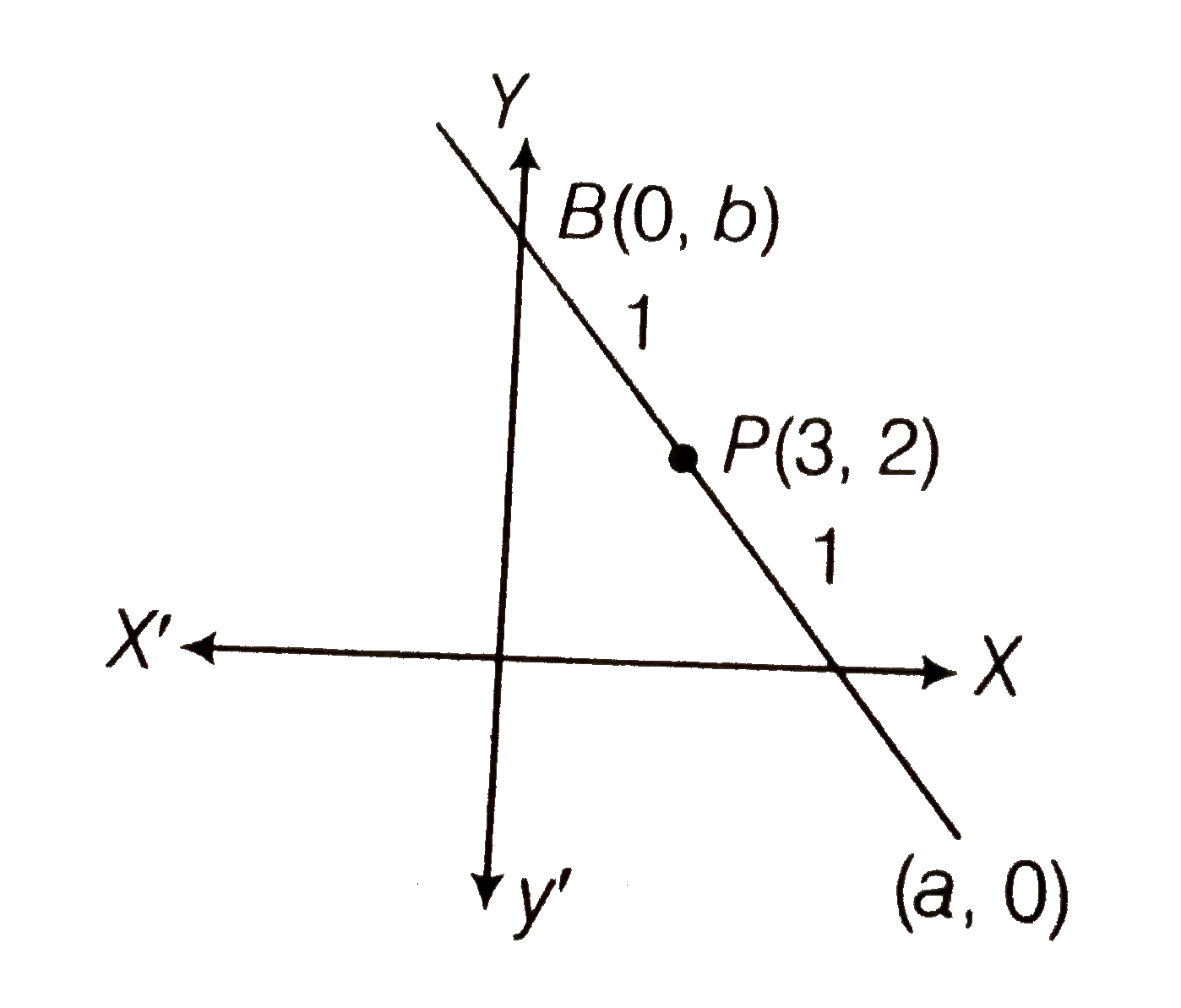
Topper's Solved these Questions
- STRAIGHT LINESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Fillers
6 Videos
- STRAIGHT LINESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - TRUE/FALSE
9 Videos
- STRAIGHT LINESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Long Answer type
9 Videos
- STATISTICSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICSEXERCISE - FILLERS
7 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - TRUE/FALSE
9 Videos
Similar Questions
A line passes through (−3,4) and the portion of the line intercepted between the coordinate axes is bisected at the point then equation of line is (A) 4x−3y+24=0 (B) x−y−7=0 (C) 3x−4y+25=0 (D) 3x−4y+24=0
View SolutionA straight line passes through the point (3, 2) and the portion of this line, intercepted between the positive axes, is bisected at this point. Find the equation of the line.
View SolutionA straight line passes through the point (α,β) and this point bisects the portion of the line intercepted between the axes. Show that the equation of the straight line is x2α+y2β=1.
View SolutionFor the curve xy=c, prove that the portion of the tangent intercepted between the coordinate axes is bisected at the point of contact.
View SolutionFor the curve xy=c, prove that the portion of the tangent intercepted between the coordinate axes is bisected at the point of contact.
View SolutionFind the equation of the bisectors of the angles between the coordinate axes.
View SolutionA straight line passes through the point (3,−2) and this point bisects theportion of the line intercepted between the axes, find the equation of the line
View SolutionThe equation straight line such that the portion of it intercepted between the coordinate axes is bisected at the point (h,k) is :
View SolutionThe equation of the curve in which the portion of the tangent included between the coordinate axes is bisected at the point of contact, is
View SolutionA line passes through the point (3,-2). Find the locus of the middle point of the portion the line intercepted between the axes.
View Solution
