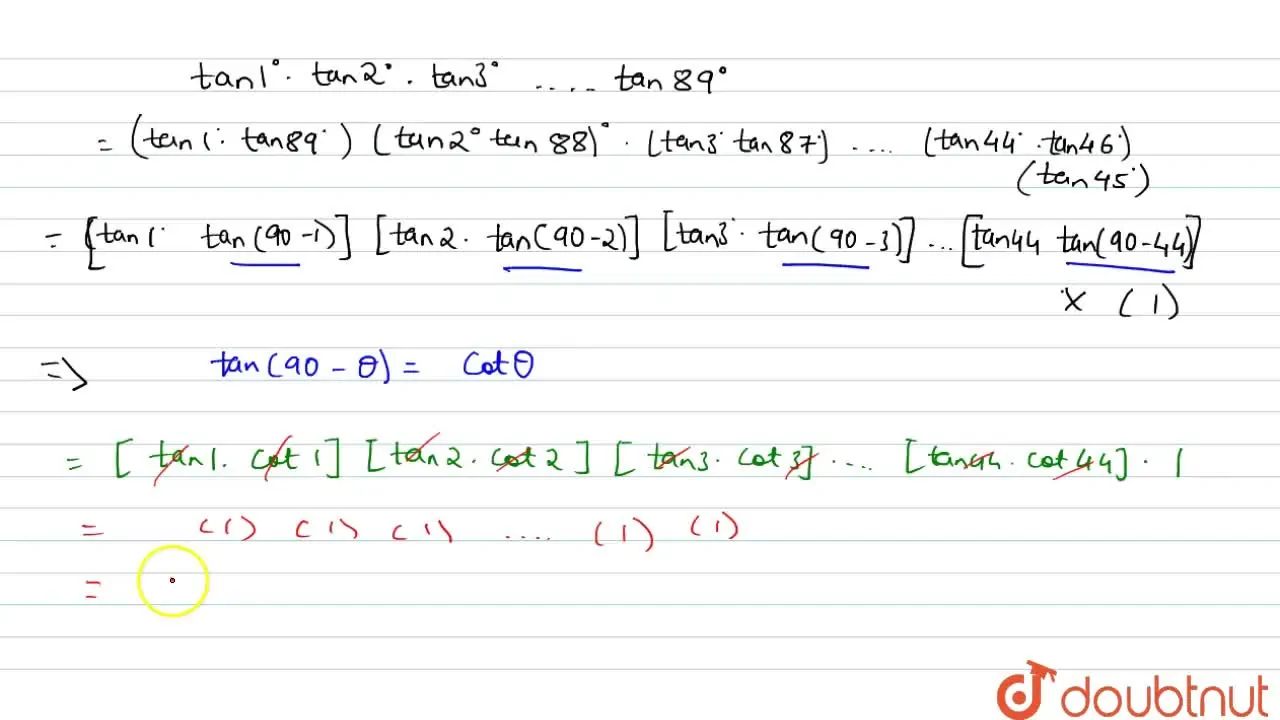
The value of tan1∘tan2∘tan3∘...tan89∘ is
A
0
B
1
C
2
D
12
Video Solution
Text Solution
The correct Answer is:B
tan1∘.tan2∘.tan3∘…….tan89∘
=tan1∘.tan2∘.tan3∘….tan44∘.tan45∘.tan46∘….tan87∘.tan88∘.tan89∘
=tan1∘.tan2∘.tan3∘…..tan44∘.(1).Cot44∘……cot3∘.cot2∘.cot1∘
[∴tan(90∘−θ)=cotθ]
=tan1∘.tan2∘.tan3∘.....tan44∘×1tan44∘...1tan30∘.1tan2∘.1tan1∘ [∴cotθ=1tanθ]
=1
|
Updated on:21/07/2023
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS11videos
INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSEXERCISE - SHORT ANSWER TYPE QUESTIONS15videos
INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 7.4 Long Answer Type Questions6videos
COORDINATE GEOMETRY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 3.4 Long Answer Type Questions13videos
PAIR OF LINEAR EQUATIONS IN TWO VARIABLES
Similar Questions
Write the value of tan10∘tan15∘tan75∘tan80∘ .
View SolutionThe value of tan9∘−tan27∘−tan63∘+tan81∘ is equal to
View SolutionThe value of tan10∘tan50∘tan70∘ is
View SolutionThe value of: tan3A−tan2A−tanA =
View SolutionThe value of tan1∘.tan2∘.tan3∘.......tan89∘ is
View SolutionThe value of tan9∘+tan36∘+tan9∘.tan36∘ is equal to
View SolutionThe value of tan−1tan(−6)is
View SolutionThe value of tan20∘+2tan50∘−tan70∘, is
View SolutionIf tan1∘tan2∘tan3∘tan4∘...tan89∘=x2−8, then find the value of x.
View SolutionThe value of 1−tan215∘1+tan215∘ is
View Solution