In figure, ∠ADC=130∘ and chord BC=chord BE. Find ∠CBE.
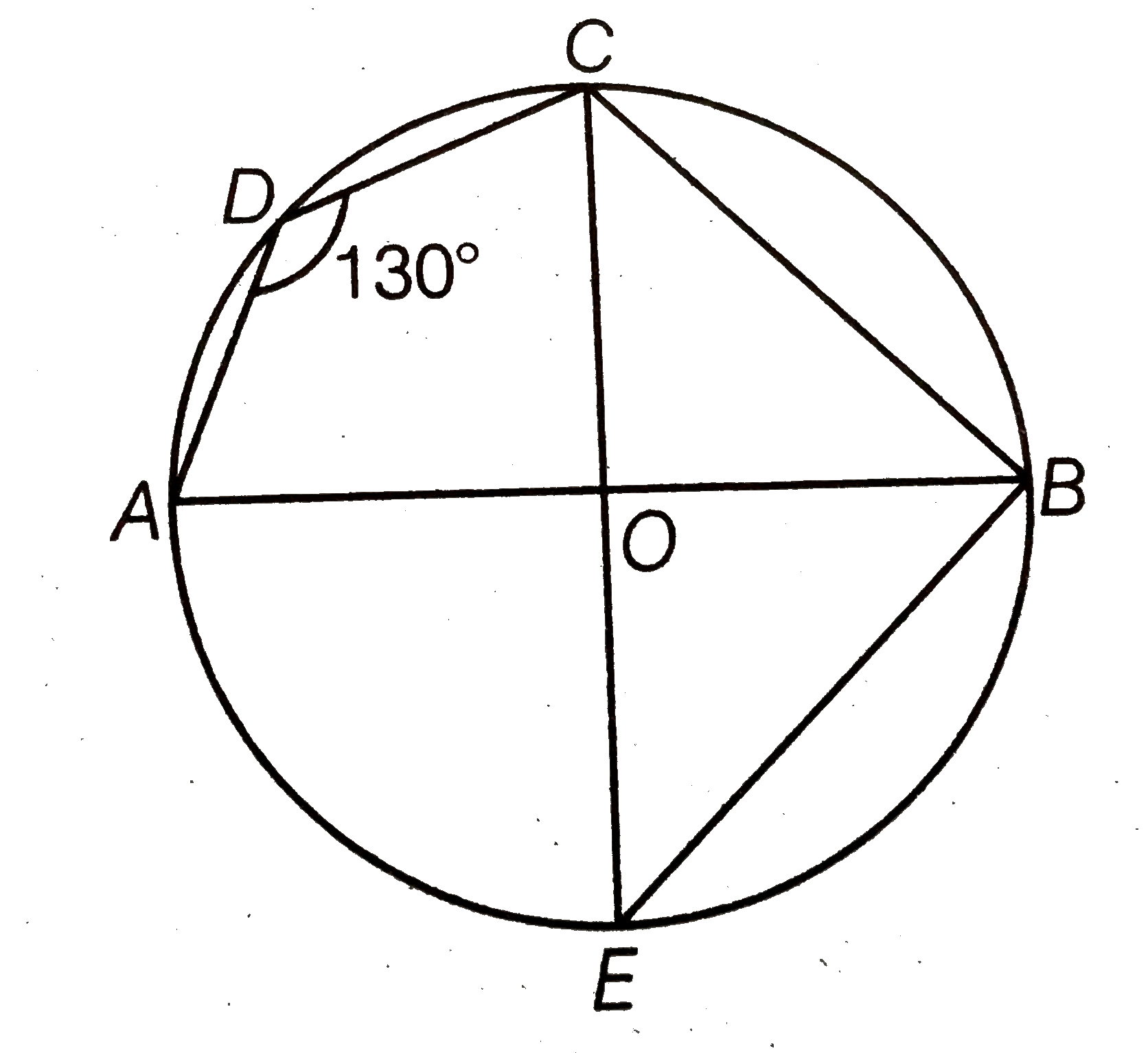
We have, ∠ADC=130∘ and chord BC=chord BE. Suppose, we consider the points A, B, C and D form a cyclic quadrilateral.
Since, the sum of opposite angles of a cyclic quadrilateral ADCB is 180∘.
∴∠ADC+∠OBC=180∘
⇒130∘+∠OBC=180∘
⇒∠OBC=180∘−130∘=50∘
InΔBOCandΔBOE,
BC=BE [given equal chord]
OC=OE [both are the radius of a circle]
and OB=OB [common side]
∴ΔBOC≅ΔBOE [by SSS songruence rule]
⇒∠OBC=∠OBE=50∘ [by CPCT]
Now, ∠CBE=∠CBO+∠EBO
=50∘+50∘=100∘
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.4
14 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.2
10 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.4 Long Answer Type Questions
10 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
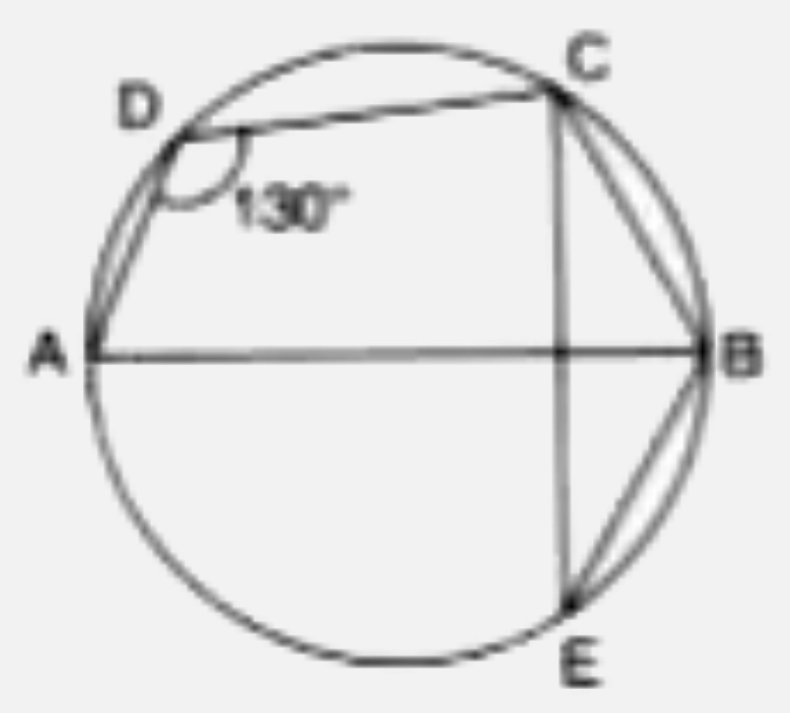
In the given figure, ∠ADC=130∘ and BC= BE. Find ∠CBE if AB⊥CE
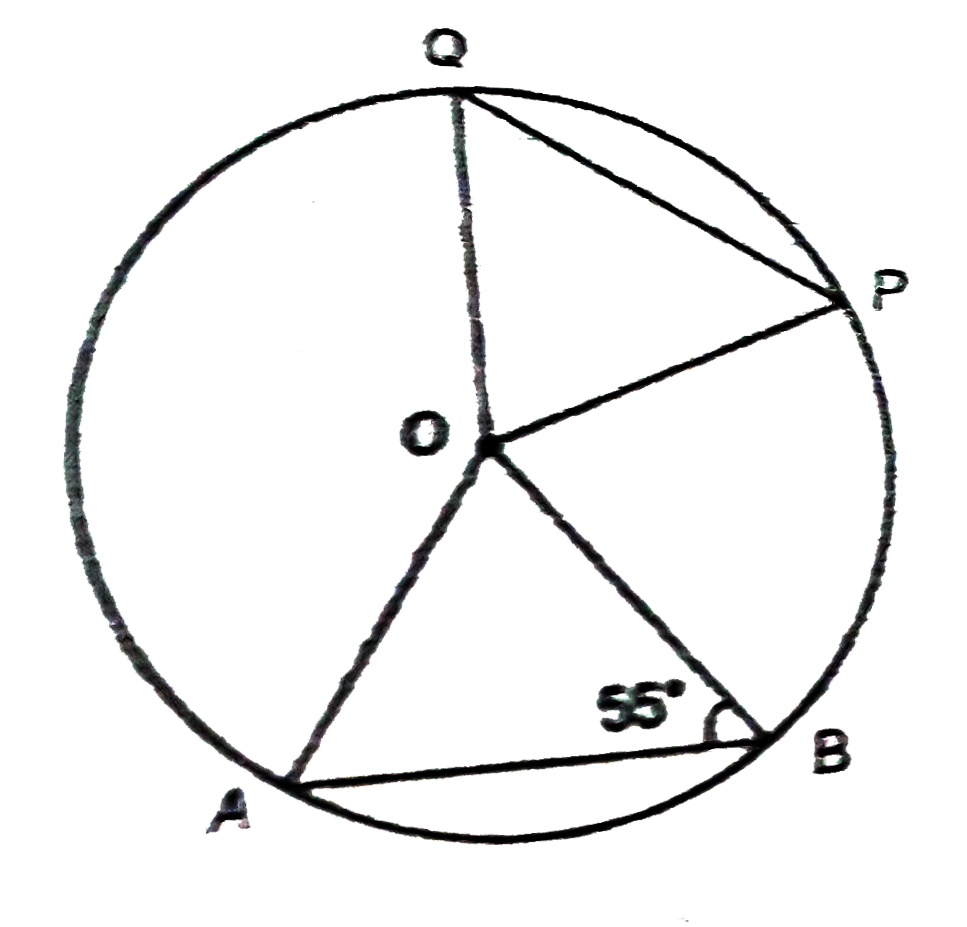
View SolutionIn the adjoining figure, chord AB= chord PQ. If ∠OBA=55∘, then find ∠POQ.
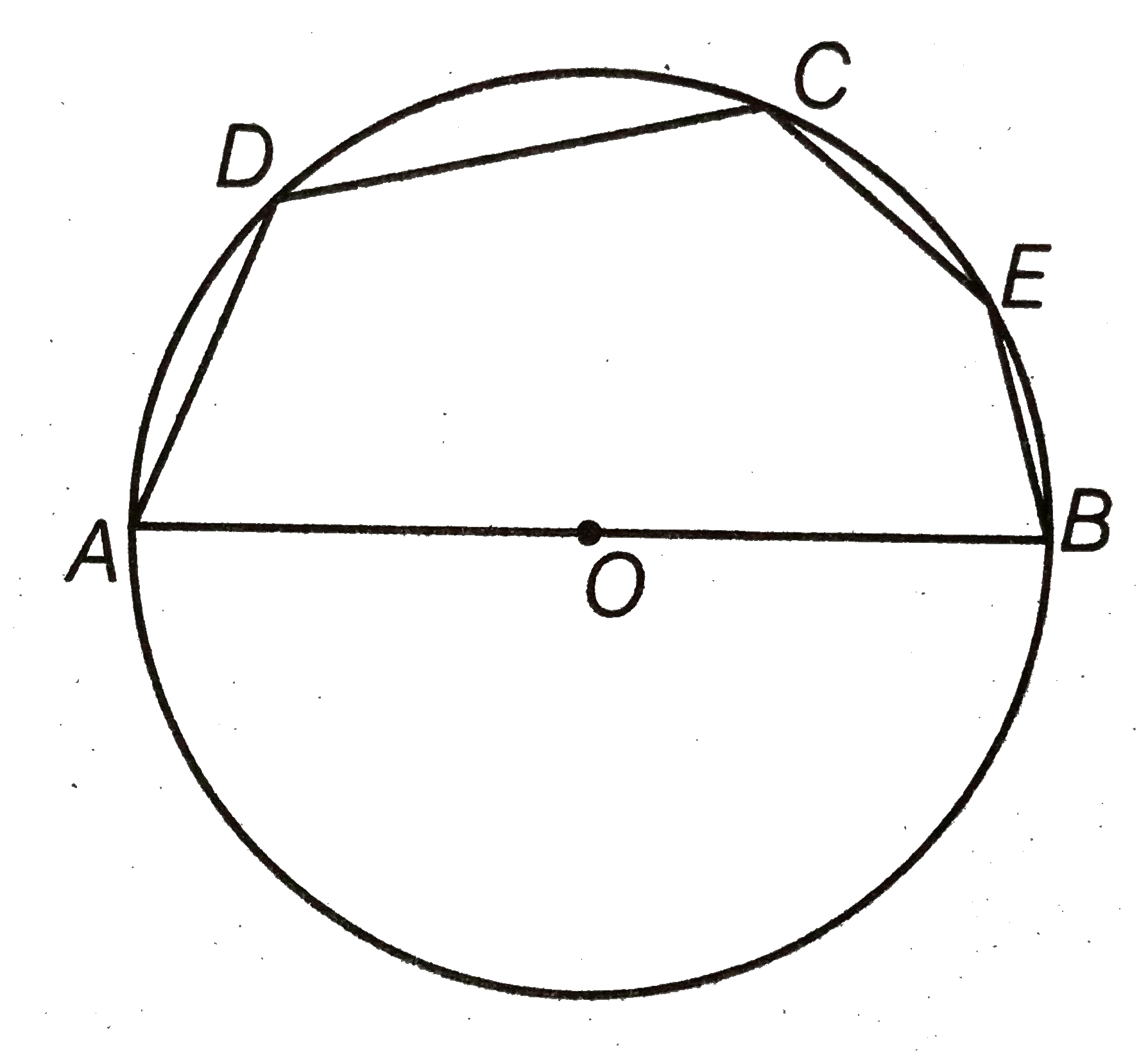
View SolutionIn figure, if AOB is a diameter and ∠ADC=120∘,then∠CAB=30∘.
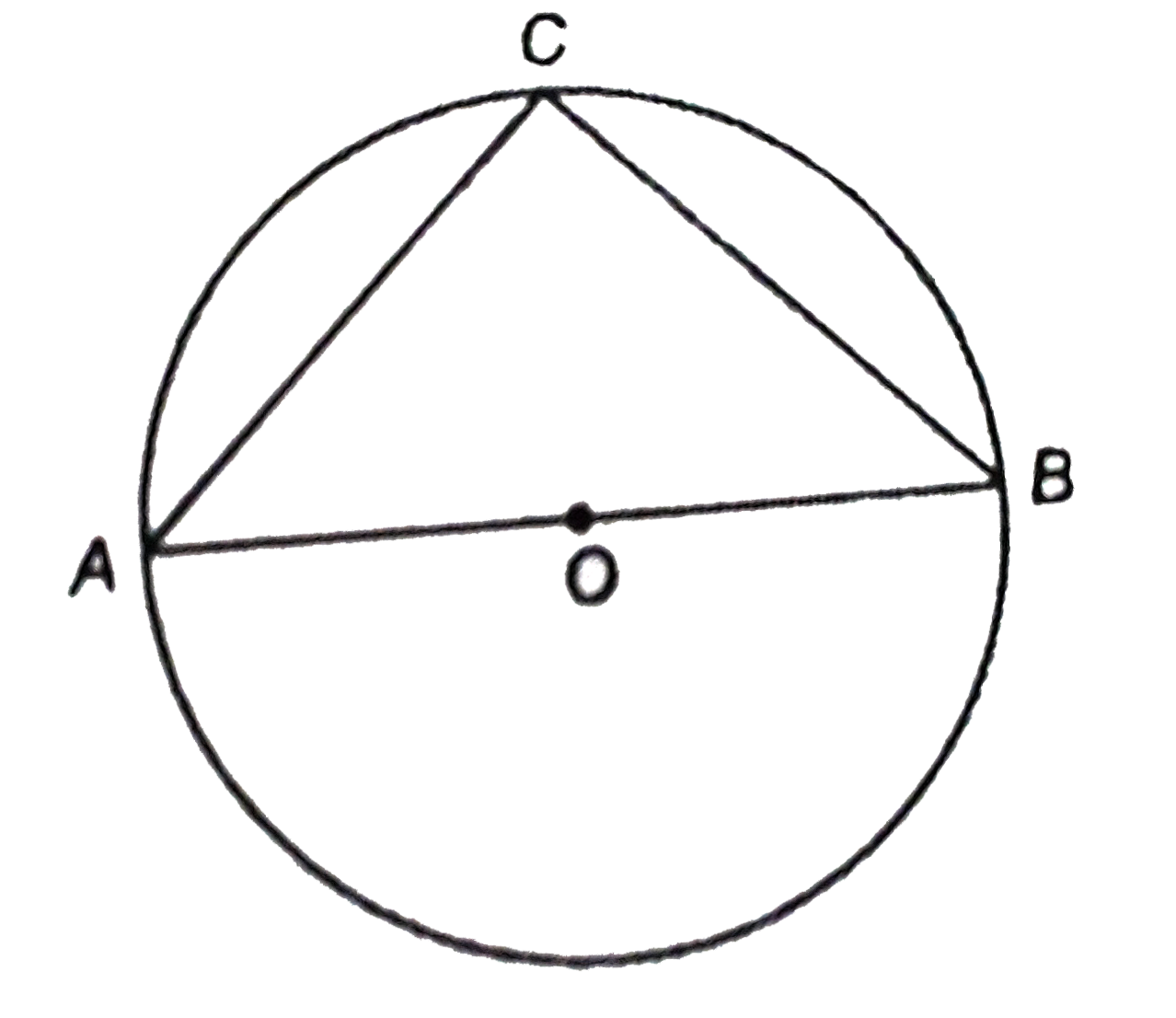
View SolutionO is the centre of a circle of diameter AB. If chord AC= chord BC, then find the value of ∠ABC.
View SolutionIn the given figure , find the length of chord AB .
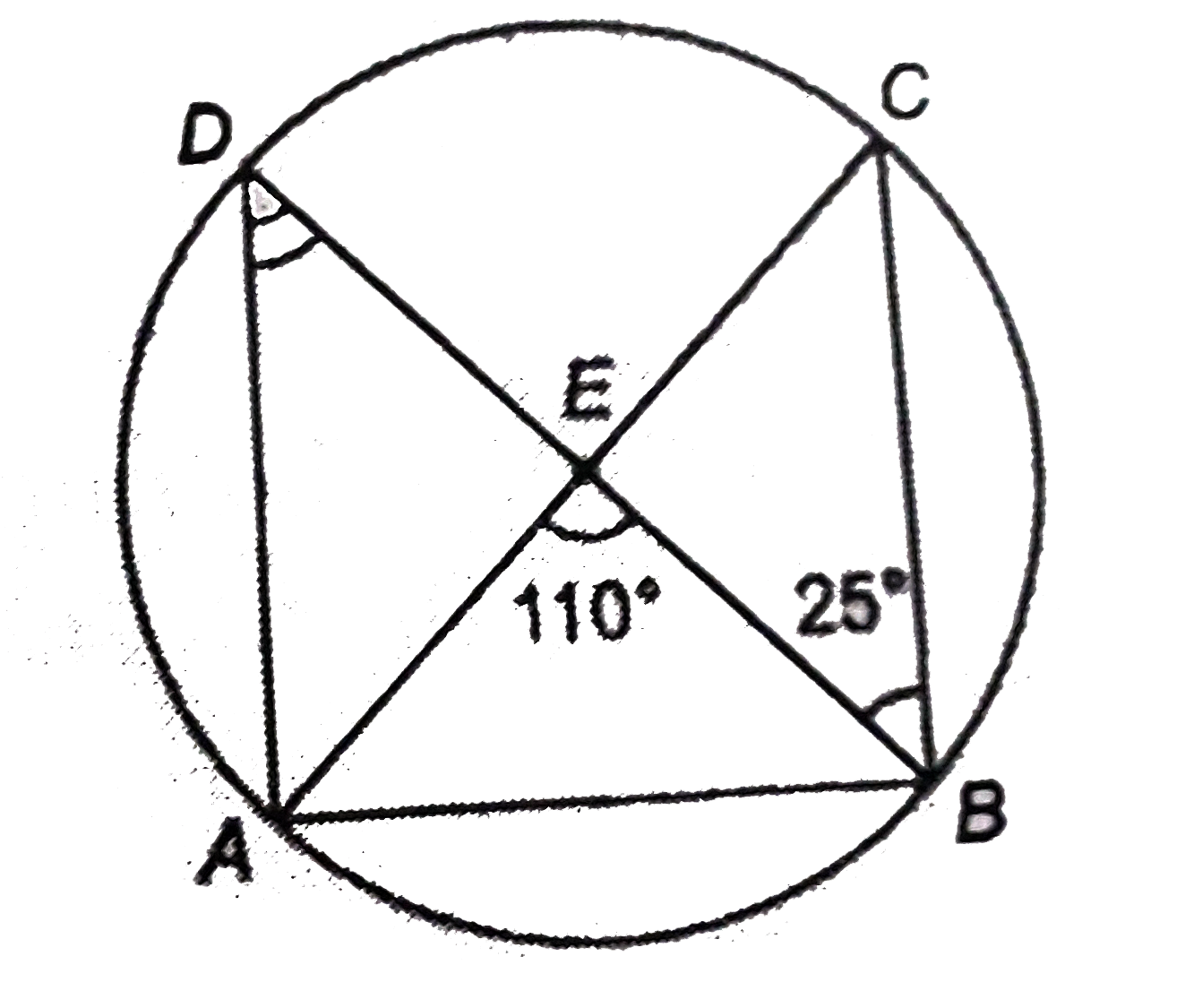
View SolutionIn the adjoining figure, AB is a chord of the circle. If ∠AEB=110∘ and ∠EBC=25∘,then find the value of ∠ADB.
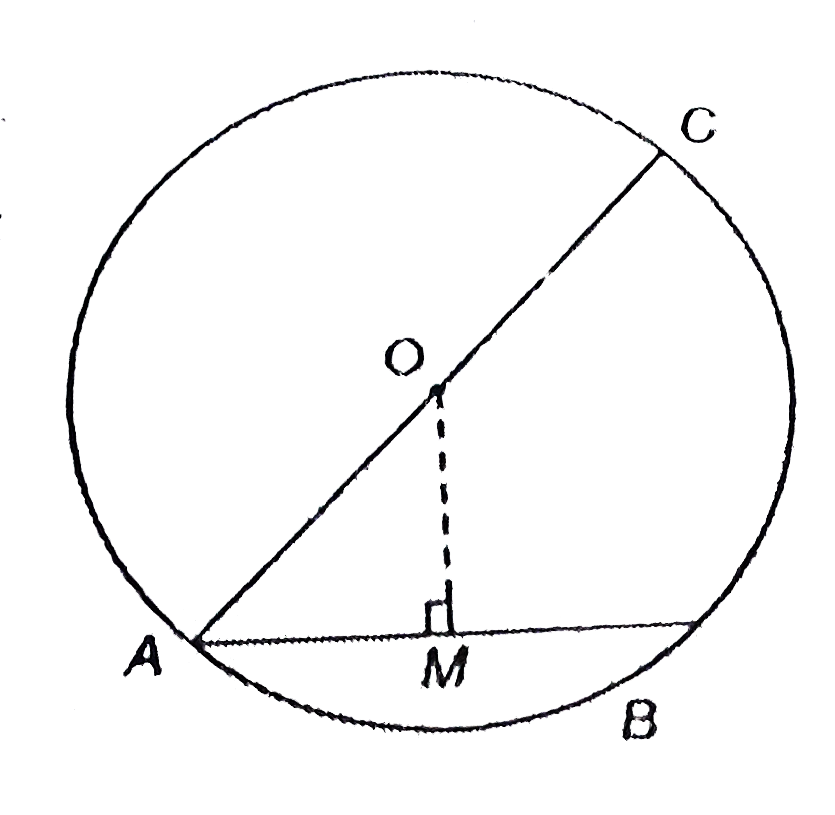
View SolutionIn the adjoining figure, ∠ADB and ∠ACB are the angles in the same segment and chord AC passes through the centre O. If ∠CAB=40∘ then find the value of ∠ADB.

View SolutionIn the adjoining figure, O is the centre of the circle.If ∠BAC=40∘, then find the value of ∠ADC.

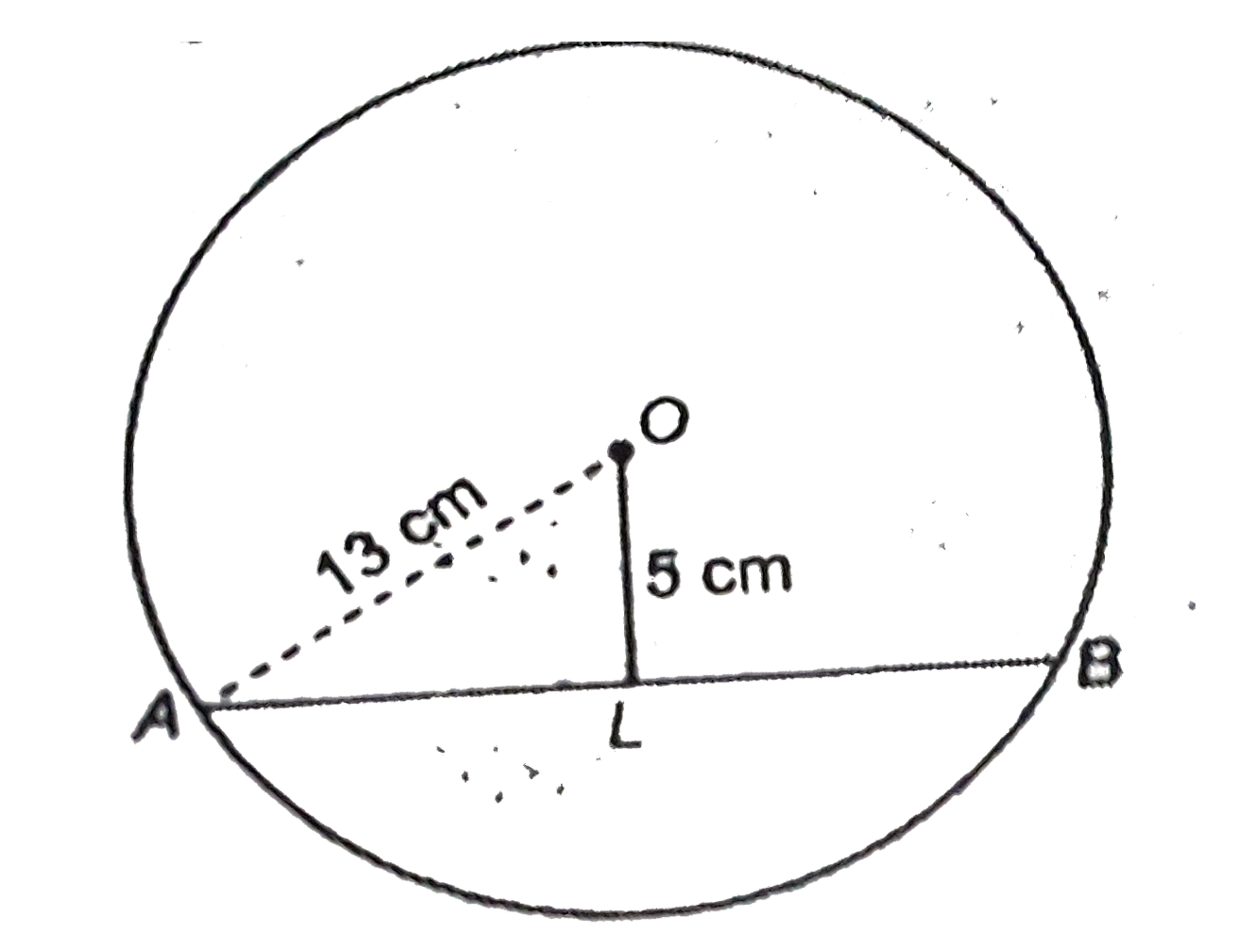
View SolutionIn the adjoining figure, O is the centre of the centre of the circle. If diameter AC=26cm and chord AB=10cm, then find the distances of the chord AB from the centre of the circle.
View SolutionIn figure, tangents PQ and PR are drawn to a circle such that ∠RPQ=30∘. A chord RS is drawn parallel to the tangent PQ. Find the ∠RQS.
View Solution
