If a triangle and a parallelogram are on the same base and between same parallels, then the ratio of the area of the triangle to the area of parallelogram is
1:3
1:2
3:1
1:4
The correct Answer is:B
To solve the problem, we need to establish the relationship between the areas of a triangle and a parallelogram that share the same base and are situated between the same parallels.
1. Understand the Configuration:
- We have a triangle and a parallelogram.
- Both shapes share the same base and are located between the same set of parallel lines.
2. Area of Parallelogram:
- The area of a parallelogram is calculated using the formula:
Area of Parallelogram=Base×Height
3. Area of Triangle:
- The area of a triangle is calculated using the formula:
Area of Triangle=12×Base×Height
4. Establishing the Relationship:
- Since both shapes share the same base and height (the height is the perpendicular distance between the two parallel lines), we can express the area of the triangle in terms of the area of the parallelogram:
Area of Triangle=12×Area of Parallelogram
5. Finding the Ratio:
- To find the ratio of the area of the triangle to the area of the parallelogram, we set up the following equation:
Area of TriangleArea of Parallelogram=12×Area of ParallelogramArea of Parallelogram
- Simplifying this gives:
Area of TriangleArea of Parallelogram=12
6. Expressing the Ratio:
- The ratio of the area of the triangle to the area of the parallelogram can be expressed as:
1:2
Conclusion:
The ratio of the area of the triangle to the area of the parallelogram is 1:2.
- We have a triangle and a parallelogram.
- Both shapes share the same base and are located between the same set of parallel lines.
- The area of a parallelogram is calculated using the formula:
Area of Parallelogram=Base×Height
- The area of a triangle is calculated using the formula:
Area of Triangle=12×Base×Height
- Since both shapes share the same base and height (the height is the perpendicular distance between the two parallel lines), we can express the area of the triangle in terms of the area of the parallelogram:
Area of Triangle=12×Area of Parallelogram
- To find the ratio of the area of the triangle to the area of the parallelogram, we set up the following equation:
Area of TriangleArea of Parallelogram=12×Area of ParallelogramArea of Parallelogram
- Simplifying this gives:
Area of TriangleArea of Parallelogram=12
- The ratio of the area of the triangle to the area of the parallelogram can be expressed as:
1:2
The ratio of the area of the triangle to the area of the parallelogram is 1:2.
Topper's Solved these Questions
- Areas of Parallelograms and TrianglesBook:NCERT EXEMPLAR ENGLISHChapter:Areas of Parallelograms and TrianglesExercise:Exercise-9.2 Very Short Answer Type Questions
Explore 5 Videos
- Areas of Parallelograms and TrianglesBook:NCERT EXEMPLAR ENGLISHChapter:Areas of Parallelograms and TrianglesExercise:Exercise-9.3 Short Answer Type Questions
Explore 9 Videos
- CIRCLESBook:NCERT EXEMPLAR ENGLISHChapter:CIRCLESExercise:Exercise 10.4
Explore 14 Videos
Similar Questions
If a triangle and a parallelogram are on the same base and between the same parallels, then prove that the area of the triangle is equal to half the area of the parallelogram.
View SolutionA triangle and a parallelogram are on the same base and between the same parallels. The ratio of the areas of triangle and parallelogram is
(a)1:1
(b) 1:2
(c) 2:1
(d) 1:3
View SolutionIf a triangle and a parallelogram are on the same base and between the same parallels lines, then the area of the triangle is equal to half that of the parallelogram. GIVEN : A △ABCand∣∣gmBCDE on the same base BC and between the same parallels BCandAD. TO PROVE : ar(△ABC)=1/2 ar(∣∣gmBCDE) CONSTRUCTION : Draw AL ⊥ BC and DM⊥BC, produced at M.
View SolutionTwo parallelograms are on equal bases and between the same parallels.
The ratio of their areas is
View SolutionTwo parallelograms are on equal bases and between the same parallels.
The ratio of their areas is
View SolutionIn the figure, the area of parallelogram ABCD is
View SolutionProve that Parallelograms on the same base and between the same parallels are equal in area.
View SolutionWhat is the area of the parallelogram?
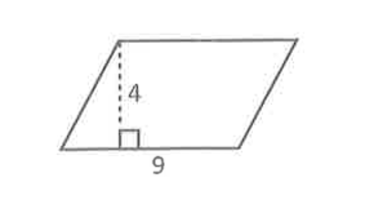
View SolutionABCD is a parallelogram. Find the ratio of the area of Δ ABC to the area of the parallelogram ABCD.
View SolutionTriangles on the same base and between the same parallels are equal in area.
View Solution