The mid-point of the sides of triangle along with any of the vertices as the fourth point make a parallelogram of area equal to
12ar(ABC)
13ar(ABC)
14ar(ABC)
ar(ABC)
The correct Answer is:A
To solve the problem, we need to find the area of the parallelogram formed by the midpoints of the sides of triangle ABC and one of its vertices. Let's go through the solution step by step.
1. Identify the Triangle and Midpoints:
Let triangle ABC have vertices A, B, and C. We denote the midpoints of sides AB, BC, and AC as D, E, and F respectively.
2. Understanding the Parallelogram:
We will consider the parallelogram formed by one vertex (say A) and the midpoints D, E, and F. For our case, we will focus on the parallelogram ADEF.
3. Area of Triangle ABC:
The area of triangle ABC can be denoted as Area(ABC).
4. Area of Triangles Formed by Midpoints:
Since D, E, and F are midpoints, the triangles ADF, DEF, and EBC are formed. The area of triangle DEF will be equal to one-fourth of the area of triangle ABC. This is because the midpoints divide the triangle into smaller triangles of equal area.
Area(DEF)=14Area(ABC)
5. Area of Triangle ADF:
The area of triangle ADF is equal to the area of triangle DEF because they share the same base DF and height from A to line DF.
Area(ADF)=Area(DEF)=14Area(ABC)
6. Area of Parallelogram ADEF:
The area of the parallelogram ADEF is equal to twice the area of triangle ADF (since a parallelogram can be split into two triangles).
Area(ADEF)=2×Area(ADF)=2×14Area(ABC)=12Area(ABC)
7. Conclusion:
Therefore, the area of the parallelogram ADEF is equal to half the area of triangle ABC.
Final Answer:
The area of the parallelogram formed by the midpoints of the sides of triangle ABC and one of its vertices is 12Area(ABC).
---
Let triangle ABC have vertices A, B, and C. We denote the midpoints of sides AB, BC, and AC as D, E, and F respectively.
We will consider the parallelogram formed by one vertex (say A) and the midpoints D, E, and F. For our case, we will focus on the parallelogram ADEF.
The area of triangle ABC can be denoted as Area(ABC).
Since D, E, and F are midpoints, the triangles ADF, DEF, and EBC are formed. The area of triangle DEF will be equal to one-fourth of the area of triangle ABC. This is because the midpoints divide the triangle into smaller triangles of equal area.
The area of triangle ADF is equal to the area of triangle DEF because they share the same base DF and height from A to line DF.
The area of the parallelogram ADEF is equal to twice the area of triangle ADF (since a parallelogram can be split into two triangles).
Therefore, the area of the parallelogram ADEF is equal to half the area of triangle ABC.
The area of the parallelogram formed by the midpoints of the sides of triangle ABC and one of its vertices is 12Area(ABC).
Topper's Solved these Questions
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.2 Very Short Answer Type Questions
5 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.3 Short Answer Type Questions
9 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.4
14 Videos
Similar Questions
The mid points of the sides of a triangle ABC along with any of the vertices as the fourth point make a parallelogram of area equal to: ar (ABC) (b) 12ar (ABC) 13ar (ABC) (d) 14 ar (ABC)
View SolutionIf the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram, so formed will be half of the area of the given quadrilateral (figure).
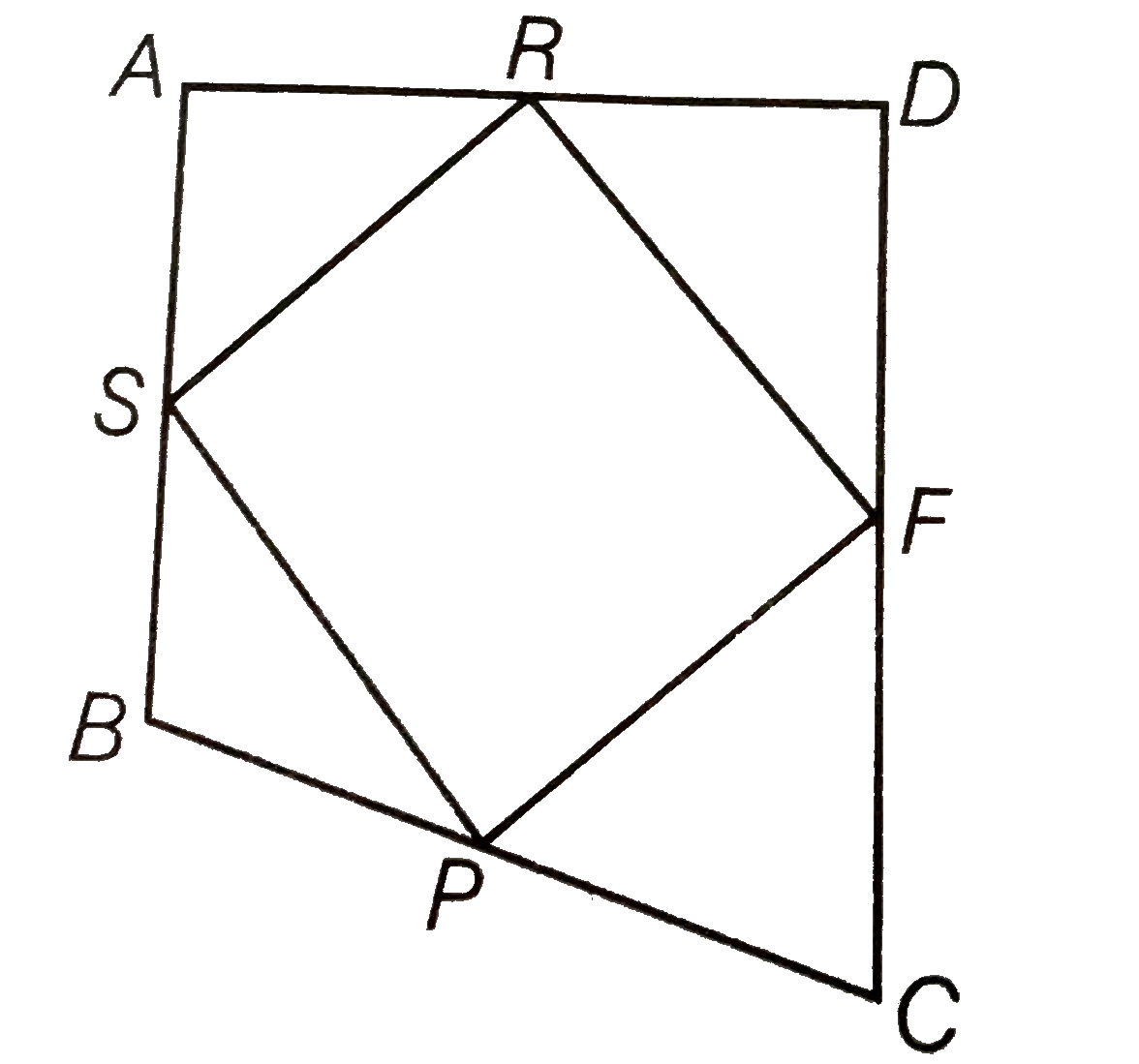
View SolutionE, F, G and H are the mid-points of the sides of a parallelogram ABCD. Show that area of quadrilateral EFGH is half of the area of parallelogram ABCD.
View SolutionFind the area of the triangle formed by joining the mid–points of the sides of the triangle whose vertices are (0, –1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle.
View SolutionIf the coordinates of the mid-points of the sides of a triangle are (1, 1), (2, −3) and (3, 4) , find the vertices of the triangle.
View SolutionThe line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, CA and AB respectively of ΔABC. Prove that:
(i) □BDEF is a parallelogram
(ii) area of ΔDEF=14× area of ΔABC
(iii) □BDEF=12× area of ΔABC

View SolutionThe mid-points of the sides of a triangle are (1,5,−1),(0,4,−2)and(2,3,4). Find its vertices.
View SolutionThe mid-points of the sides of a triangle are (1,5,−1),(0,4,−2)and(2,3,4). Find its vertices.
View SolutionThe mid-points of the sides of a triangle are (1, 5, -1),(0,4,-2) and (2, 3, 4). Find its vertices.
View Solution
