Two springs of constants k1 and k2 have equal maximum velocities, when executing simple harmonic motion. The ratio of their amplitudes (masses are equal) will be
k1k2
(k1k2)1/2
k2k1
(k2k1)1/2
The correct Answer is:B
Since maximum velocity is Aω where ω is angular frequency,
∴A1ω1=A2ω2orA1A2=ω2ω1
But ω=√km∴A1A2=√k2√k1=√k2k1
Topper's Solved these Questions
- OSCILLATIONS AND WAVES BOOK - MTG GUIDECHAPTER - OSCILLATIONS AND WAVES EXERCISE - CHECK YOUR NEET VITALS
25 Videos
- OSCILLATIONS AND WAVES BOOK - MTG GUIDECHAPTER - OSCILLATIONS AND WAVES EXERCISE - AIPMT / NEET MCQs
43 Videos
- OSCILLATIONS AND WAVES BOOK - MTG GUIDECHAPTER - OSCILLATIONS AND WAVES EXERCISE - AIPMT / NEET MCQs
43 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - AIPMT / NEET (MCQs)
37 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - MTG GUIDECHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - AIPMT / NEET MCQ
14 Videos
Similar Questions
Two particles A and B of equal masses are suspended from two massless springs of spring constants K1andK2 respectively. If the maximum velocities during oscillations are equal. the ratio of the amplitude of A and B is
View SolutionThe figure shows a mass M attached to a series arrangement of two springs of spring constants k1andk2 where k2=2k1. If the mass M oscillates in simple harmonic motion with amplitude A, the amplitude of the point O is αAβ. Find β−α.
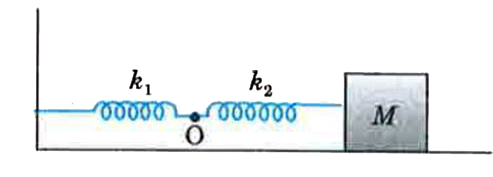
View Solution
Knowledge Check
Two springs, of spring constants k1 and K2, have equal highest velocities, when executing SHM. Then, the ration of their amplitudes (given their masses are in the ratio 1:2) will be
A2k1/k2B2k2/k1C√2k1/k2D√k2/2k1The maximum velocity a particle, executing simple harmonic motion with an amplitude 7 mm, 4.4 m//s. The period of oscillation is.
A(a) 0.01sB(b) 10sC(c ) 0.1sD(d) 100sThe phase of a particle executing simple harmonic motion is π2 when it has
AMaximum velocityBMaximum accelerationCMaximum energyDMaximum displacement
Two particles A and B of equal masses are suspended from two massless springs of spring constants k1 and k2, respectively, If the maximum velocities, during oscillation, are equal, the ratio of amplitude of A and B is
View SolutionFor a particle executing simple harmonic motion, the amplitude is A and time period is T. The maximum speed will be
View SolutionTwo bodies P&Q of equal mass are suspended from two separate massless springs of force constant k1&k2 respectively. If the maximum velocity of them are equal during their motion, the ratio of amplitude of P to Q is :
View SolutionTwo particle A and B of equal masses are suspended from two massless springs of spring constants k1 and k2, respectively. If the maximum velocities, during oscillations are equal, the ratio of amplitude of A and B is (4/3)×1000kg/m3. What relationship betwen t and t0 is ture?
View SolutionThe total mechanical energy of a particle executing simple harmonic motion is E when the displacement is half the amplitude of its kinetic energy will be
View Solution
