The mass M shown in figure ocillates in simple harmonic motion with amplitude A.
The amplitude of the point P is
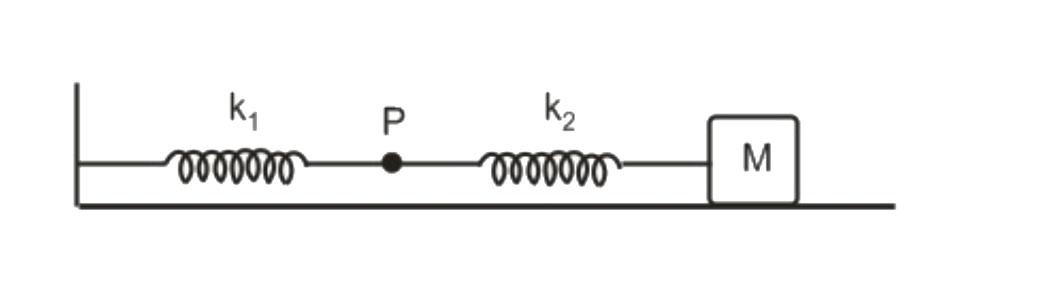
k1Ak2
k2Ak1
k1Ak1+k2
k2Ak1+k2
The correct Answer is:A
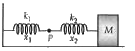
Here the two springs are in series. Let the mass M be pulled through small distance A towards right hand side. Due to it, let x1andx2 be the extension in two springs.
When two springs are in series, the tension or the force is same for both the springs. So k1x1=k2x2orx2=k1x1k2
Total extension, A=x1+x2=x1+k1x1k2,A=x1(k2+k1k2)
∴ Amplitude of point P = extension of spring k1
=x1=k2Ak1+k2
Topper's Solved these Questions
- OSCILLATIONS AND WAVES Book:MTG GUIDEChapter:OSCILLATIONS AND WAVES Exercise:CHECK YOUR NEET VITALS
Explore 25 Videos
- OSCILLATIONS AND WAVES Book:MTG GUIDEChapter:OSCILLATIONS AND WAVES Exercise:AIPMT / NEET MCQs
Explore 43 Videos
- OSCILLATIONS AND WAVES Book:MTG GUIDEChapter:OSCILLATIONS AND WAVES Exercise:AIPMT / NEET MCQs
Explore 43 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY Book:MTG GUIDEChapter:MOTION OF SYSTEM OF PARTICLES AND RIGID BODY Exercise:AIPMT / NEET (MCQs)
Explore 37 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:MTG GUIDEChapter:PHYSICAL WORLD AND MEASUREMENTExercise:AIPMT / NEET MCQ
Explore 14 Videos
Similar Questions
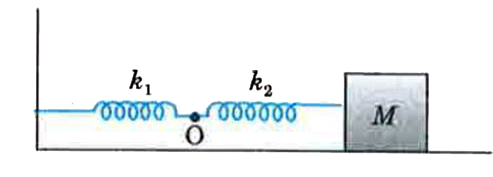
The figure shows a mass M attached to a series arrangement of two springs of spring constants k1andk2 where k2=2k1. If the mass M oscillates in simple harmonic motion with amplitude A, the amplitude of the point O is αAβ. Find β−α.
View SolutionA particle executes simple harmonic motion of amplitude A. At what points is its speed half the maximum speed ?
View Solution
Knowledge Check
The mass M shown in the figure oscillates in simple harmnonic motion and amplitude A. The amplitude of the point P is
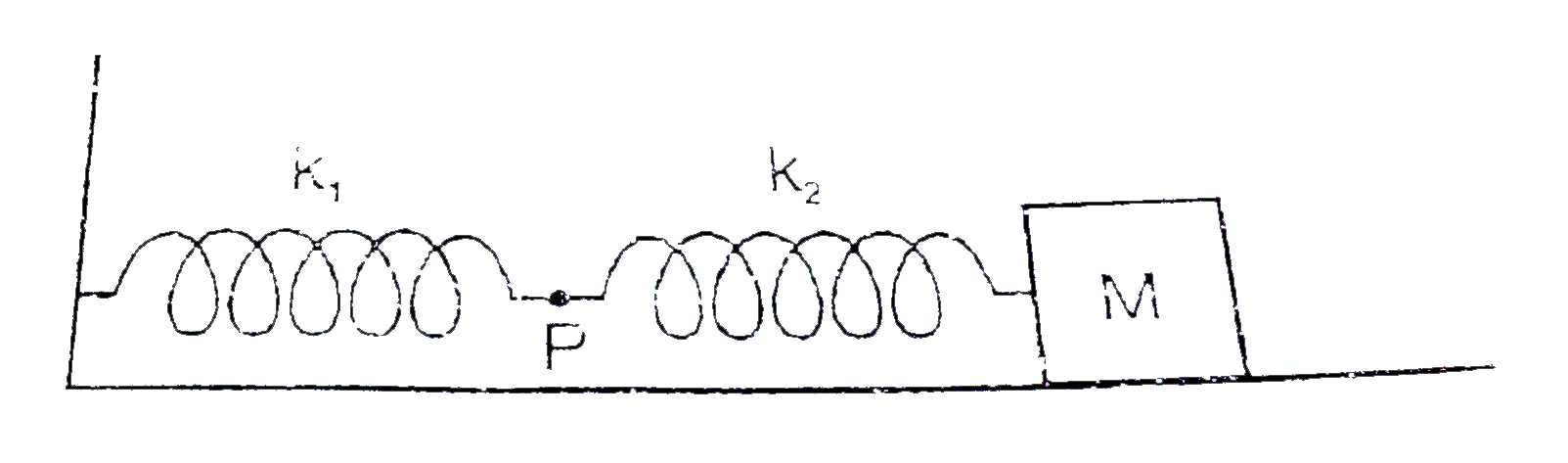 Ak1Ak2Bk2Ak1Ck1Ak1+k2Dk2Ak1+k2
Ak1Ak2Bk2Ak1Ck1Ak1+k2Dk2Ak1+k2The mass M shown in the figure oscillates in simple harmonic motion with amplitude A. The maximum speed of junction P is V0. Assume springs are massless. Select the correct option/s
 AAmplitude of potential energy is springs S1 and S2 is respectivley 19kA2 and kA2BAmplitude of potential energy is S1 and S2 is respectively 29kA2 and 19kA2CV0=A√8k27MDV0=A√8k81M
AAmplitude of potential energy is springs S1 and S2 is respectivley 19kA2 and kA2BAmplitude of potential energy is S1 and S2 is respectively 29kA2 and 19kA2CV0=A√8k27MDV0=A√8k81MA simple harmonic motino has amplitude A and time period T. The maxmum velocity will be
A4ATB2ATC2π√ATD2πAT
A particle is executing simple harmonic motion with an amplitude 9 cm. Locate the point where its speed be one-third of its maximum speed.
View SolutionA particle is executing linear simple harmonic motion of amplitude 'A'. The fraction of the total energy is the kinetic when the displacement is half of the amplitude is
View SolutionThe maximum velocity a particle, executing simple harmonic motion with an amplitude 7 mm, 4.4 m//s. The period of oscillation is.
View SolutionA mass m oscillates with simple harmonic motion with frequency f=ω2π and amplitude A on a spring of stiffness constant K. Which of the following is not correct ?
View SolutionThe equation of a simple harmonic motion (amplitude 5 cm and time period 0.5 second) is
View Solution

