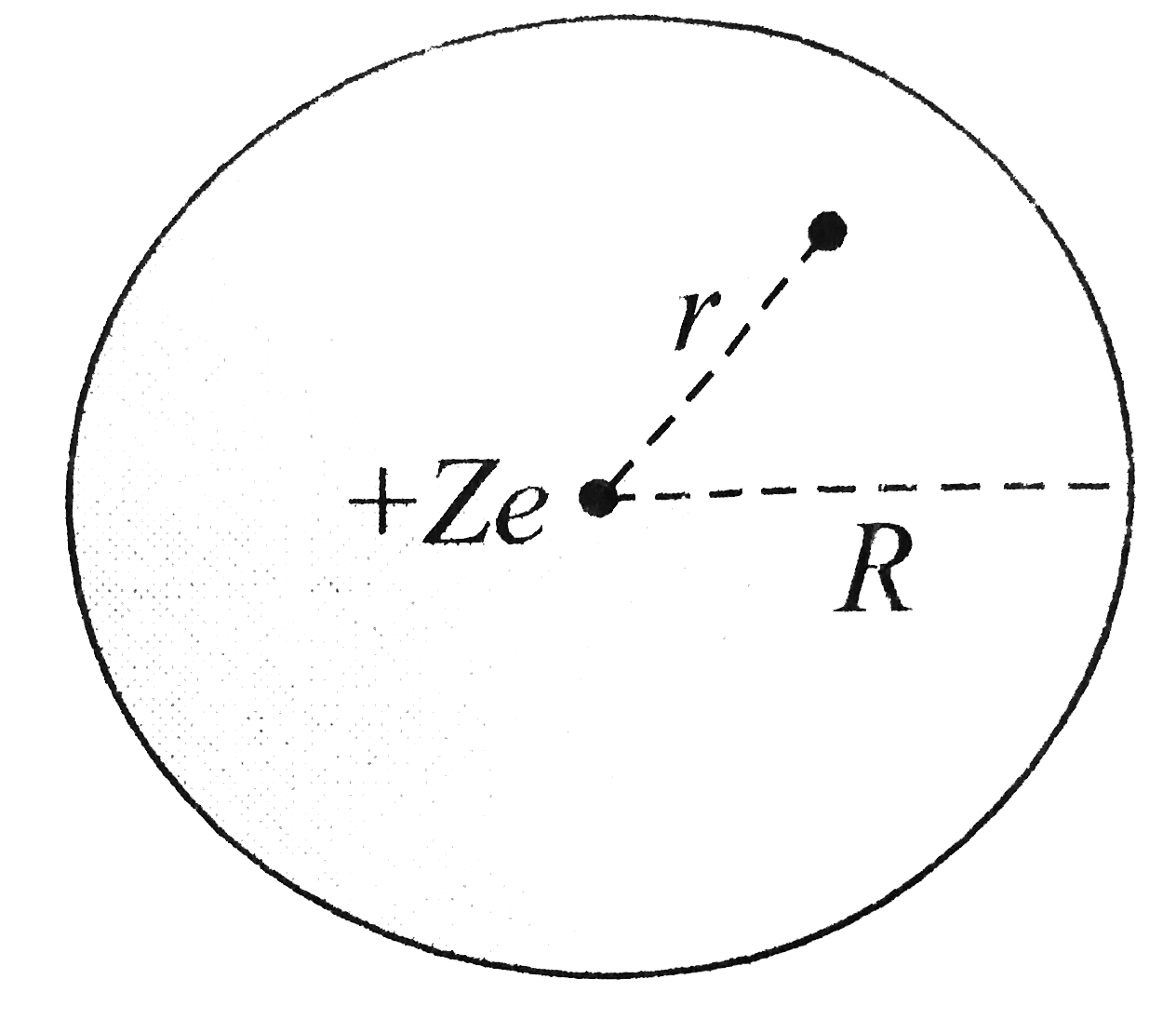
An early model for an atom considered it to have a positively charged point nucleus of charge Ze, surrounded by a uniform density of negative charge up to a radius R. The atom as a whole is neutral. For this model, what is the electric field at a distance r from the nucleus ?
 Solution in Gujrati
Solution in Gujrati
Here, nucleus of atom of an element with atomic no. Z has total positive charge + Ze (where + e is the charge of one proton) surrounding to this central positive point charge, uniform volume distribution of equal negative charge was considered up to radius R, as per the early model of neutral atom.
If volume charge density of this negative charge is then,
.............(1)
Now let us find out electric field for following three cases.
(i) For (inside the atom)
Consider spherical Gaussian surface with radius and surface area . Magnitude of electric field at any point on this surface is same because of spherical symmetry. Let it be ,
Here net charge enclosed by above Gaussian surface is,
(From equation (1))
.........(2)
Applying Gauss theorem for considered Gaussian surface,
........(3)
Above electric field is directed radialh outward.
(ii) For (outside the atom)
Consider spherical Gaussian surface witri radius and surface area . Magnimde of electric field at any point on this surface! is same because of spherical symmetry. Let it be
Here, net charge enclosed by above Gaussian surface,
...........(4)
(iii) On the surface of atom (r = R)
If we consider spherical Gaussian surface with radius r = R then charge enclosed by it is also q = Ze + (-Ze) = 0 and so electric field on the surface of atom is .
From equation (3) also, we get electric field on the surface of atom,
(For r=R)
Conclusion :
(i) At distance from the centre of atom, electric field is,
(Radially outward)
(ii) At distance from the centre of atom, electric field is,
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION B NUMERICALS (NUMERICAL FROM TEXTUAL EXERCISES)
Explore 24 Videos
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION B NUMERICALS (ADDITONAL EXERCISE)
Explore 10 Videos
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION A QUESTIONS- ANSWERS (TRY YOURSELF )
Explore 73 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:KUMAR PRAKASHANChapter:DUAL NATURE OF RADIATION AND MATTERExercise:Section-D (MCQs asked in GUJCET/Board Exam)
Explore 1 Video
- ELECTROMAGNETIC INDUCTIONBook:KUMAR PRAKASHANChapter:ELECTROMAGNETIC INDUCTIONExercise:Section D MCQs (MCQs asked in Competitive Exams )
Explore 38 Videos
Similar Questions
What is the force between two small charged spheres having charges of 2×10−7C and 3×10−7 C placed 30 cm apart in air ?
View SolutionAn oil drop of 12 excess electrons is held stationary under a constant electric field of 2.55×104NC−1. (Millikan's oil drop experiment). The density of the oil is 1.26gcm−3. Estimate the radius of the drop, (g=9.81ms−2,e=1.60×10−19C).
View SolutionWhy spark is seen while removal of synthetic clothes in dark in winter?
View SolutionMass of photon in motion is ………
View SolutionThree pure inductance each of 3 H are connected as shown in figure. The equivalent inductance of this connection between points A and B is ......

View SolutionAccording to early model of an atom, the atom is considered it to have a positively charged point nucleus of charge Ze surrounded by a uniform density of negative charge up to a radius R. The atom as a whole is neutral. The electric field at a distance r from the nucleus is (r<R)
View SolutionAn early model for an atom considered it to have a positively charged point nucleas of charge Ze, surrounded by a unifrom density of negative charge upto a radius R. The atom as a whole is neutral. For this model, What is the electric field at a distance r from the nucleas ?
View Solutionप्रारम्भ में परमाणु के मॉडल (atomic model) के लिए यह माना गया था कि प्रत्येक परमाणु +Ze आवेशयुक्त एक बिन्दुवत नाभिक (nucleus) होता है जो R त्रिज्या के गोले (sphere) तक उसी परिमाण के ऋण आवेश से एकसमान रूप से (uniformly) घिरा है ताकि परमाणु पूर्णरुमेण उदासीन (neutral) हो। इस मॉडल के लिए नाभिक से r दूरी पर विधुत-क्षेत्र ज्ञात करें, यदि (i) r>R तथा (ii) r<R
View SolutionAccording to early model of an atom,the atom is considered it to have a positively charged point nucleus of charge Ze surrounded by a uniform density of negative charge up to a radius R. The atom as a whole is neutral. The electric field at a distancer from the nucleus is (r<R)

View SolutionThe classical concept of atomic structure is that negative charges are uniformly distributed inside a sphere of radius R keeping the nucleus of positive charge Ze at the centre of that sphere. An atom as a whole is electrically neutral. find out the electric field at a distance r from the nucleus, according do this atomic model.
View Solutionपरमाणु के प्रारंभिक प्रतिरूप में यह माना गया था कि आवेश Ze का बिंदु आमाप का घनात्मक नाभिक होता है जो त्रिज्या तक एकसमान घनत्व के ऋणावेश से घिरा हुआ है। परमाणु पूर्ण रूप में विधुत उदासीन है। इस प्रतिरूप के नाभिक से r दूरी पर विधुत क्षेत्र कितना है ?
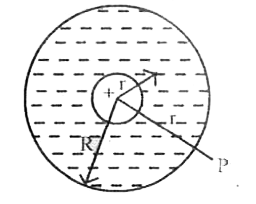
View SolutionAn early model for an atom considered to have a positively charged point nucleus of charge Ze surrounded by a uniform density of negative charge up to a radius R. The atom as a whole is neutral. For this model, what is the electric field a distance r from the nucleus, where r>R ?
View SolutionAn early model for an atom consider it to have a positively charged point inucleus of charges ze surronded by a whole is neutral for this model what is the electric field at a distance r from the nucleus
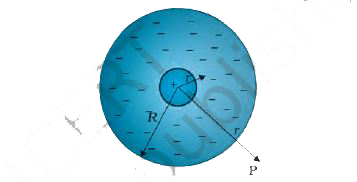
View SolutionAn early model for an atom considered it to have a positively charged point nucleus of charge Ze, surrounded by a uniform density of negative charge up to a radius R. The atom as a whole is neutral. For this model, what is the electric field at a distance r from the nucleus ?

View SolutionAn atom was earlier assumed to be sphere of radius a having a positively charged point nucleus of charge +Ze at its centre . This nucleus was believed to be surrounded by a uniform desity of negative charge that made the atom neutral as a whole.
Use this theorem to find the electric field of this atom at a distance r(r>a) from the centre of the atom.
View Solution