An electron falls through a distance of 1.5cm in a uniform electric field of magnitude 2×104NC−1. The direction of the field is reversed keeping its magnitude unchanged and a proton falls through the same distance. Compute the time of fall in each case.Solution in Gujrati
Here, electric force, Fe=qE=ma⇒a=qEm
Linear distance travelledl at the end of time t is,
d=v0t+12at2
∴d=12qEmt2,(∴v0=0 and a=qEm)
∴t2=2mdqE
∴t=√2mdeE......(1)
(∴ For electron and proton magnitude of charge is q=e)
Linear distance travelled at the end of time t is,
d=v0t+12at2
∴d=12(qEm)t2(∴v0=0 and a=qEm)
∴t2=2mdqE
∴t=√2mdeE............(1)
(∴ For electron and proton magnitude of charge is q = e)
From equation (1), time taken by electron to pass through distance d (opposite to electric field)
te=√2medeE
=√2×9.1×10−31×0.0151.6×10−19×2×104
=2.92×10−9 s
∴te=2.92ns (Nano second)...........(2)
From equation (1), time taken by proton to pass through distance d (parallel to electric field)
tp=√2mpdeE
=√2×1.67×10−27×0.0151.6×10−19×2×104
=1.251×10−7s
=125.1×10−9s
∴tp=125.1ns.............(3)
From equation (2) and (3),
tp>te (∴ Here, t∝√m and mp>me)
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION B NUMERICALS (NUMERICAL FROM TEXTUAL EXERCISES)
24 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION B NUMERICALS (ADDITONAL EXERCISE)
10 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION A QUESTIONS- ANSWERS (TRY YOURSELF )
73 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - KUMAR PRAKASHANCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section-D (MCQs asked in GUJCET/Board Exam)
1 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section D MCQs (MCQs asked in Competitive Exams )
38 Videos
Similar Questions
What is the force between two small charged spheres having charges of 2×10−7C and 3×10−7 C placed 30 cm apart in air ?
View SolutionAn oil drop of 12 excess electrons is held stationary under a constant electric field of 2.55×104NC−1. (Millikan's oil drop experiment). The density of the oil is 1.26gcm−3. Estimate the radius of the drop, (g=9.81ms−2,e=1.60×10−19C).
View SolutionWhy spark is seen while removal of synthetic clothes in dark in winter?
View SolutionMass of photon in motion is ………
View SolutionThree pure inductance each of 3 H are connected as shown in figure. The equivalent inductance of this connection between points A and B is ......

View SolutionAn electron (mass m_(e ) )falls through a distance d in a uniform electric field of magnitude E. , The direction of the field is reversed keeping its magnitudes unchanged, and a proton(mass m_(p) ) falls through the same distance. If the times taken by the electrons and the protons to fall the distance d is t_("electron") and t_("proton") respectively, then the ratio t_("electron")//t_("proton") .
View SolutionAn electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
View SolutionAn electron falls through a distance of 1.5 cm in a uniform electric field of magnitude 2.4xx10^(4) NC^(-1) [Fig.1.12 (a)] . The direction of the field is reversed keeping its magnitude unchagned and a proton falls through the same distance [Fig. 1.12 (b) ]. Complute the time of fall in each case. Contrast the situation (a) with that of free fall under gravity.
View SolutionA proton falls dwon through a distance of 2 cm in a uniform electric field of magnitude 3.34xx10^(3) NC^(-1) . Determine (i) the acceleration of the electron (ii) the time taken by the proton to fall through the distance of 2 cm, and (iii) the direction of the electric field.
View SolutionAn electron falls through a distance of 1.5 cm in a uniform electric field of magnitude 2.0×104NC−1. The direction of the field is reversed keeping its magnitude unchanged and a proton falls through the same distance. Compute the time of fall in each case. Contrast the situation with that of 'free fall under gravity'.

View Solutionकोई इलेक्ट्रॉन विरामावस्था से किसी एकसमान तथा। ऊपर की ओर ऊध्याधर C विद्युत क्षेत्र E में दी गई दूरी। तक गिरता है। अब विद्युत क्षेत्र का परिमाण अपरिवा रखते हुए इसकी दिशा उत्क्रमित कर दी जाती है। किसी तक प्रोटॉन को विरामावस्था से उतनी ही ऊर्ध्वाधर दरी h तक. इसमें गिरने दिया जाता है। प्रोटॉन के गिरने में लिए गए समय की तुलना में इलेक्ट्रॉन द्वारा गिरने में लिया गया समय है-
View SolutionAn electron falls through distance of 1.5 cm in a uniforth electric field of magnitude 2.0xx 10 ^(4) NC^(-1) The direction of the field is reversed keeping its magnitude unchanged and a proton falls through the same distance compute the time of falls in each case . Contrast the situation with that of 'free fall under gravity'
View SolutionAn electron falls throgh a distance of 1.5 cm in a uniform electric field of magnitude 2.0 xx10^(4) N c^(-1) the direction of the field is reversed keeping its magnitude unchanged and a proton falls through the same distance compute the time of falls in each case contrast the situation with that of free fall under gravity
View SolutionAn electron falls throgh a distance of 1.5 cm in a uniform electric field of magnitude 2.0×104Nc−1 the direction of the field is reversed keeping its magnitude unchanged and a proton falls through the same distance compute the time of falls in each case contrast the situation with that of free fall under gravity
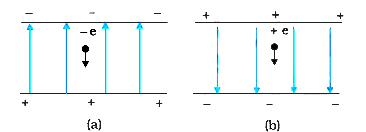
View SolutionAn electron falls through a distance of 1.5 cm in a uniform electric field of magnitude 2.4×104NC−1 [Fig.1.12 (a)]. The direction of the field is reversed keeping its magnitude unchagned and a proton falls through the same distance [Fig. 1.12 (b) ]. Complute the time of fall in each case. Contrast the situation (a) with that of free fall under gravity.
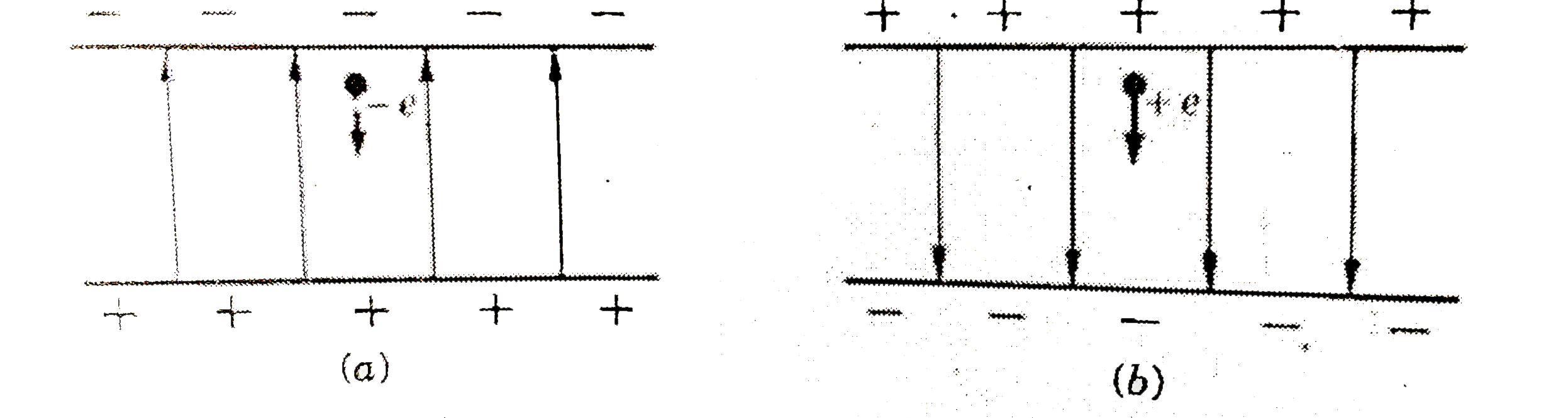
View Solution
