A 55.0-kg skateboarder starts out with a speed of 1.80 m/s. He does +80.0J of work on himself by pushing with his feet against the ground. In addition, friction does −265J of work on him. In both cases, the forces doing the work are non-conservative. The final speed of the skateboarder is 6.00 m/s. Caculate the change [Δ(P.E.)=(P.E.)f−(P.E.)0] in the gravitational potential energy .
−1246J
−1086J
−821J
−716J
The correct Answer is:B
To calculate the change in gravitational potential energy (ΔP.E.) of the skateboarder, we can follow these steps:
Step 1: Calculate the initial kinetic energy (KEi)
The initial kinetic energy can be calculated using the formula:
KEi=12mv2i
Where:
- m=55.0kg (mass of the skateboarder)
- vi=1.80m/s (initial speed)
Calculating:
KEi=12×55.0kg×(1.80m/s)2
KEi=12×55.0×3.24=89.1J
Step 2: Calculate the final kinetic energy (KEf)
The final kinetic energy can be calculated using the same formula:
KEf=12mv2f
Where:
- vf=6.00m/s (final speed)
Calculating:
KEf=12×55.0kg×(6.00m/s)2
KEf=12×55.0×36=990J
Step 3: Calculate the change in kinetic energy (ΔKE)
The change in kinetic energy is given by:
ΔKE=KEf−KEi
Calculating:
ΔKE=990J−89.1J=900.9J
Step 4: Calculate the total work done (Wtotal)
The total work done on the skateboarder is the sum of the work done by the skateboarder and the work done against friction:
Wtotal=Wself+Wfriction
Where:
- Wself=+80.0J
- Wfriction=−265.0J
Calculating:
Wtotal=80.0J−265.0J=−185.0J
Step 5: Use the work-energy principle to find ΔP.E.
According to the work-energy principle:
Wtotal=ΔKE+ΔP.E.
Rearranging gives us:
ΔP.E.=Wtotal−ΔKE
Calculating:
ΔP.E.=−185.0J−900.9J=−1085.9J
Conclusion
The change in gravitational potential energy is:
ΔP.E.≈−1086J
The initial kinetic energy can be calculated using the formula:
KEi=12mv2i
Where:
- m=55.0kg (mass of the skateboarder)
- vi=1.80m/s (initial speed)
KEi=12×55.0kg×(1.80m/s)2
KEi=12×55.0×3.24=89.1J
The final kinetic energy can be calculated using the same formula:
KEf=12mv2f
Where:
- vf=6.00m/s (final speed)
KEf=12×55.0kg×(6.00m/s)2
KEf=12×55.0×36=990J
The change in kinetic energy is given by:
ΔKE=KEf−KEi
Calculating:
ΔKE=990J−89.1J=900.9J
The total work done on the skateboarder is the sum of the work done by the skateboarder and the work done against friction:
Wtotal=Wself+Wfriction
Where:
- Wself=+80.0J
- Wfriction=−265.0J
Wtotal=80.0J−265.0J=−185.0J
According to the work-energy principle:
Wtotal=ΔKE+ΔP.E.
Rearranging gives us:
ΔP.E.=Wtotal−ΔKE
Calculating:
ΔP.E.=−185.0J−900.9J=−1085.9J
The change in gravitational potential energy is:
ΔP.E.≈−1086J
Topper's Solved these Questions
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (More than one choice correct type)
10 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Linked Comprehension)
9 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PROBLEMS
58 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)
4 Videos
Similar Questions
A skier is pulled by towrope up frictionless ski slope that makes an angle of 12∘ with the horizontal . The rope moves parallel to the slope with a constant speed of 1.0 m/s. The force of the rope does 880 J of work on the skier as the skier moves a distance of 7.0 m up the incline. (a) If the rope moved with a constant speed of 2.0 m/s, how much work would the force of the rope do on the skier as the skier moved a distance of 8.0 m up the incline ? At what rate is the force of the rope doing work on the skier when the rope moves with a speed of (b) 1.0 m/s and (c) 2.0 m/s ?
View SolutionA 1.50 kg snowball is fired from a cliff 11.5 m high. The snowball's initial velocity is 16.0 m/s, directed to 41.0∘ above the horizontal . (a) How much work is done on the snowball by the gravitational force during its flight to the flat ground below the cliff ? (b) What is the change in the gravitational potential energy of the snowball-Earth system during the flight ? (c) If that gravitational potential energy is taken to be zero at the height of the cliff, what is its value when the snowball reaches the ground ?
View Solution
Knowledge Check
A pitcher throws a 0.140 -kg baseball, and it approaches the bat at a speed of 40.0 m/s. The bat does Wnc=70.0J of work on the ball in hitting it. Ignoring air resistance, determine the speed of the ball after the ball leaves the bat and is 25.0 m above the point of impact.
A23.4m/sB48.5m/sC45.9m/sD51.0m/sA particle of mass 1 kg slides in a horizontal circle of radius 20 m with a constant speed of 1m/s. The only forces in the vertical direction acting on the particle are its weight and the normal reaction, however no information is available about the forces in the horizontal plane. Over a period of time whole energy dissipated due to work done by friction is conducted to ground and simultaneously radiated to surround. If the coefficient of friction is μ=0.5. Then (Take g =10 m//s^(2):
AThe magnitude of frictional force acting on the block must be 5 NBThe frictional force must be in tangential direction.CThe frictional force must be towards the centre.DNo comment can be made about the direction or magnitude of friction based on the given data.A 55-kg box is being pushed a distance of 7.0 m across the floor by a force →p whose magnitude is 150 N. The force →P is parallel to the displacement of the box. The coefficient of kinetic friction is 0.25. Determine the work done on the box by each of the four forces that act on the box. Be sure to include the proper plus or minus sign for the work done by each force. ( The four forces and the force P, the frictional force f, the normal force N, and the force due to gravity mg. )
AWpWfWNWmg1.0×103J−940J−3.8×103J3.8×103JBWpWfWNWmg1.0×103−940JzeroJzeroJCWpWfWNWmgzeroJ940J−3.8×103J3.8×103JDWpWfWNWmg−1.0×103JzeroJzeroJ−3.8×103J
In figure, k=100N/m,M=1kg and F=10N
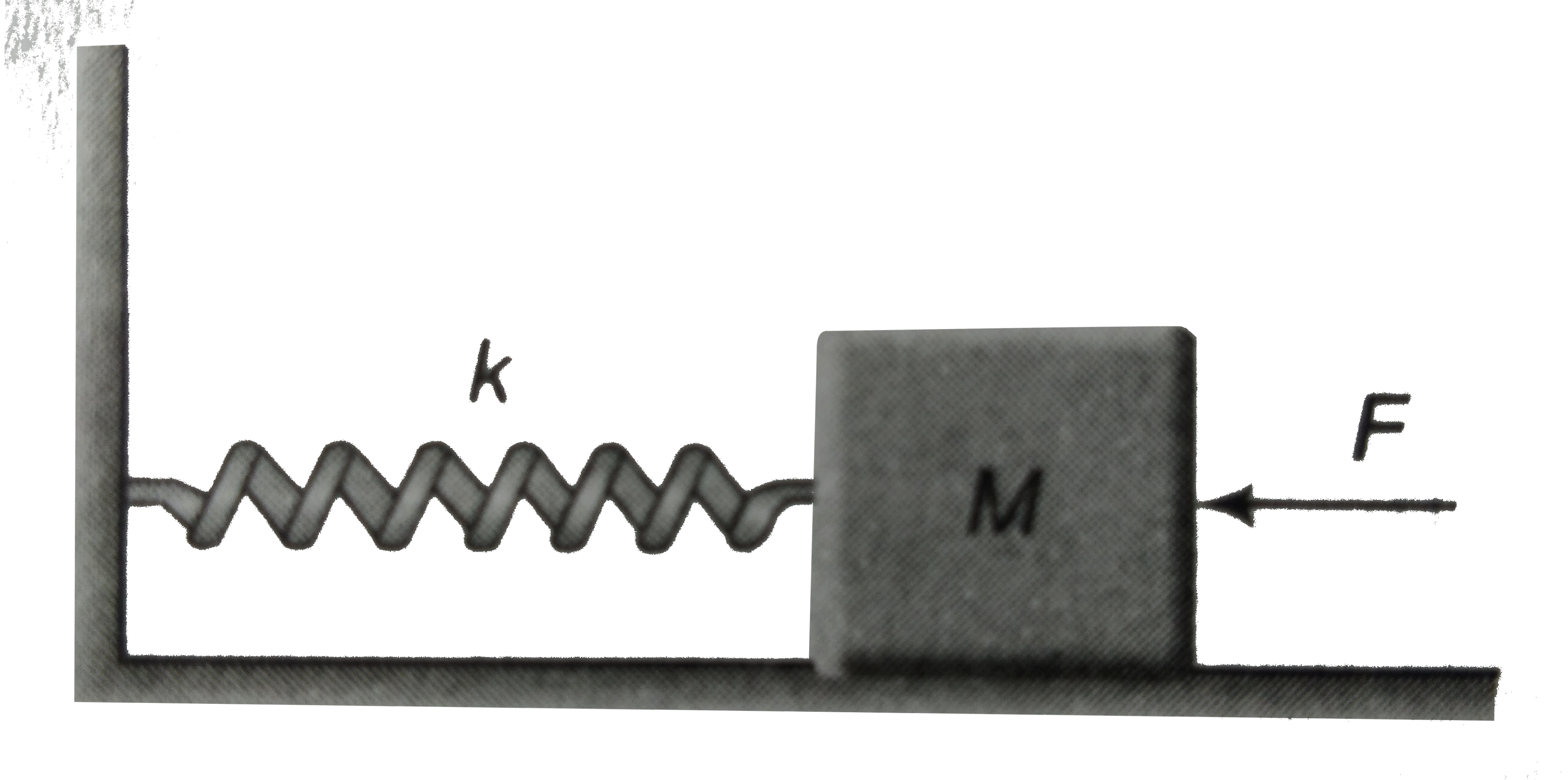
(a) Find the compression of the spring in the equilibrium position
(b) A sharp blow by some external agent imparts a speed of 2m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
(c) Find the time period of the resulting simple harmonic motion.
(d) Find the amplitude.
(e) Write the potential energy of the spring when the block is at the left estreme.
(f) Write the potential energy of the spring when the block is at the right extreme.
The answers of (b), (e) and (f) are different. Explain why this does not violate the principle of conservation of energy ?
View SolutionThe gravitational field in a certain region is given as
→E=(3Nkg−1)ˆi+(5Nkg−1)ˆj
(a) What will be magnitude of gravitational force acting on a particle of mass 3 g which is placed at the origin ?
(b) What will be gravitational potential at point (12m, 0) and (0, 6m) considering that potential at origin is zero ?
(c ) A particle of mass 3 kg is moved from the origin to the point (9m, 4m). Calculate the change of gravitational potential energy.
(d) If the above particle is moved from (5m, 0) to (0, 5m), then find the change in potential energy.
View SolutionThe loaded cab of an elevator has a mass of 5.0×103 kg and moves 210 m up the shaft in 23 s at constant speed. At what average rate does the force from the cable do work on the cab ?
View SolutionA uriform chain hangs over the edge of a horizontal platform. A machine does 1.0 J of work in pulling the rest of the chain onto the platform. The chain has a mass of 2.0 kg and a length of 3.0 m. What length was initially hanging over the edge ? On the Moon, the gravitational acceleration is 1/6 of 9.8m/s2.
View SolutionIn a spring gun, a spring of force constant 200N/mis compressed 0.15 m. When fired, 80% of the elastic potential energy stored in the spring is eventually converted into kinetic energy of a 0.1 kg uniform ball that is rolling without slipping at the base of a ramp. The ball continues to roll without slipping up the ramp with 90% of the kinetic energy at the bottom converted into an increase in gravitational potential energy at the instant it stops.
(a) What is the speed of the ball's centre ofmass at the base of the ramp
(b) At this position, what is the speed of a point at the top of the ball?
(c ) What maximum vertical height up the ramp does the ball move?
View Solution
