How much power is needed to lift a 75- kg student vertically upward at a constant speed of 0.33 m/s ?
12.5W
115W
242.5
230W
The correct Answer is:C
To find the power needed to lift a 75 kg student vertically upward at a constant speed of 0.33 m/s, we can follow these steps:
Step 1: Identify the Force Required
The force required to lift the student is equal to the weight of the student, which can be calculated using the formula:
F=m⋅g
where:
- m=75kg (mass of the student)
- g=9.8m/s2 (acceleration due to gravity)
Step 2: Calculate the Weight
Now we can calculate the weight:
F=75kg⋅9.8m/s2
F=735N
Step 3: Use the Power Formula
Power is defined as the rate of doing work. When lifting an object at a constant speed, the power can be calculated using the formula:
P=F⋅v
where:
- P = power (in watts)
- F = force (in newtons)
- v = velocity (in m/s)
Step 4: Substitute Values into the Power Formula
Now we can substitute the values we have:
P=735N⋅0.33m/s
Step 5: Calculate the Power
Now, we perform the multiplication:
P=735⋅0.33
P=242.55W
Conclusion
The power needed to lift the 75 kg student vertically upward at a constant speed of 0.33 m/s is approximately:
P≈242.5W
The force required to lift the student is equal to the weight of the student, which can be calculated using the formula:
F=m⋅g
where:
- m=75kg (mass of the student)
- g=9.8m/s2 (acceleration due to gravity)
Now we can calculate the weight:
F=75kg⋅9.8m/s2
F=735N
Power is defined as the rate of doing work. When lifting an object at a constant speed, the power can be calculated using the formula:
P=F⋅v
where:
- P = power (in watts)
- F = force (in newtons)
- v = velocity (in m/s)
Now we can substitute the values we have:
P=735N⋅0.33m/s
Now, we perform the multiplication:
P=735⋅0.33
P=242.55W
The power needed to lift the 75 kg student vertically upward at a constant speed of 0.33 m/s is approximately:
P≈242.5W
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (More than one choice correct type)10videos
WORK, POWER, AND ENERGY
- BOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Linked Comprehension)9videos
WORK, POWER, AND ENERGY
- BOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PROBLEMS58videos
WORK, POWER, AND ENERGY
- BOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)4videos
WAVES-I
Similar Questions
A block is.moving in horizontal circular motion with constant speed of 10ms (with respect to lift) about a point O on the smooth surface of the lift. The lift is moving upward with constant speed of 10ms and the length of string of the lift is 2m, The radius of curvature (in m ) of the motion of block with respect to ground is
'(##CEN_KSR_PHY_JEE_C07_038_Q23##)'View SolutionA 15.0 kg package is moving at a speed of 10.0 m/s vertically upward along a y axis when it explodes into three fragments: a 2.00 kg fragment is shot upward with an initial speed of 20.0 m/s and a 3.00 kg fragment is shot in the positive direction of a horizontal x axis with an initial speed of 5.00 m/s. Find (a) the speed of the third fragment right after the explosion and (b) the total kinetic energy provided by the explosion.
View Solution
Knowledge Check
- Question 1 - Select One
What power is needed to lift a 49-kg person a vertical distance of 5.0 m in 20.0 s ?
A12.5WB60WC25WD120W - Question 2 - Select One
The KE and PE of the ball half way up, when a ball of mass 0.1 kg is thrown vertically upwards with an initial speed of 20 m/s is
A10 J, 20 JB15 J, 8JC8 J, 16 JD10 J, 10 J - Question 3 - Select One
An open lift is coming down from the top of building at a constant speed v=10m/s. A boy standing on the lift throws a stone vertically upwards at a speed of 30 m/s w.r.t. himself. The time after which he will catch the stone is:
A4 secB6 secC8 secD10 sec
A person in an elevator accelerating upwards with an acceleration of 2 ms−2, tosses a coin vertically upwards with a speed of 20 ms−1. After how much time will the coin fall back into his hand ?
( take g=10ms−2)View SolutionA chain consisting of 5 links each of mass 0.1 kg is lifted vertically up with a constant acceleration of 2.5m/s2. The force of interaction between 1st and 2nd links as shown
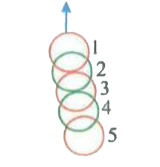 View Solution
View SolutionA stone of 3 kg is thrown vertically upward with an initial speed of 20 m/s. If g=10m/s2, then find the kinetic energy and potential energy after 1 s.
View SolutionA chain consisting of 5 links each of mass 0.1 kg is lifted vertically with a constant acceleration of 2.5m/s2 as shown in the figure. The force of interaction between the top link and the link immediately below it, will be
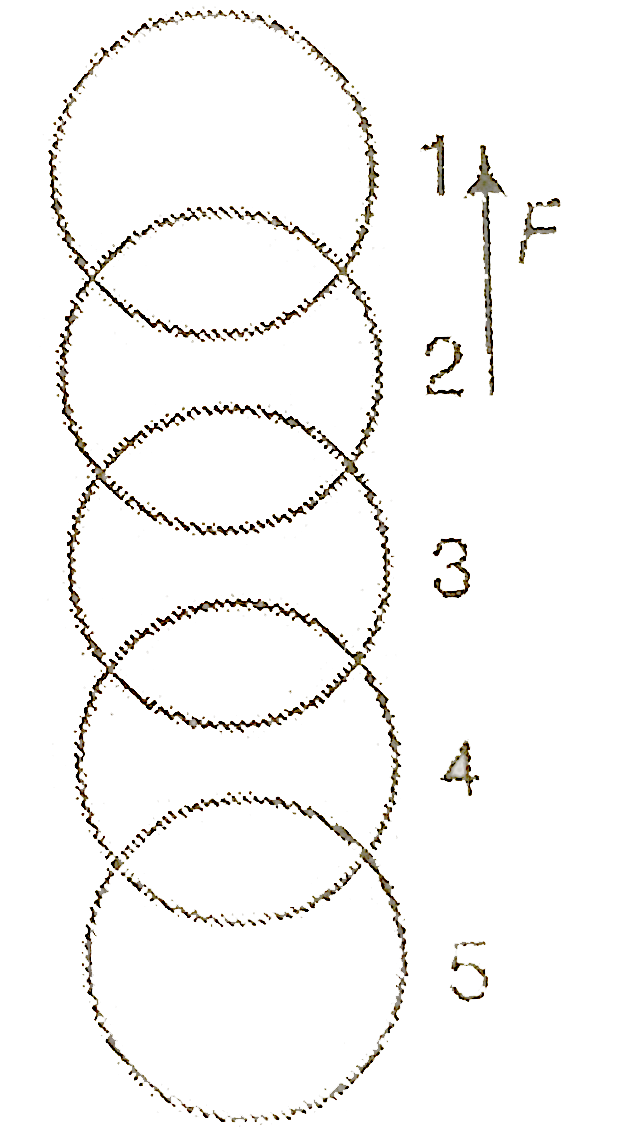 View Solution
View SolutionA stone of 3kg is thrown vertically upward with an initial speed of 20m/s. If g=10m/s2, then find the kinetic energy and potential energy after 1s.
View Solution
