A 63-kg skier goes up a snow-convered hill that makes an angle of 25∘ with the horizontal . The initial speed of the skier is 6.6 m/s. After coasting a distance of 1.9 m up the slope, the speed of the skier is 4.4 m/s. Find the work done by the kinetic frictional force that acts on the skis .
−760J
+270J
+300J
−270J
The correct Answer is:D
To find the work done by the kinetic frictional force acting on the skier, we can use the work-energy principle. According to this principle, the work done by all forces acting on an object is equal to the change in its kinetic energy plus the change in its potential energy.
1. Identify Given Data:
- Mass of the skier, m=63kg
- Initial speed, vi=6.6m/s
- Final speed, vf=4.4m/s
- Distance traveled up the slope, d=1.9m
- Angle of the slope, θ=25∘
2. Calculate Initial Kinetic Energy (KE_initial):
KEinitial=12mv2i=12×63×(6.6)2
KEinitial=12×63×43.56=1377.78J
3. Calculate Final Kinetic Energy (KE_final):
KEfinal=12mv2f=12×63×(4.4)2
KEfinal=12×63×19.36=609.36J
4. Calculate Change in Potential Energy (PE):
The change in potential energy when the skier goes up the slope is given by:
PE=mgh=mgdsin(θ)
where g=10m/s2 (approximate value for gravitational acceleration).
PE=63×10×1.9×sin(25∘)
First, calculate sin(25∘):
sin(25∘)≈0.4226
Now, substituting the values:
PE=63×10×1.9×0.4226≈507.87J
5. Apply the Work-Energy Principle:
According to the work-energy principle:
Wfriction+KEinitial=KEfinal+PE
Rearranging gives:
Wfriction=KEfinal+PE−KEinitial
Substituting the values:
Wfriction=609.36+507.87−1377.78
Wfriction=1117.23−1377.78=−260.55J
6. Final Result:
The work done by the kinetic frictional force is approximately:
Wfriction≈−270J
- Mass of the skier, m=63kg
- Initial speed, vi=6.6m/s
- Final speed, vf=4.4m/s
- Distance traveled up the slope, d=1.9m
- Angle of the slope, θ=25∘
KEinitial=12mv2i=12×63×(6.6)2
KEinitial=12×63×43.56=1377.78J
KEfinal=12mv2f=12×63×(4.4)2
KEfinal=12×63×19.36=609.36J
The change in potential energy when the skier goes up the slope is given by:
PE=mgh=mgdsin(θ)
where g=10m/s2 (approximate value for gravitational acceleration).
PE=63×10×1.9×sin(25∘)
First, calculate sin(25∘):
sin(25∘)≈0.4226
Now, substituting the values:
PE=63×10×1.9×0.4226≈507.87J
According to the work-energy principle:
Wfriction+KEinitial=KEfinal+PE
Rearranging gives:
Wfriction=KEfinal+PE−KEinitial
Substituting the values:
Wfriction=609.36+507.87−1377.78
Wfriction=1117.23−1377.78=−260.55J
The work done by the kinetic frictional force is approximately:
Wfriction≈−270J
Topper's Solved these Questions
- WORK, POWER, AND ENERGYBook:RESNICK AND HALLIDAYChapter:WORK, POWER, AND ENERGYExercise:PRACTICE QUESTIONS (More than one choice correct type)
Explore 10 Videos
- WORK, POWER, AND ENERGYBook:RESNICK AND HALLIDAYChapter:WORK, POWER, AND ENERGYExercise:PRACTICE QUESTIONS (Linked Comprehension)
Explore 9 Videos
- WORK, POWER, AND ENERGYBook:RESNICK AND HALLIDAYChapter:WORK, POWER, AND ENERGYExercise:PROBLEMS
Explore 58 Videos
- WAVES-IBook:RESNICK AND HALLIDAYChapter:WAVES-IExercise:Practice Questions (Integer Type)
Explore 4 Videos
Similar Questions
A skier is pulled by towrope up frictionless ski slope that makes an angle of 12∘ with the horizontal . The rope moves parallel to the slope with a constant speed of 1.0 m/s. The force of the rope does 880 J of work on the skier as the skier moves a distance of 7.0 m up the incline. (a) If the rope moved with a constant speed of 2.0 m/s, how much work would the force of the rope do on the skier as the skier moved a distance of 8.0 m up the incline ? At what rate is the force of the rope doing work on the skier when the rope moves with a speed of (b) 1.0 m/s and (c) 2.0 m/s ?
View SolutionA particle of mass 20 g is thrown vertically upwards with a speed of 10 m/s. Find the work done by the force of gravity during the time the particle goes up.
View Solution
Knowledge Check
If a body is thrown with a speed of 19.6m/s making an angle of 30∘ with the horizontal,then the time of flight is
A1sB2sC2√3sD5sA block of mass 1 kg slides down a vertical curved track that is one quadrant of a circle of radius 1m. Its speed ast the the bottom si 2m/s. The work done by frictional force is :
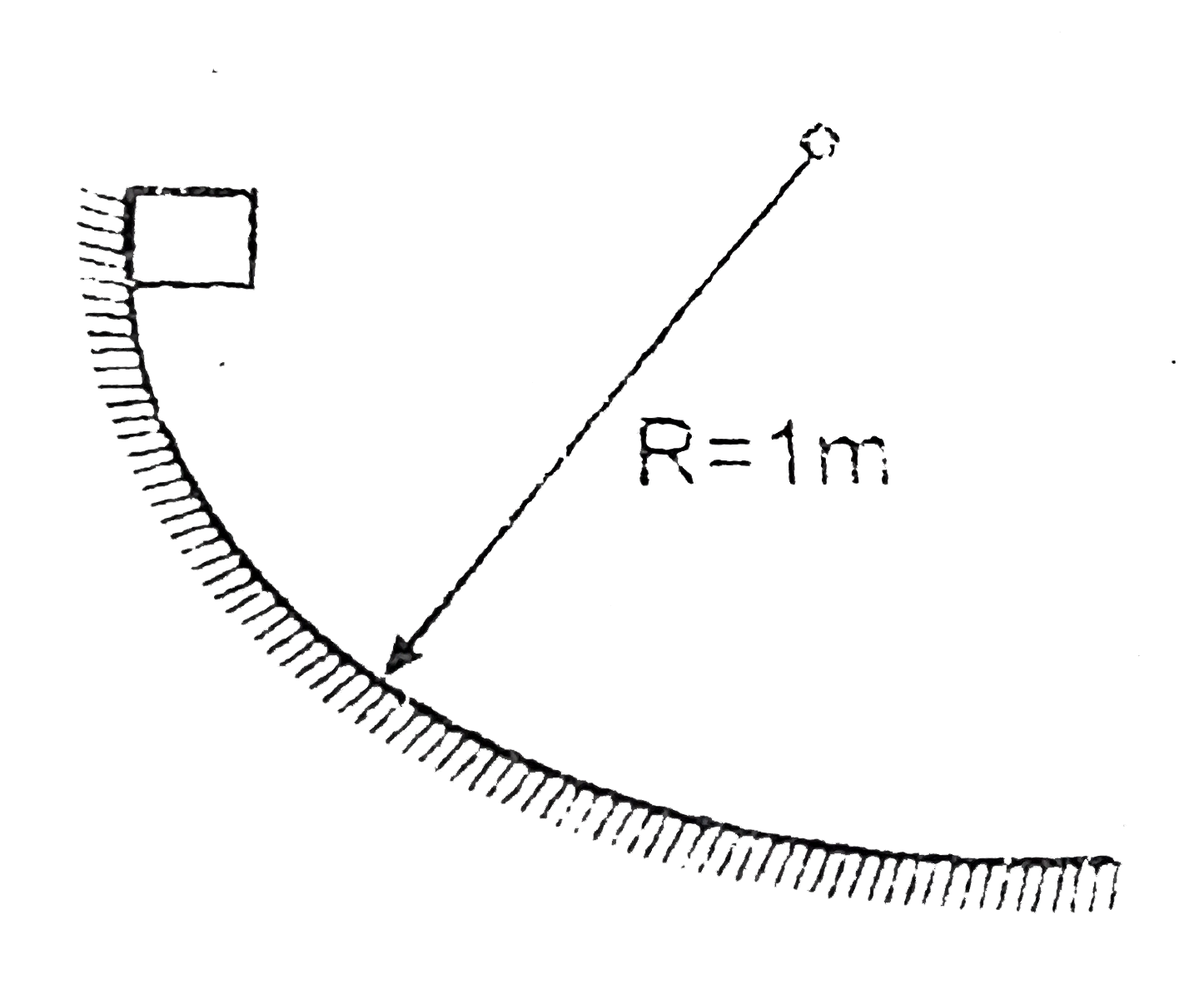 A8JB−8JC4JD−4J
A8JB−8JC4JD−4JA force increases the speed of a 1.0 kg object from 4 m/s to 8m/s . The work done by the force will be ____.
A8JB32JC24JD16J
A uniform disc of mass m and radius R is rolling up a rough inclined plane which makes an angle of 30∘ with the horizontal. If the coefficients of static and kinetic friction are each equal to μ and the only force acting are gravitational and frictional, then the magnitude of the frictional force acting on the disc is and its direction is .(write up or down) the inclined plane.
View SolutionA person pushes a block of mass 4 kg up a frictionless inclined plane 10 m long and that makes an angle of 30∘ with the horizontal . Then the work done is
View SolutionA force increases the speed of a 1.0 kg object from 4 m/s to 8 m/s. The work done by the force will be __________.
View SolutionA particle of mass 100 g is thrown vertically upwards with a speed of 5 m/s. The work done by the force of gravity during the time the particle goes up is
View SolutionA particle of mass 100g is thrown verically upward with a speed of 5m/s . The work done by the of gravity during the time the particle goes up is
View Solution

