The velocity of projectile at the point of projection is 2ˆi+3ˆjm/s. The velocity at a point where the projectile reaches is Solution in Telugu
−2ˆi+3ˆjm/s
2ˆi−3ˆjm/s
2ˆi+3ˆjm/s
−(2ˆi+3ˆj)m/s
The correct Answer is:B
Step by step video, text & image solution for The velocity of projectile at the point of projection is 2hati + 3hatj m//s. The velocity at a point where the projectile reaches is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-2 (Horizontal projectile motion)
14 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-2 (Circular motion)
7 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-2 (A boat in a river)
7 Videos
- MAGNETISMBOOK - AAKASH SERIESCHAPTER - MAGNETISMEXERCISE - PROBLEMS (LEVEL-II)
13 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
Similar Questions
A body is projected horizontally from a height of 78.4 m with a velocity 10ms−1. Its velocity after 3 seconds is - (g=10ms−2] (Take direction of projection on →i and vertically upward direction on →j)
View SolutionA point on the rim of a wheel 3 m in diameter has linear velocity of 18ms−1. The angular velocity of the wheel is
View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite point on the other bank. If the river is flowing at a speed of 3 kmph, he has to swim in the direction
View SolutionThe force between the two poles is reduced to 'x' newtons when their separation is increased n times. It is increased by 'y' newtons when their separation is made 1/nth of their original value. What is the relation between x and y?
View SolutionA particle is projected over a triangle from one end of a horizontal base and garzing the vertex falls on the other end of the base . If α and β the base angles and θ the angle of projection then show that tanθ=tanα+tanβ.
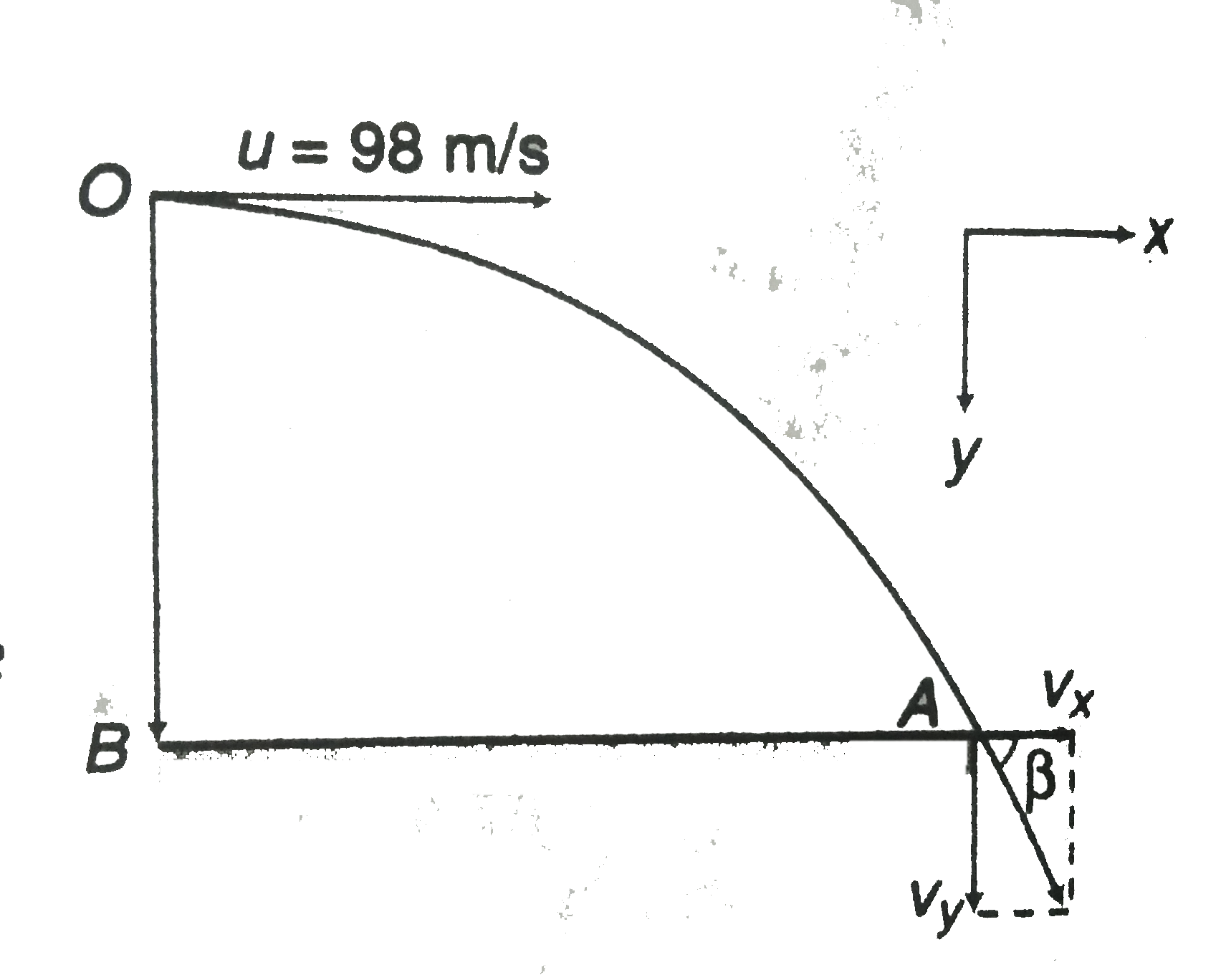
View SolutionA projectile is fired horizontally with velocity of 98 m/s from the top of a hill 490 m high. Find
(a) the time taken by the projectile to reach the ground,
(b) the distance of the point where the particle hits the ground from foot of the hill and
(c) the velocity with which the projectile hits the ground. (g=9.8m/s2)
 .
.
View SolutionThe velocity of a projectile at the initial point A is (2hat(i)+3hat(j))m//s .Its velocity (in m//s) at point B is
View SolutionThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s. Its velocity (in m/s) at point B is

View SolutionA projectile is thrown into air so as to have the maximum possible range equal to 200 . Taking projection point as origin, the coordinates of the point where the velocity of the projectile is minimum, are
View Solutionकिसी प्रक्षेप्य का प्रारंभिक बिंदु A पर वेग (2ˆi+3ˆj) मी/से है | प्रक्षेप्य का बिंदु B पर वेग है -
View SolutionA particle is projected at an angle of60∘ above the horizontal with a speed of 10m/s .When the projectile reaches highest point, change in velocity is
View SolutionThe velocity of projectile at the point of projection is 2hati + 3hatj m//s . The velocity at a point where the projectile reaches is
View SolutionAt highest point velocity of projectile is …... (θ = angle of projection).
View Solutionकिसी प्रक्षेप्य का प्रारंभिक बिन्दुA पर वेग (2ˆi+3ˆj)m/s है, तो इसका बिन्दु B पर वेग (m/s में) होगा
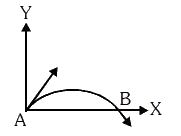
View SolutionThe velocity of projectile at the point of projection is 2ˆi+3ˆjm/s. The velocity at a point where the projectile reaches is
View SolutionA projectile is fired horizontally with velocity of 98 m/s from the top of a hill 490 m high. Find (a) the time taken by the projectile to reach the ground, (b) the distance of the point where the particle hits the ground from foot of the hill and (c) the velocity with which the projectile hits the ground. (g= 9.8 m//s^2) .
View Solution
