(a) State Kirchhoff's rules for an electirc network. Using Kirchhoff's rules, obtain the balance condition in terms of the resistances of four arms of Wheatstone bridge.
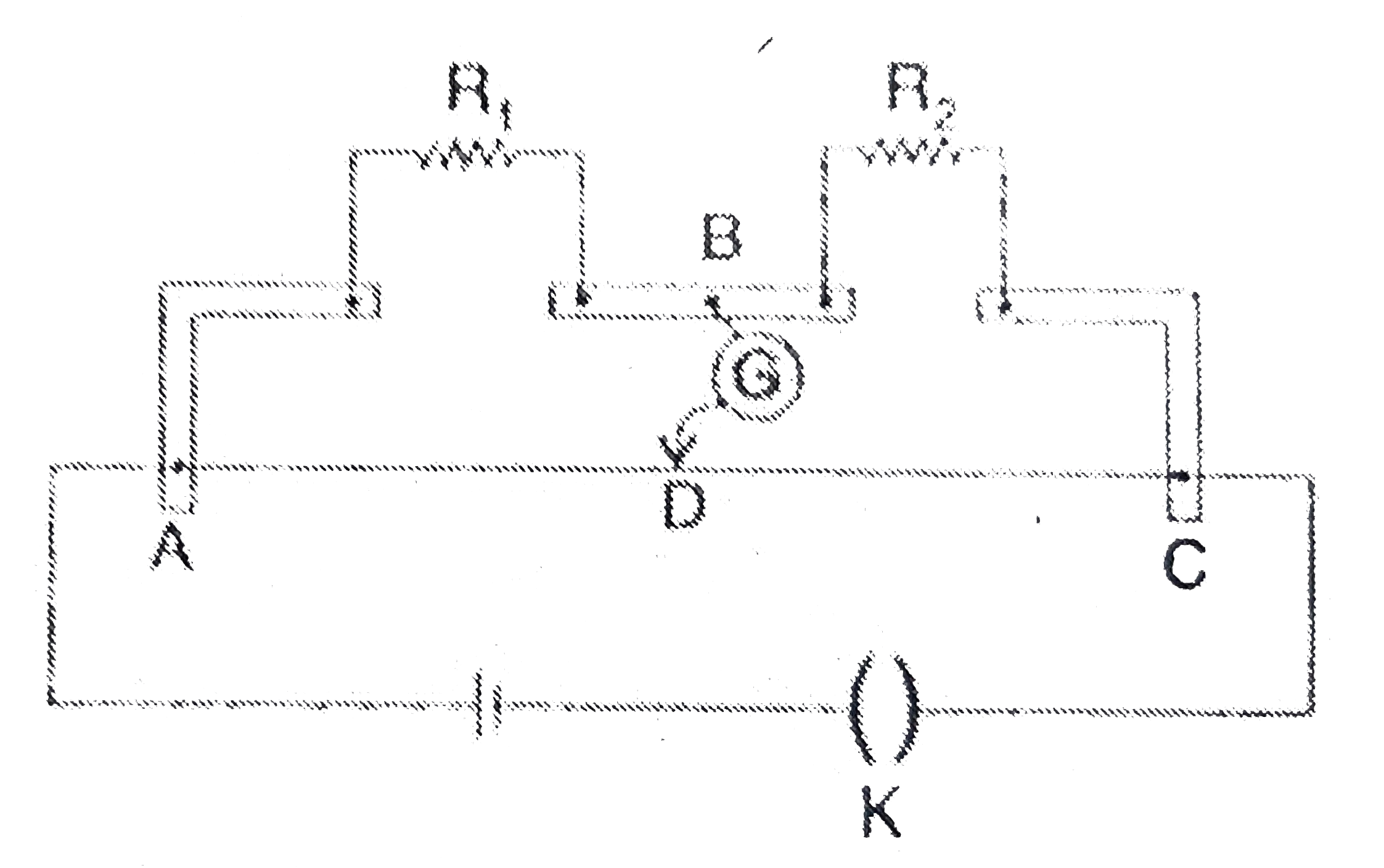
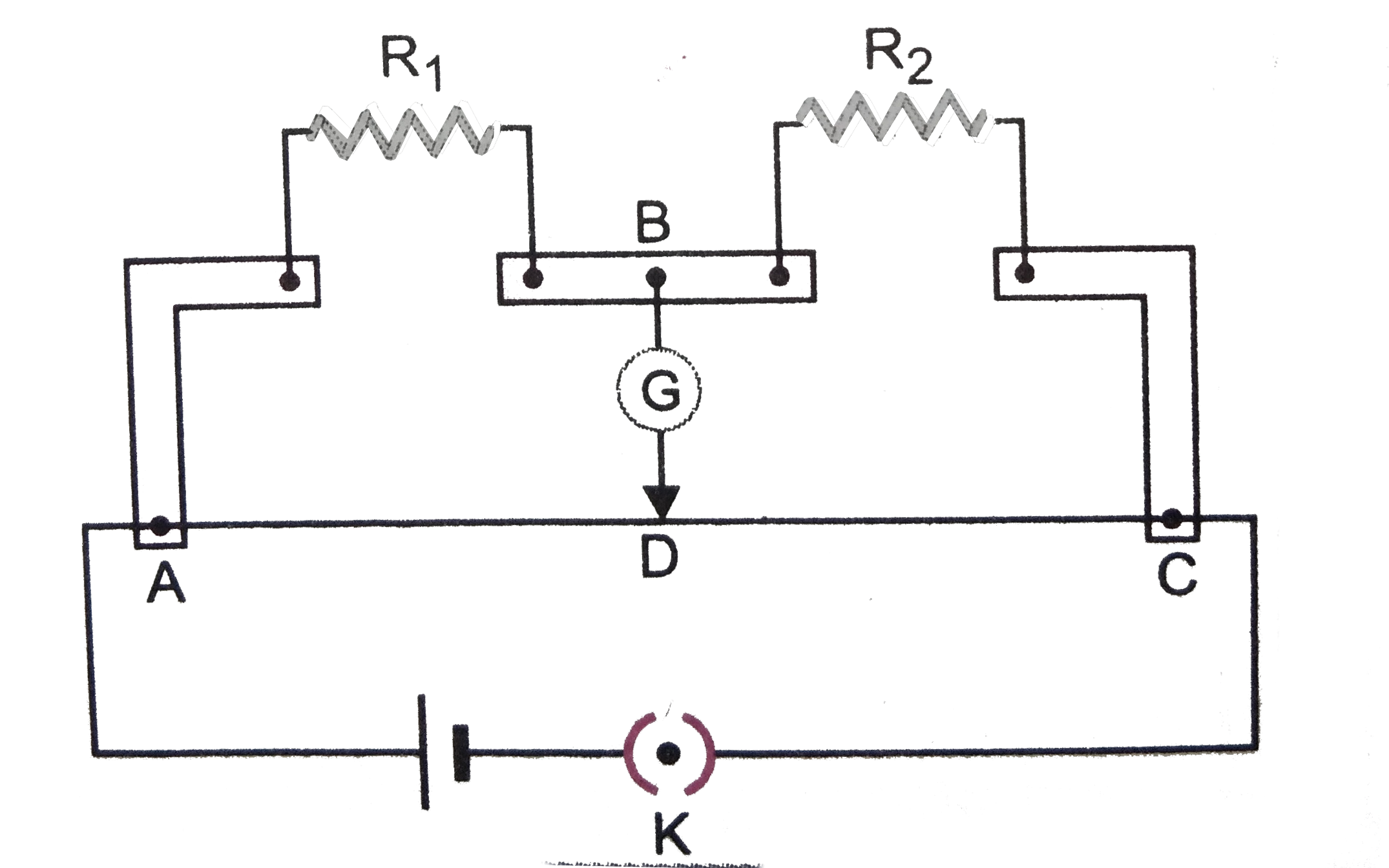
(b) In the meter bridge experimental set up, shown in the figure, the null point 'D' is obtained at a distance of 40 cm from end A of the meter bridge wire. If a resistance of 10Ω is connected in series with R1, null point is obtained at AD=60cm. Calculate the values of R1andR2.
(a) Kirchhoff's First Law - junction Rule. The algebraic sum of the currents meeting at a point in an electrical circuit is always zero.
Let the currents be I1,I2,I3andI4.
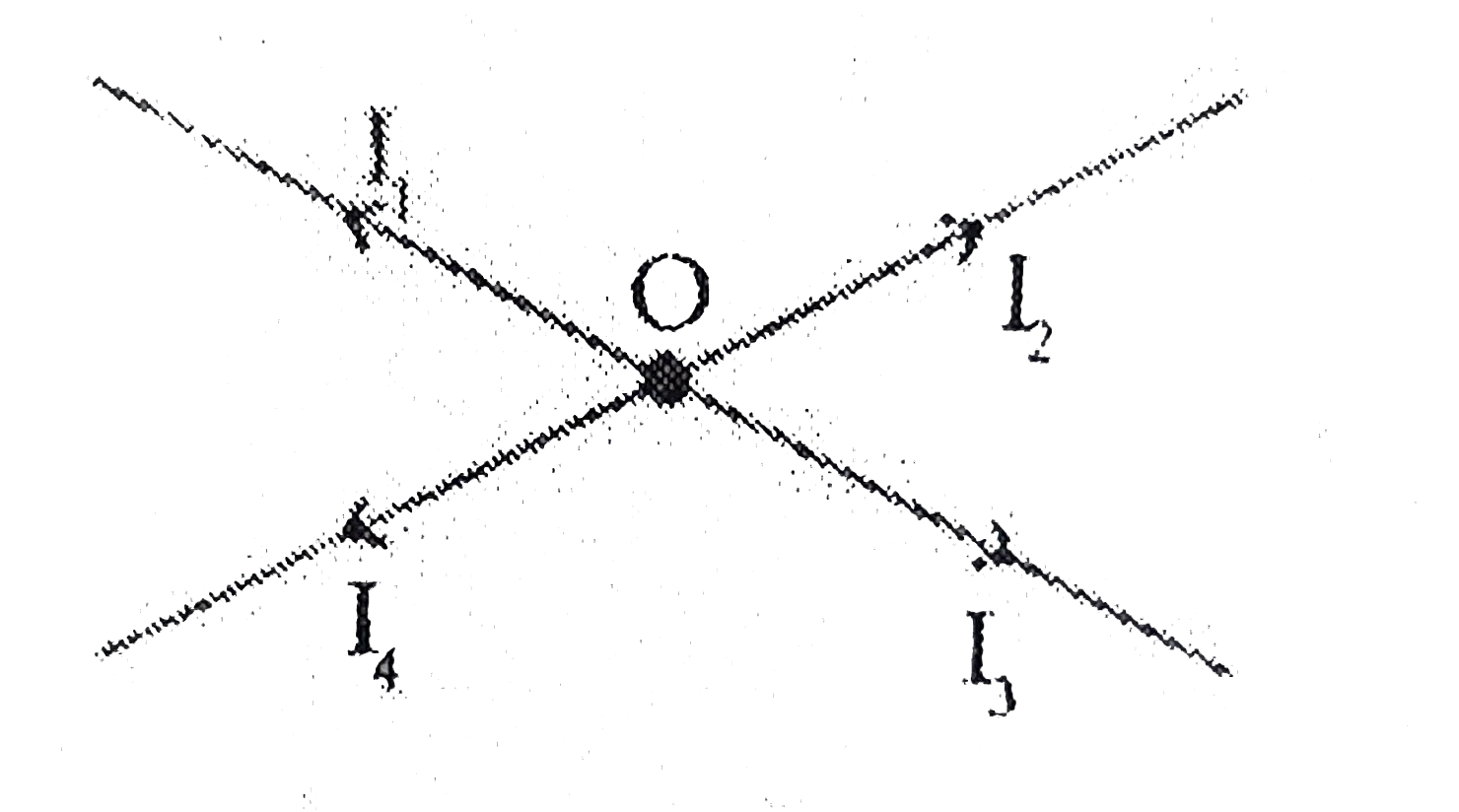
Convention : Current towards the junction -positive Current always from the junction-negative
I3+(−I1)+(−I2)+(−I4)=0
Kirchhoff's Second Law -Loop Rule.
In a closed loop, the algebric sum of the emf's is equal to the algebric sum of the products of the resistances and current flowing through them.
For closed part BACB,E1−E2−I1R1+I2R2−I3R3
For closed part CADC,E2=I3R3+I4R4+I5R5

Wheatstone Bridge : The Wheatstone Bridge is an arrangement of four resistance as shown in the following figure.
R1,R2,R3andR4 are the four resistances.
Galvanometer (a) has a current Ig flowing through it at balanced condition,
Ig=0
Applying junction rule at B,
∴I2=I4
Applying junction rule at D.
∴I1=I3
Applying loop rule to closed loop ADBA,
−I1R1+O+I2R2=O
∴I1I2=R2R1 ...(i)
Applying loop rule to closed loop CBDC,
I2R4+O−I1R3=O ...(ii).
From equations (i) and (ii)
R2R1=R4R3
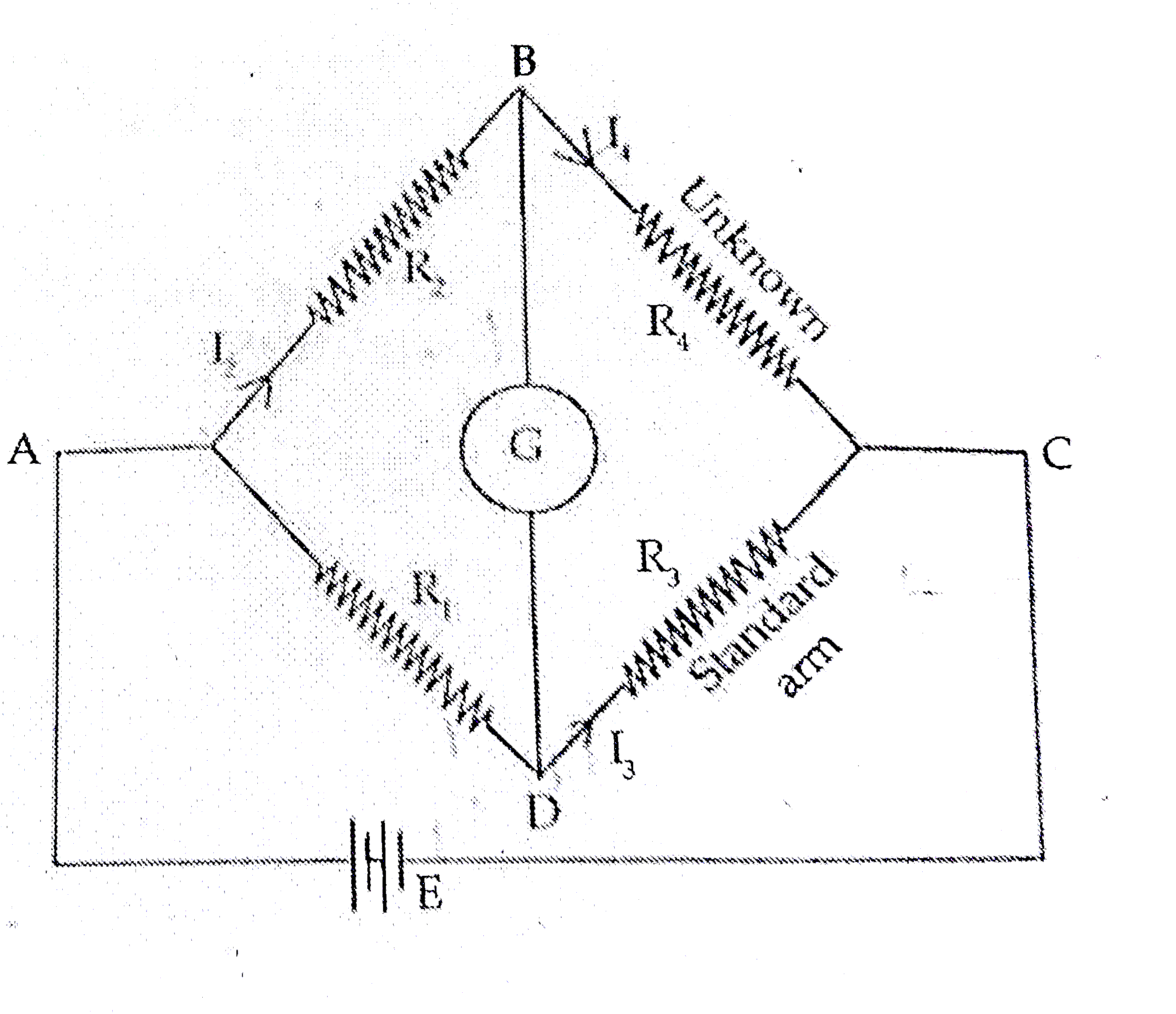
This is the required balanced condition of Wheatstone bridge.
(b) Considering both the situations and writing them in the form of equations.
Let R be the resistance per unit length of the potential meter wire,
R1R2=R'×40R'(100−40)=4060=23⇒R1+10R2=R'×60R'(100−60)=6040=32
R1R2=23 ...(i)
⇒R1+10R2=32 ...(ii)
Putting the value of R1, from equation (i) subtracting in equation (ii)
23+10R2=32
R2=12Ω
Recalling equation (i), again
R112=23
R1=8Ω.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET - II (Delhi Board)
8 Videos
- XII BOARDSBOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET - III (Delhi Board)
8 Videos
- XII BOARDSBOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET - III]
10 Videos
- SAMPLE PAPER 2019BOOK - XII BOARDS PREVIOUS YEARCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
(a) State Kirchhoff's rules for an electric network. Using Krichhoff's rules, obtain the balance condition in terms of the resistances of four arms of Wheatstone bridge,
(b) In the meterbridge experimental set up, shown in the Fig., the null point 'D' is obtained at a distance of 40 cm from end A of the meterbridge wire. If a resistance of 10Ω is connected in series with R1, null point is obtained at AD = 60 cm. Calculate the values of R1 and R2

View SolutionIn the meterbridge experimental set up, shown the null point D is obtained at a distance of 40cm from end A of the meter bridge wire if a resistance of 10Ω is connected in series with R1, null point is obtained at AD=30cm
View Solution
Knowledge Check
In a meter bridge, the null point is found at a distance of 40 cm from A. If now a resistance of 12 Ω is connected in parallel with S, the null point occurs at 60 cm from A. Determine the value of R.
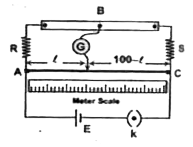 A15ΩB12ΩC10ΩD5Ω
A15ΩB12ΩC10ΩD5Ω
Use Kirchhoff's rules to obtain the balance condition in a Wheatstone bridge.
View SolutionIn meter bridge, the null points is found at a distance of 60.0 cm front end A. If now a resistance of 5Ω is connected in series with S, the null point occurs at 50 cm. Determine the values of R and S,
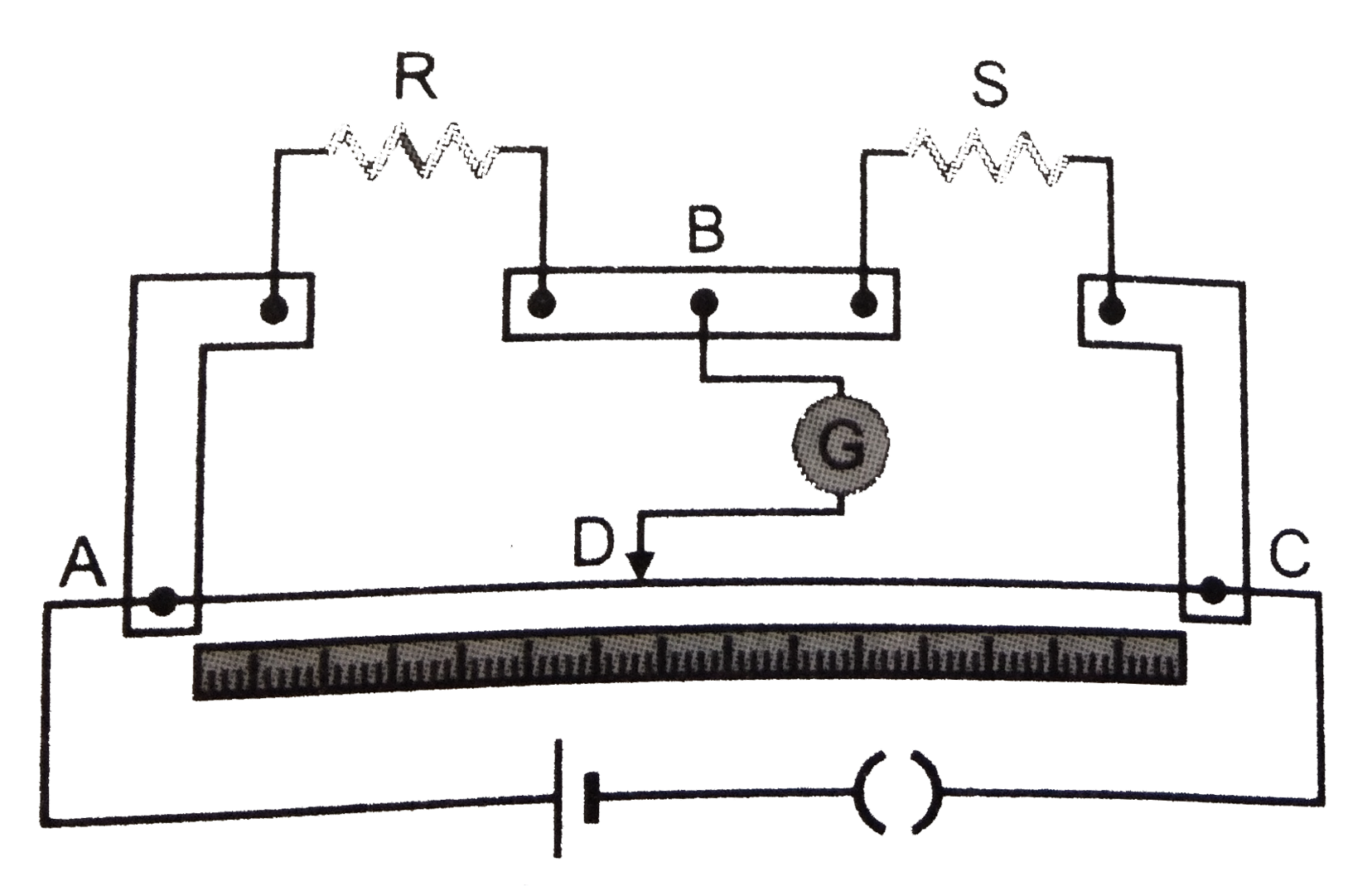
View SolutionState the Kirchhoff's rules used in electric networks. How are these rules justified ?
View SolutionCircuit diagram of metre bridge is shown in figure. The null point is found at a distance of 40cm from A. it now a resistance of 12Ω is connected in parallel with S, the null point occurs at 64cm.
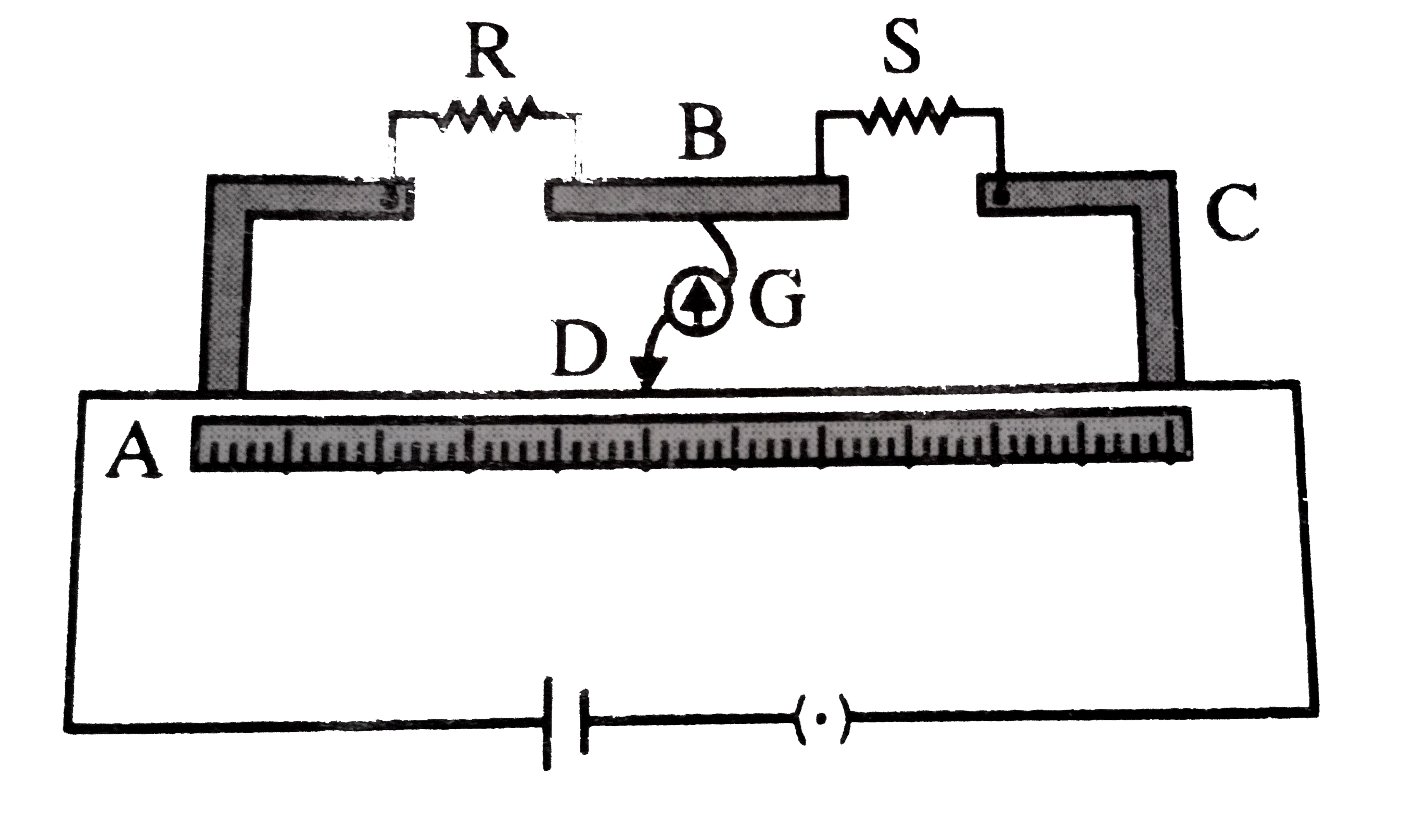
View SolutionIn a meter bridge, the null point is found at a distance of 40cm from A. If a resistance of 12Ω in connected in parallel with S the null point occurs at 50.0cm from A Determine the value of R and S

View SolutionUse Kirchhoff s rules to obtain conditions for the balance condition in a Wheatstone bridge.
View SolutionIn the experimental set up of meter bridge shown in the figure, the null point is obtained at a distance of 40 cm from A. If a 10Ω resistance that should be connected in parallel with (R1+10)Ω such that the null point shifts back to its initial position is :
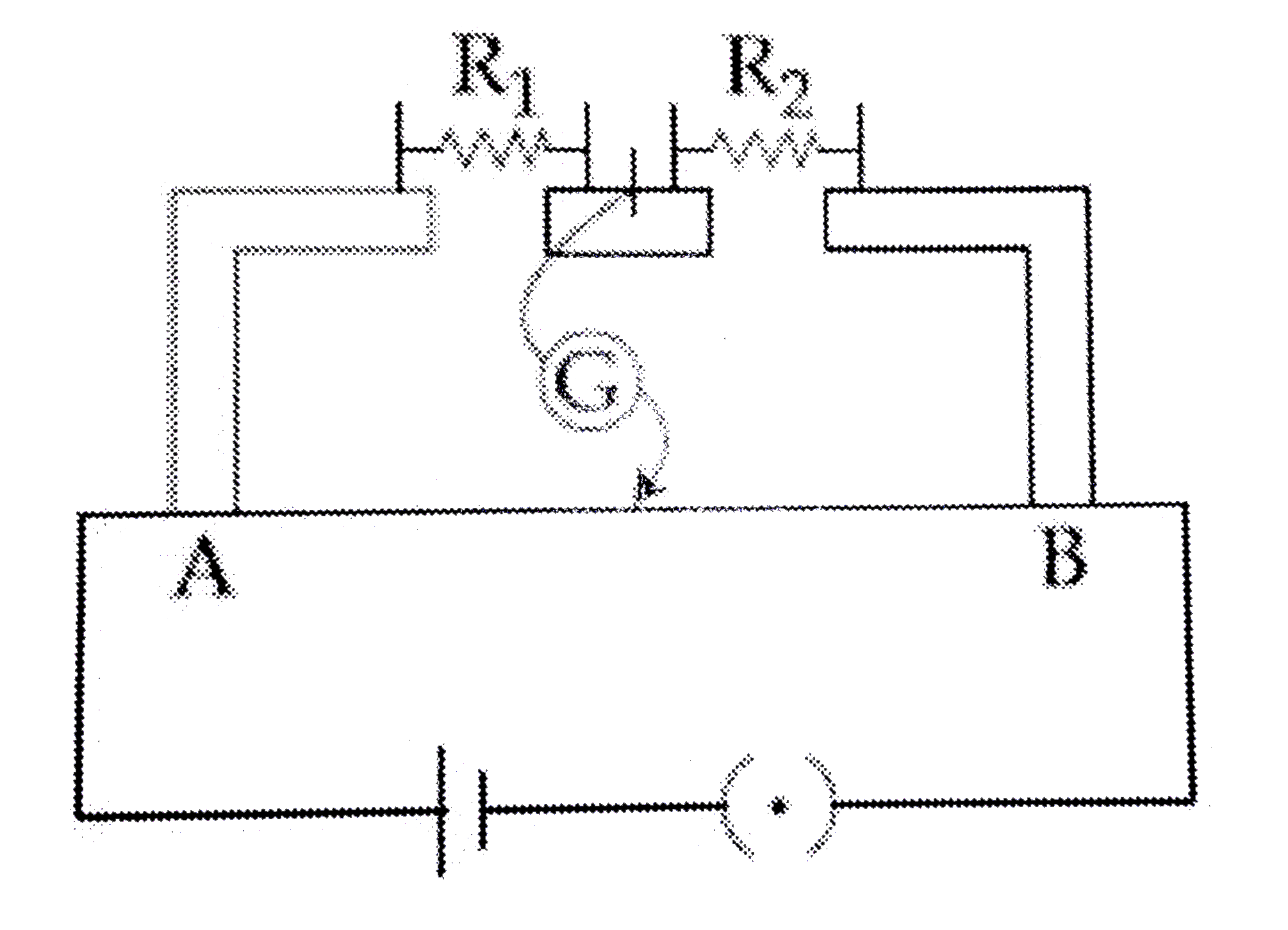
View Solution