A circular park is surrounded by a road 28 m wide. Find the area of the road if the circumference of the park is 880 m.
The correct Answer is:
Step by step video, text & image solution for A circular park is surrounded by a road 28 m wide. Find the area of the road if the circumference of the park is 880 m. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
- BOOK - VK GLOBAL PUBLICATIONCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - Proficiency Exercise (Long Answer Questions)19videos
AREAS RELATED TO CIRCLES
- BOOK - VK GLOBAL PUBLICATIONCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - Self -Assessment Test11videos
AREAS RELATED TO CIRCLES
- BOOK - VK GLOBAL PUBLICATIONCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - Proficiency Exercise (Short Answer Questions -I)13videos
AREAS RELATED TO CIRCLES
- BOOK - VK GLOBAL PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONS EXERCISE - SELF ASSESSMENT TEST10videos
ARITHMETIC PROGRESSIONS
Similar Questions
A circular park is surrounded by a road 21 m wide. If the radius of the park is 105 m, then find the area of the road.
 View Solution
View SolutionA square lawn is surrounded by a path 2.5 m wide. If the area of the path is 165 m2 , find the area of the lawn.
View SolutionA square lawn is surrounded by a path 2.5 m wide. If the area of the path is 165m2 find the area of the lawn.
View SolutionA circular pond is surrounded by a 2 m wide circular path. If outer circumference of circular path is 44 m, find the inner circumference of the circular path. Also find area of the path.
View SolutionA circular garden is surrounded by 3 m wide path. The circumference of the garden excluding the path is 88 m.
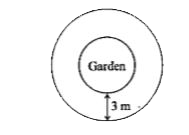
What is the area of the path surrounding the garden?View SolutionA rectangular park is 2034 m long and 1512 m wide. What is the area of the park?
View SolutionA square park is surrounded by a path of uniform width 2 metres all around it. The area of the path is 288 sq. metres. Find the perimeter of the park.
View SolutionA circular track runs around a circular park. If the difference between the circumference of the track and the park is 66 metres, find the width of the track.
View SolutionA circular track runs around a circular park . If the difference between the circumference of the track and the park is 66 cm , then find the width of the track .
View SolutionA circular road runs around a circular ground. If the difference between the circumference of the outer circle and the inner circle is 66 metres, the width of the road is :
(Take π=227)View Solution


