A radioactive sample decays with an average life of 20ms. A capacitor of capacitance 100μF is charged to some potential and then the plates are connected through a resistance R. What should be the value of R so that the ratio of the charge on the capacitor to the activity of the radioactive sample remains constant in time?
200Ω
400Ω
600Ω
800Ω
The correct Answer is:A
The activity of the sample at time t is given by A=A0e−λt where λ is the decay constant and A0 is the activity at time t = 0 when the capacitor plates are connected. The charge on the capacitor at time t is given by Q=Q0e−t/CR
where Q0 is the charge at t = 0 and C = 100 μF is the capacitance. Thus,
QA=Q0A0e−t/CRe−λt
It is independent of t if λ=1CR
or R=1λC=tavC=20×10−3s100×10−6F=200Ω
Topper's Solved these Questions
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS ( CATEGORY 1 : Single Option Correct Type )
1 Videos
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS
5 Videos
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE WORKOUT (CATEGORY 2: Single Option Correct Type )
15 Videos
- MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONBOOK - MTG-WBJEECHAPTER - MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONEXERCISE - WB-JEE Previous years questions
17 Videos
- OSCILLATIONS AND WAVESBOOK - MTG-WBJEECHAPTER - OSCILLATIONS AND WAVESEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3: ONE OR MORE THAN ONE OPTION CORRECT TYPE (2 MARKS))
2 Videos
Similar Questions
A radioactive sample has an average life of 30 ms and is decaying. A capacitor of capacitance 200 μF is first charged and later connected with resistor 'R'. If the ratio of charge on capacitor to the activity of radioactive sample is fixed with respect to time then the value of 'R' should be ______Ω.
View SolutionThe average life of a radioactive sample is 100 millisecond. A charged capacitor having capacitance 200 μF is connected across a resistance R. For what value of R, the ratio of charge on capacitor to the activity of the sample remains constant with time or independent of time.
View Solution
Knowledge Check
A charged capacitor of capacitance C is discharged through a resistance R. A radioactive sample decays with an average-life τ.Find the value of R for which the ratio of the electrostatic field energy stored in the capacitor to the activity of the radioactive sample remains constant in time.
AτCB2τCCτ2CD3τ2CFind the energy stored in a capacitor of capacitance 100μF when it is charged to a potential difference of 20 V.
A0.02JB0.04JC0.01JD0.05JA inductor of inductance L is decayed through a resistance R. A radioactive smaple decays with an average life T. The value of R for which the electric energy stored in the inductor to the activity of radioactive sample remains constant
ALTB2LTCL2TD(LT)2
A charged capacitor of capacity C is discharge through a resistance R. A radioactive sample decays with an average life J. If the ratio of electrostatic field energy stored in the capacitor to the activity of the radioactive sample remains constant with time then R=(xJ/C). where x is ________
View SolutionTwo capacitors of capacitance 10 μF and 20 μF are charged to potential 20 V and 10 V respectively. If they are connected to share charges, then loss of energy is
View SolutionA charged capacitor of capacitance 40μF is discharged through a 50Ω resistance. Determine the time constant of the circuit.
View SolutionA capacitor is charged to a certain potential and then allowed to discharge through a resistance R. The ratio of charge on the capacitor to current in the circuit
View SolutionA capacitor of capacitance 10μF is charged by connecting through a resistance of 20Omga and battery of 20 V. What is the energy supplied by the battery?
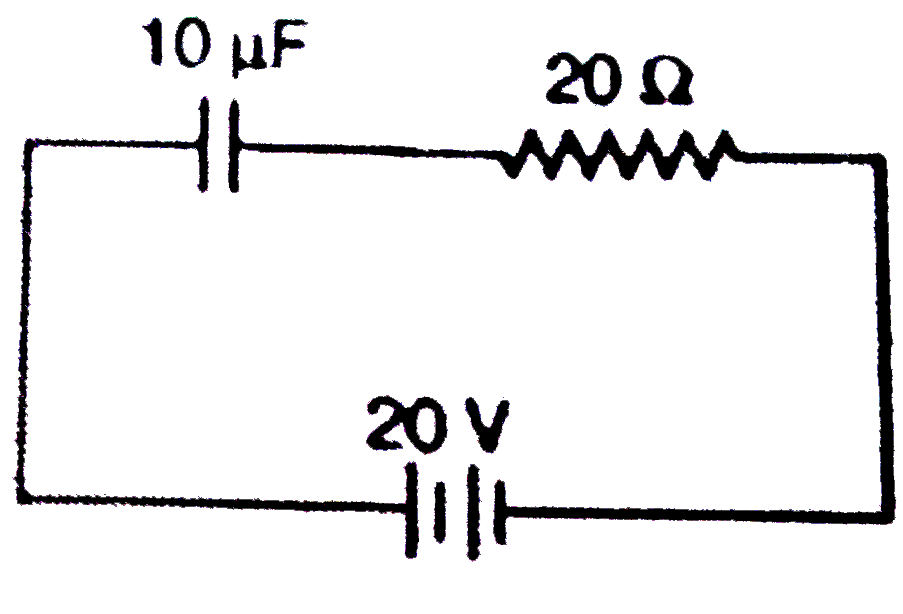
View Solution
