A radioactive sample has an average life of 30 ms and is decaying. A capacitor of capacitance 200 μF is first charged and later connected with resistor 'R'. If the ratio of charge on capacitor to the activity of radioactive sample is fixed with respect to time then the value of 'R' should be ______Ω.
The correct Answer is:150
To solve the problem, we need to find the value of the resistance R such that the ratio of the charge on the capacitor Q to the activity of the radioactive sample N remains constant over time.
1. Understanding the parameters:
- Average life of the radioactive sample, Tm=30ms=30×10−3s.
- Capacitance of the capacitor, C=200μF=200×10−6F.
2. Radioactive decay and capacitor discharge:
- The activity N of the radioactive sample at time t is given by:
N(t)=N0e−λt
where N0 is the initial activity and λ is the decay constant.
- The charge Q on the capacitor at time t when discharging through a resistor R is given by:
Q(t)=Q0e−tRC
where Q0 is the initial charge on the capacitor.
3. Setting up the ratio:
- We are given that the ratio QN is constant:
Q(t)N(t)=constant
- Substituting the expressions for Q(t) and N(t):
Q0e−tRCN0e−λt=constant
- This simplifies to:
Q0N0e(λ−1RC)t=constant
4. Condition for constant ratio:
- For the ratio to remain constant, the exponent must be zero:
λ−1RC=0
- Rearranging gives:
λ=1RC
5. Finding the decay constant λ:
- The decay constant λ is related to the average life Tm by:
λ=1Tm
- Substituting Tm=30×10−3s:
λ=130×10−3=100030≈33.33s−1
6. Substituting λ into the equation:
- From λ=1RC:
33.33=1R⋅200×10−6
- Rearranging gives:
R=133.33⋅200×10−6
7. Calculating R:
- Performing the calculation:
R=133.33⋅200×10−6=10.006666≈150Ω
Final Answer:
The value of R should be 150Ω.
---
- Average life of the radioactive sample, Tm=30ms=30×10−3s.
- Capacitance of the capacitor, C=200μF=200×10−6F.
- The activity N of the radioactive sample at time t is given by:
N(t)=N0e−λt
where N0 is the initial activity and λ is the decay constant.
Q(t)=Q0e−tRC
where Q0 is the initial charge on the capacitor.
- We are given that the ratio QN is constant:
Q(t)N(t)=constant
- Substituting the expressions for Q(t) and N(t):
Q0e−tRCN0e−λt=constant
- This simplifies to:
Q0N0e(λ−1RC)t=constant
- For the ratio to remain constant, the exponent must be zero:
λ−1RC=0
- Rearranging gives:
λ=1RC
- The decay constant λ is related to the average life Tm by:
λ=1Tm
- Substituting Tm=30×10−3s:
λ=130×10−3=100030≈33.33s−1
- From λ=1RC:
33.33=1R⋅200×10−6
- Rearranging gives:
R=133.33⋅200×10−6
- Performing the calculation:
R=133.33⋅200×10−6=10.006666≈150Ω
The value of R should be 150Ω.
Topper's Solved these Questions
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - PHYSICS (SECTION - A)
40 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - PHYSICS (SECTION - B)
20 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section B )
10 Videos
- JEE MAINS 2020BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2020EXERCISE - PHYSICS
246 Videos
- JEE MAINS 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -B)
10 Videos
Similar Questions
A radioactive sample decays with an average life of 20ms. A capacitor of capacitance 100μF is charged to some potential and then the plates are connected through a resistance R. What should be the value of R so that the ratio of the charge on the capacitor to the activity of the radioactive sample remains constant in time?
View SolutionThe average life of a radioactive sample is 100 millisecond. A charged capacitor having capacitance 200 μF is connected across a resistance R. For what value of R, the ratio of charge on capacitor to the activity of the sample remains constant with time or independent of time.
View Solution
Knowledge Check
A charged capacitor of capacitance C is discharging through a resistor of resistance R. At what time the charge on the capacitor is equal to one half of its initial value?
ARC2BRCloge2CRC loge2D1RCloge2A capacitor of capacitance 15μF has a charge 30μC and stored energy is W. If charge increased to 60μC the energy stored will be.
AWB4WC6WD1/2 WA capacitor of capacitance value 1μF is charged to 30 V and the battery is then disconnected. If it is connected across a 2μF capacotor, then the energy lost by the system is
A300μJB450μJC225μJD150μF
A capacitor of capacitance 100μF discharges through a resistor R. At the same time a radioactive substances decays with mean life 30 ms. If ratio of charge on capacitor and activity of substance does not change with time then find R.
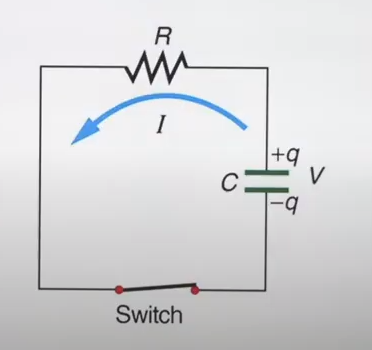
View SolutionA capacitor of capacitance as C is given a charge Q. At t=0,it is connected to an ideal battery of emf (ε)through a resistance R. Find the charge on the capacitor at time t.
View SolutionA charged capacitor of capacity C is discharge through a resistance R. A radioactive sample decays with an average life J. If the ratio of electrostatic field energy stored in the capacitor to the activity of the radioactive sample remains constant with time then R=(xJ/C). where x is ________
View SolutionA capacitor of capacitance as C is given a charge Q. At t=0,it is connected to an ideal battery of emf (ε)through a resistance R. Find the charge on the capacitor at time t.
View SolutionA capacitor of capacitance 200μF is connected to a battery through a resistance of 5kΩ at time t = 0. Charge stored in the capacitor in first second in larger than the charge stored in the next
View Solution
