When a capacitor is discharged through a resistor, which of the following is false?
The current in the capacitor is equal to the current in the resistor
The capacitor voltage decreases by half after a time equal to RC
The voltage across the capacitor is equal to the voltage across the resistor at any instant
The current in the circuit takes an infinite time to reach zero
The correct Answer is:D
To determine which statement is false when a capacitor is discharged through a resistor, we can analyze each statement based on the principles of capacitor discharge in an RC circuit.
1. Understanding Capacitor Discharge: When a capacitor discharges through a resistor, the stored energy in the capacitor is released, causing current to flow through the circuit. Electrons move from the negative plate of the capacitor to the positive plate, effectively reducing the charge on the capacitor.
Hint: Recall the basic principle of how a capacitor discharges in a circuit.
2. Analyzing the Statements:
- Statement 1: "The current in the capacitor is equal to the resistor."
- This statement is true. In a series circuit, the current flowing through the capacitor is the same as that flowing through the resistor.
Hint: Remember that in a series circuit, current remains constant throughout.
- Statement 2: "The capacitor voltage decreases by half after a time equal to RC."
- This statement is true. The voltage across a discharging capacitor decreases exponentially, and after a time equal to RC (the time constant), the voltage will indeed drop to approximately 36.8% of its initial value, which is often approximated as "half" for practical purposes.
Hint: Consider the exponential decay formula for capacitor discharge.
- Statement 3: "The voltage across the capacitor is equal to the voltage across the resistor."
- This statement is true. At any instant during the discharge, the voltage across the capacitor is equal to the voltage across the resistor due to Kirchhoff's voltage law.
Hint: Think about how voltage is distributed in a series circuit.
- Statement 4: "The current in the circuit takes an infinite time to reach zero."
- This statement is false. While the current decreases exponentially and approaches zero, it never actually takes an infinite time to reach zero; it gets very close to zero in a finite time.
Hint: Reflect on the nature of exponential decay and how it behaves over time.
3. Conclusion: After analyzing all the statements, we find that Statement 4 is the false statement regarding the discharge of a capacitor through a resistor.
Final Answer:
The false statement is: "The current in the circuit takes an infinite time to reach zero."
- Statement 1: "The current in the capacitor is equal to the resistor."
- This statement is true. In a series circuit, the current flowing through the capacitor is the same as that flowing through the resistor.
Hint: Remember that in a series circuit, current remains constant throughout.
- This statement is true. The voltage across a discharging capacitor decreases exponentially, and after a time equal to RC (the time constant), the voltage will indeed drop to approximately 36.8% of its initial value, which is often approximated as "half" for practical purposes.
- This statement is true. At any instant during the discharge, the voltage across the capacitor is equal to the voltage across the resistor due to Kirchhoff's voltage law.
- This statement is false. While the current decreases exponentially and approaches zero, it never actually takes an infinite time to reach zero; it gets very close to zero in a finite time.
The false statement is: "The current in the circuit takes an infinite time to reach zero."
Topper's Solved these Questions
- CIRCUITSBOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (More than One Correct Choice Type)
12 Videos
- CIRCUITSBOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Linked Comprehension)
11 Videos
- CIRCUITSBOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Problems
45 Videos
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (Integer )
4 Videos
- CIRCULAR MOTIONBOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - CHECK POINTS
6 Videos
Similar Questions
The figure-3.375 shows two circuits with a charged capacitor that is to be discharged through a resistor as shown in the figure. The ratio of initial charges on capacitors is given as q2/q1=2. If both switches are closed at time t=0, the charges become equal at 10−4ln2s. Find the resistance R.
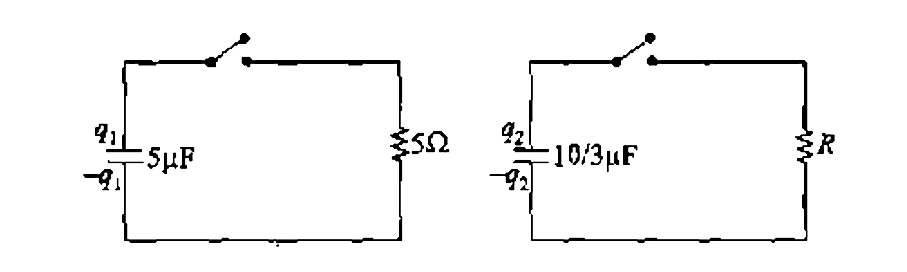
View SolutionA capacitor with an initial potential difference of 80.0 V is discharged through a resistor when a switch between them is closed at t=0. At t= 10.0s, the potential difference across the capacitor is 1.00V (a) What is time constant of the circuit? (b) What is the potential difference across the capacilor
View Solution
Knowledge Check
When a charged capacitor is discharged through a resistor, heat is produced in the resistor
In charging a capacitor, energy is stored in the capacitor.ABoth A and R falseBBoth A and R true and R is not correct reason for ACA is true and R is falseDBoth A and R are true and R is correct reason of A.When a capacitor discharges through a resistance R, the time constant is τ and the maximum current in the circuit is i0
AThe initial charge on the capacitor was i0τBThe initial charge on the capacitor was 12i0τCThe initial energy stored in the capacitor was i20τDThe initial energy stored in the capacitor was 12i20RτWhen a capacitor discharges through a resistance R, the time constant is τ and the maximum current in the circuit is i0. Then,
Athe initial charge on the capacitor was i0τBthe initial charge on the capacitor was 12i0τCthe initial energy stored in the capacitor was t20RτDthe initial energy stored in the capacitor was 12t20Rτ
A charged capacitor of 5×10−2 F capacity is discharged through a resistor R of 20Ω and a copper voltamete of internal resistance 30Ω connected in series. If 4.62×10−6 kg copper is deposited, calculated the heat generated ini the resistor R.
View SolutionA charged capacitor is discharged through a resistance. The time constant of the circuit is η. Then the value of time constant for the power dissipated through the resistance will be
View SolutionWhen the uncharged capacitor is charged through a resistor 'R' by a battery of emf 'epsilon’' as shown, the time taken to get charge that is 50% of the equilibrium value would be
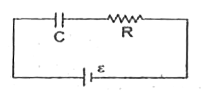
View Solutionfigure, shows a source of alternating voltage connected to a capacitor and a resistor. Which of the following phasor diagrams correctly describes the phase relationship between (IC) the current between the source and the capacitor and (IR) the current in the resistor?

View SolutionAssertion (A) : When a chraged condenser discharges through a resistor, the tiem taken for half the charge to be lost is always same, irrespective of the initial value of the charge.
Reason (R ) : The rate of decay of charge in a CR circuit is a linear function of time.
View Solution
