A certan substance has a dielectric constant of 5.6 and a dielectric strength of 18mV/m . IF it is used as the dielectric material in a parallel plate capacitor. What minium area should the plates of the capacitor have to obtain a capacitance of 3.9×10−2μF and to ensure that the capacitor will be able to withstand a potential difference of 4.0 kV?
To solve the problem, we need to find the minimum area of the plates of a parallel plate capacitor that can achieve a specified capacitance while also being able to withstand a certain voltage without breaking down. Here's the step-by-step solution:
Step 1: Understand the given values
- Dielectric constant (K) = 5.6
- Dielectric strength (E) = 18 mV/m = 18 × 10^-3 V/m
- Desired capacitance (C) = 3.9 × 10^-2 µF = 3.9 × 10^-8 F
- Voltage (V) = 4.0 kV = 4.0 × 10^3 V
Step 2: Relate capacitance to area
The capacitance C of a parallel plate capacitor with a dielectric is given by:
C=K⋅C0=K⋅A⋅ϵ0d
Where:
- C0 is the capacitance without the dielectric,
- A is the area of the plates,
- ϵ0 is the permittivity of free space (ϵ0≈8.85×10−12F/m),
- d is the separation between the plates.
Step 3: Relate voltage and electric field
The electric field E between the plates is given by:
E=Vd
From this, we can express d in terms of V and E:
d=VE
Step 4: Substitute d into the capacitance formula
Substituting d into the capacitance formula:
C=K⋅A⋅ϵ0VE=K⋅A⋅ϵ0⋅EV
Step 5: Solve for area A
Rearranging the formula to find A:
A=C⋅VK⋅ϵ0⋅E
Step 6: Plug in the values
Now we can substitute the known values into the equation:
A=(3.9×10−8F)⋅(4.0×103V)(5.6)⋅(8.85×10−12F/m)⋅(18×10−3V/m)
Step 7: Calculate A
Calculating the numerator:
3.9×10−8⋅4.0×103=1.56×10−4
Calculating the denominator:
5.6⋅8.85×10−12⋅18×10−3=9.35448×10−12
Now substituting these values:
A=1.56×10−49.35448×10−12≈0.0167m2
Final Answer
The minimum area A of the plates should be approximately 0.0167m2 or 167cm2.
---
- Dielectric constant (K) = 5.6
- Dielectric strength (E) = 18 mV/m = 18 × 10^-3 V/m
- Desired capacitance (C) = 3.9 × 10^-2 µF = 3.9 × 10^-8 F
- Voltage (V) = 4.0 kV = 4.0 × 10^3 V
The capacitance C of a parallel plate capacitor with a dielectric is given by:
C=K⋅C0=K⋅A⋅ϵ0d
Where:
- C0 is the capacitance without the dielectric,
- A is the area of the plates,
- ϵ0 is the permittivity of free space (ϵ0≈8.85×10−12F/m),
- d is the separation between the plates.
The electric field E between the plates is given by:
E=Vd
From this, we can express d in terms of V and E:
d=VE
Substituting d into the capacitance formula:
C=K⋅A⋅ϵ0VE=K⋅A⋅ϵ0⋅EV
Rearranging the formula to find A:
A=C⋅VK⋅ϵ0⋅E
Now we can substitute the known values into the equation:
A=(3.9×10−8F)⋅(4.0×103V)(5.6)⋅(8.85×10−12F/m)⋅(18×10−3V/m)
Calculating the numerator:
3.9×10−8⋅4.0×103=1.56×10−4
Calculating the denominator:
5.6⋅8.85×10−12⋅18×10−3=9.35448×10−12
Now substituting these values:
A=1.56×10−49.35448×10−12≈0.0167m2
The minimum area A of the plates should be approximately 0.0167m2 or 167cm2.
Topper's Solved these Questions
- CAPACITANCEBook:RESNICK AND HALLIDAYChapter:CAPACITANCEExercise:PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
Explore 23 Videos
- CAPACITANCEBook:RESNICK AND HALLIDAYChapter:CAPACITANCEExercise:PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
Explore 12 Videos
- CAPACITANCEBook:RESNICK AND HALLIDAYChapter:CAPACITANCEExercise:CHECKPOINTS
Explore 4 Videos
- ALL ABOUT ATOMS Book:RESNICK AND HALLIDAYChapter:ALL ABOUT ATOMS Exercise:CHECKPOINT
Explore 1 Video
- CENTER OF MASS Book:RESNICK AND HALLIDAYChapter:CENTER OF MASS Exercise:Practice Questions (Integer )
Explore 4 Videos
Similar Questions
Hard rubber has a dielectric constant of 2.8 and a dielectric strengh (maximum electric field) of 18×106 volt/meter. If it is used as the dielectric material filling the full space in a parallel plate capacitor. Minimum area may the plates of the capacitor have in order that the capacitance be 7.0×10−2μF is equal to πnm2. What should be the value of n if capacitor be able to withstand a potential difference of 4000 volts . (∈0=10−936π S.I unit)
View SolutionThe potential difference between the plates of a parallel plate capacitor is changing at the rate of 106Vs .If the capacitance is 2μF ,the displacement current in the dielectric of the capacitor will be
View Solution
Knowledge Check
A parallel -plate capacitor is to be designed, using a dielectric of dielectric constant 3, so as to have a dielectric strength of 10Vm−2. If the voltage rating of the capacitor is 12kV, the minimum area of each plate required to have a capacitance of 80pF is
A10.5×10−6m2B21.7×10−6m2C25.0×10−5m2D12.5×10−5m2If a dielectric slab of thickness 5 mm and dielectric constant K=6 is introduced between the plates of a parallel plate air capacitor, with plate separation of 8 mm, then its capacitance is
A1. decreasedB2. unaffectedC3. almost halvedD4. almost doubledA parallel plate capacitor with oil as a dielectric between the plates has a capacitance C. if the oil, with dielectric constannt (K=3), is removed, then the capacitance of the capacitor will be
A3CBC√3CC3D√3C
Two dielectric slabs of dielectric constants K1andK2 are filled in between the two plates, each of area A, of the parallel plate capacitor as shown in the figure. Find the net capacitance of the capacitor.
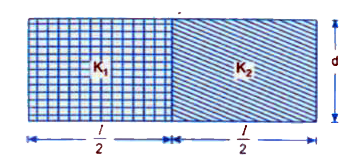
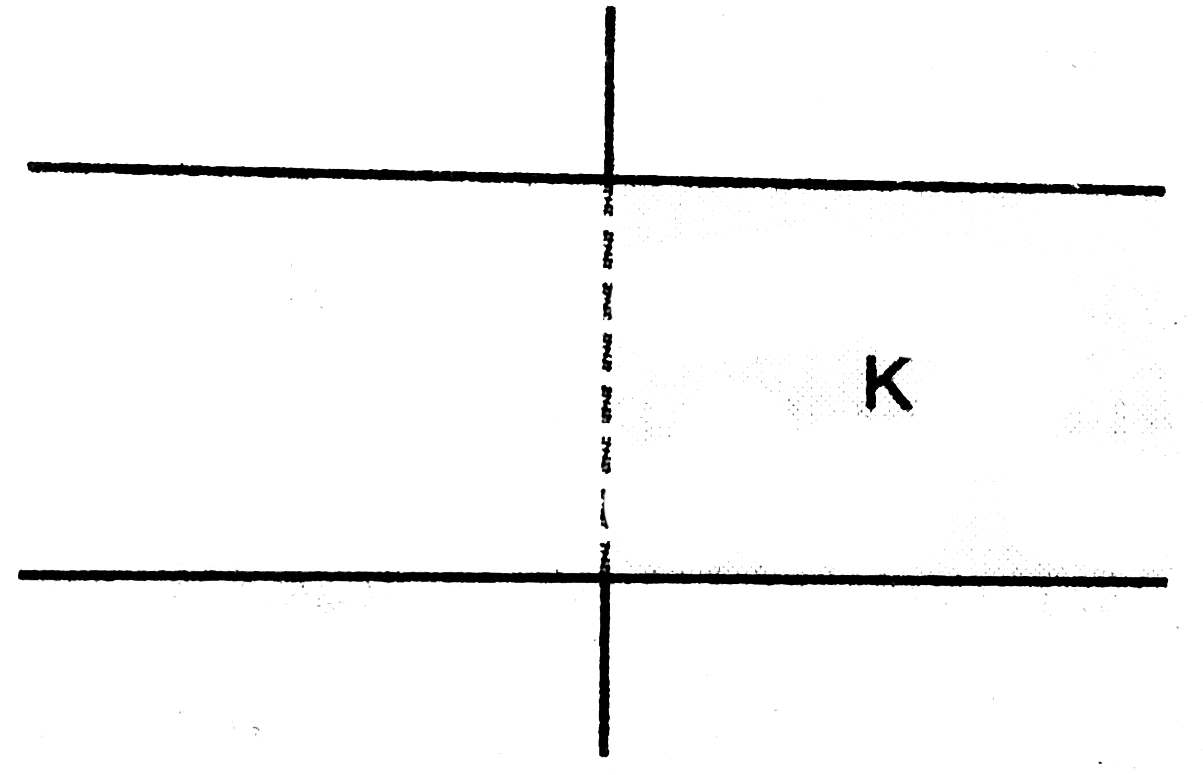
View SolutionAs shown in Fig, a dielectric material of dielectric constant K is inserted in half portion between plates of parallel plate capacitor. If its initial capacitance is C, what is the new capacitance ?
View SolutionA slab of material of dielectric constant K has the same area as the plates of a parallel plate capacitor, but has a thickness 3d/4. Find the ratio of the capacitance with dielectric inside it to its capacitance without the dielectric.
View SolutionThe area A of a parallel plate capacitor is divided into two equal, halves and filled with two media of dielectric constants K1andK2 respectively. The capacitance of the capacitor will be
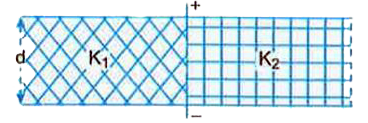
View SolutionA parallel plate capacitor with a sheet of paper (dielectric constant K) between the plates has a capacitance C. If the sheet is removed then capacitance of the capacitor becomes
View Solution