A dielectric material is to fill the space in a capacitor Initially, with only air in place, the capacitance is With the dielectric material in place, the capacitor should store at a maximum potential difference of 350.8V. (a) What dielectric constant is required? (b) Of the materials in Table 25-1, which material should be used?
To solve the problem step by step, we will follow these calculations:
Given Data:
- Initial capacitance (C₀) = 8.0 µF = 8.0 × 10⁻⁶ F
- Energy stored (U) = 3.2 µJ = 3.2 × 10⁻⁶ J
- Maximum potential difference (V) = 350.8 V
Step 1: Calculate the new capacitance (C) using the energy formula.
The energy stored in a capacitor is given by the formula:
U=12CV2
Rearranging the formula to find C:
C=2UV2
Substituting the known values:
C=2×(3.2×10−6)(350.8)2
Step 2: Calculate the value of C.
Calculating V2:
V2=(350.8)2=123168.64
Now substituting this back into the equation for C:
C=2×(3.2×10−6)123168.64
C=6.4×10−6123168.64
C≈5.2×10−5F=52μF
Step 3: Relate the new capacitance (C) to the dielectric constant (K).
The capacitance with the dielectric is given by:
C=KC₀
Where:
- C₀ is the capacitance without the dielectric (8.0 µF).
- K is the dielectric constant.
Rearranging the equation gives:
K=CC₀
Step 4: Substitute the values to find K.
Substituting the values we found:
K=52μF8.0μF
K=528=6.5
Final Answers:
(a) The dielectric constant required is 6.5.
(b) To determine which material to use, you would refer to Table 25-1, which lists dielectric constants for various materials. The material with a dielectric constant closest to 6.5 should be selected.
- Initial capacitance (C₀) = 8.0 µF = 8.0 × 10⁻⁶ F
- Energy stored (U) = 3.2 µJ = 3.2 × 10⁻⁶ J
- Maximum potential difference (V) = 350.8 V
The energy stored in a capacitor is given by the formula:
U=12CV2
C=2UV2
C=2×(3.2×10−6)(350.8)2
Calculating V2:
V2=(350.8)2=123168.64
C=2×(3.2×10−6)123168.64
C=6.4×10−6123168.64
C≈5.2×10−5F=52μF
The capacitance with the dielectric is given by:
C=KC₀
- C₀ is the capacitance without the dielectric (8.0 µF).
- K is the dielectric constant.
K=CC₀
Substituting the values we found:
K=52μF8.0μF
K=528=6.5
(a) The dielectric constant required is 6.5.
Topper's Solved these Questions
- CAPACITANCEBook:RESNICK AND HALLIDAYChapter:CAPACITANCEExercise:PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
Explore 23 Videos
- CAPACITANCEBook:RESNICK AND HALLIDAYChapter:CAPACITANCEExercise:PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
Explore 12 Videos
- CAPACITANCEBook:RESNICK AND HALLIDAYChapter:CAPACITANCEExercise:CHECKPOINTS
Explore 4 Videos
- ALL ABOUT ATOMS Book:RESNICK AND HALLIDAYChapter:ALL ABOUT ATOMS Exercise:CHECKPOINT
Explore 1 Video
- CENTER OF MASS Book:RESNICK AND HALLIDAYChapter:CENTER OF MASS Exercise:Practice Questions (Integer )
Explore 4 Videos
Similar Questions
A parallel plate capacitor has a capacitance of 2μF. A slab of dielectric constant 5 is inserted between the plates and the capacitor is charged to 100V and then isolated . (a) What is the new potential diff., if the dielectric slab is removed ? (b) How much work is required to remove teh dielectric slab ?
View SolutionA dielectric (k=3.2) is placed in a capacitor of distance 1m and area 2m2. The width of dielectric is 0.5m. If net capacitance is xε0
View Solution
Knowledge Check
The capacitance of a parallel plate capacitor is 16μF. When a glass slab is placed between the plates, the potential difference reduces to 1/8th of the original value. What is dielectric constant of glass ?
A4B8C16D32A capacitor having capacitance 1μF with air is filled with two dielectrics as shown. How many times capacitance will increase ?
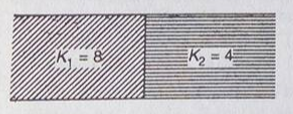 A12B6C8/3D3
A12B6C8/3D3A capacitor having capacitance 1μF with air is filled with two dielectrics as shown. How many times capacitance will increase ?
 A12B6C8/3D3
A12B6C8/3D3
In figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?

View SolutionTwo identical parallel plate air capacitors are connected in series to a battery of emf V. If one of the capacitor is completely filled with dielectric material of constant K, then potential difference of the other capacitor will become
View SolutionIn a capacitor of capacitance 20μF the distance the plates is 5mm. If a dielectric slab of width 2 dielectric constant 3 is inserted between the plates, new capacitor will be
View SolutionA paralle plate air capacitor has capacitance C. Now half of the space is fillel with a dielectric material with dielectric constant K as shown in figure . The new capacitance is C . Then

View SolutionA 6×10−4 F parallel plate air capacitor is connected to a 500 V battery. When air is replaced by another dielectric material, 7.5×10−4C charge flows into the capacitor. The value of the dielectric constant of the material is
View Solution