Two point masses m and M are separated by a distance d. If the separation d remains fixed and the masses are increased to the values 3m and 3M, respectively, how does the gravitational force between them change ?
The force will be one-third as great.
The force will be one-ninth as great.
The force will be three times as great.
The force will be nine times as great.
The correct Answer is:D
To solve the problem, we will use Newton's law of universal gravitation, which states that the gravitational force F between two point masses m and M separated by a distance d is given by the formula:
F=GmMd2
where G is the gravitational constant.
Step 1: Write the initial gravitational force
Initially, the gravitational force F1 between the masses m and M is:
F1=GmMd2
Step 2: Determine the new masses
The problem states that the masses are increased to 3m and 3M.
Step 3: Write the new gravitational force
The new gravitational force F2 between the masses 3m and 3M is:
F2=G(3m)(3M)d2
Step 4: Simplify the new gravitational force
Now, simplify F2:
F2=G9mMd2
Step 5: Compare the new force with the initial force
Now, we can compare F2 with F1:
F2=9(GmMd2)=9F1
Conclusion
Thus, the gravitational force between the two masses increases by a factor of 9 when both masses are increased to three times their original values while keeping the distance d constant. Therefore, the gravitational force becomes 9 times greater.
Final Answer
The gravitational force between the two masses becomes 9 times greater.
---
Initially, the gravitational force F1 between the masses m and M is:
The problem states that the masses are increased to 3m and 3M.
The new gravitational force F2 between the masses 3m and 3M is:
Now, simplify F2:
Now, we can compare F2 with F1:
Thus, the gravitational force between the two masses increases by a factor of 9 when both masses are increased to three times their original values while keeping the distance d constant. Therefore, the gravitational force becomes 9 times greater.
The gravitational force between the two masses becomes 9 times greater.
Topper's Solved these Questions
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)
5 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Linked Comprehension)
10 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PROBLEMS
52 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PRACTICE QUESTIONS (Integer Type )
5 Videos
- FORCE AND MOTION-IIBOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION-IIEXERCISE - PRACTICE QUESTIONS (Integer Type)
1 Videos
Similar Questions
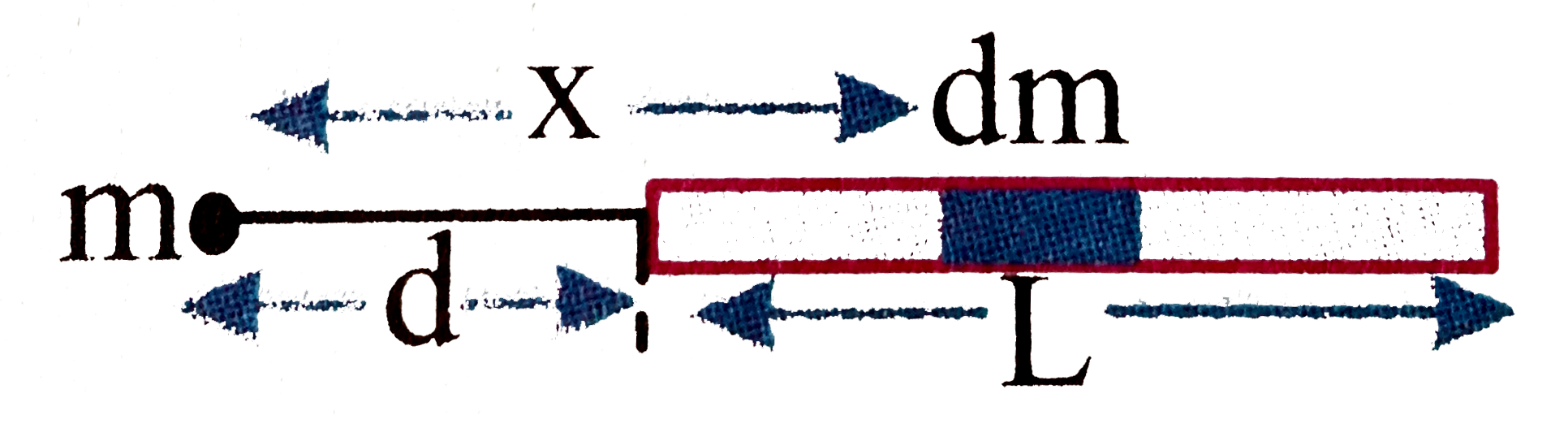
A particle of mass m is situated at a distance d from one end of a rod of mass M and length L as shown in diagram. Find the magnitude of the gravitational force between them
View SolutionTwo point particles of mass m and 2m are initially separated by a distance 4a. They are then released to become free to move. Find the velocities of both the particles when the distance between them reduces to a.
View Solution
Knowledge Check
Two point masses m and M are separated by a distance L . The distance of the centre of mass of the system from m is
AL(m/M)BL(M/m)CL(Mm+M)DL(mm+M)Two point masses m and M are separated bya distance L . The distance of the centre of mass of the system from m is
AL(m/M)BL(M/m)CL(Mm+M)DL(mm+M)Two bodies of masses m1 and m2 are separated by a distance R. The distance of the centre of mass of the bodies from the mass m1 is
Am2Rm1+m2Bm1Rm1+m2Cm1m2m1+m2RDm1+m2m1R
Two bodies of masses m1 and m2 are separated by a distance R. The distance of the centre of mass of the bodies from the mass m1 is
View SolutionTwo point masses m and 4 m are seperated by a distance d on a line . A third point mass m0 is to be placed at a point on the line such that the net gravitational force on it zero .

The distance of that point from the m mass is
View SolutionTwo convex lesses of power 2 D and 5 D are separated by a distance 13m m The power of the optical system formed is
View SolutionTwo identical spheres each of mass M and radius R are separated by a distance 3R. The force of attraction between them is proportional to
View SolutionThe gravitational force between two particles of masses m1 and m2 separeted by the same distance, then the gravitational force between them will be
View Solution
