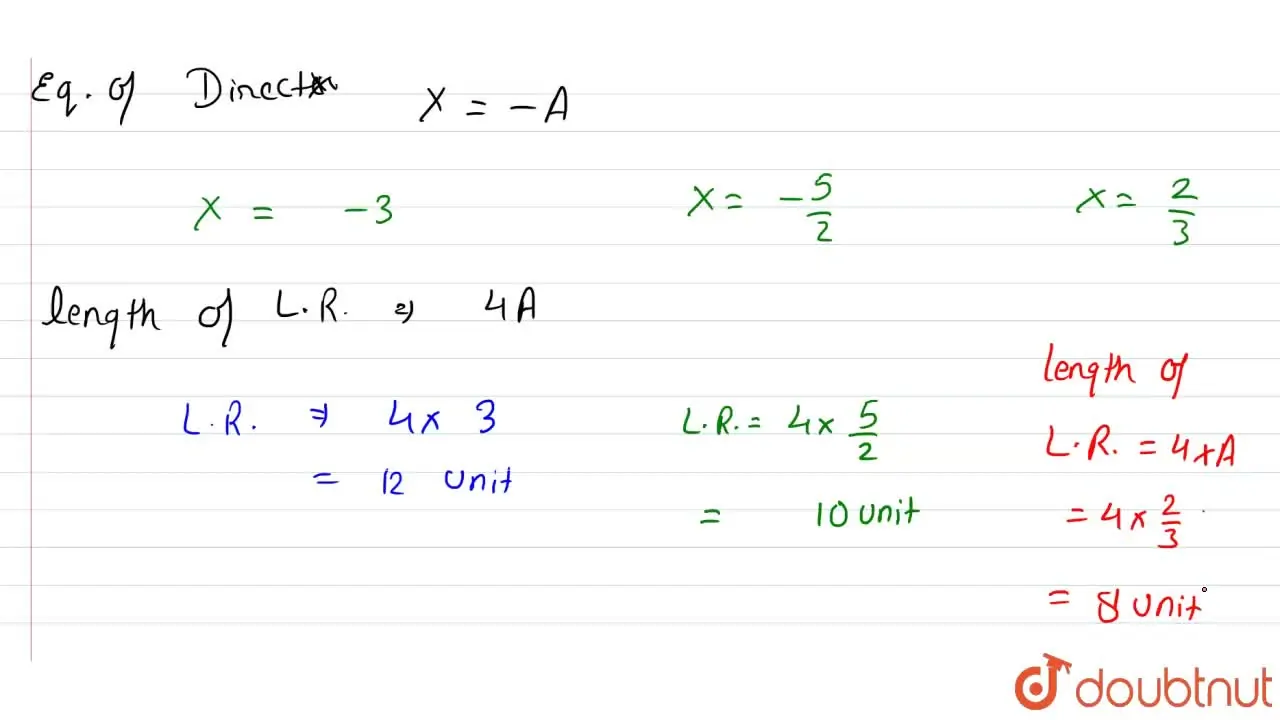Find the coordinates of the focus and the vertex, the equations of the directrix and the axis, and length of the latus rectum of the parabola:
(i) y2=12x (ii) y2=10x (iii)3y2=8x
(i)F(3,0),O(0,0),x+3=0,y=0,12units
(ii)F(52,0),O(0,0),2x+5=0,y=0,10units
(iii)F(23,0),O(0,0),3x+2=0,y=0,83units
Related Playlists
Similar Questions
Find the coordinates of the focus and the vertex, the equations of the directrix and the axis, and length of the latus rectum of the parabola:
(i) y2=−8x (ii)y2=−6x (iii)5y2=−16xView SolutionFind the coordinates of the focus and the vertex, the equations of the directix and the axis, and the lendth of the latus rectum of the parabola y2=−12x
View SolutionFind the coordinates of the focus and the vertex, the equations of the directix and the axis, and the lendth of the latus rectum of the parabola y2=8x
View SolutionFind the coordinates of the focus and the vertex, the equations of the directix and the axis, and the lendth of the latus rectum of the parabola x2=−16Y
View SolutionFind the coordinates of the focus and the vertex, the equations of the directrix and the axis, and length of the latus rectum of the parabola: (i)x2=16y (ii)x2=10y (iii)3x2=8y
View SolutionFind the coordinates of the focus and the vertex, the equations of the directix and the axis, and the lendth of the latus rectum of the parabola x2=6y
View SolutionFind the coordinates of the focus and the vertex, the equations of the directrix and the axis, and length of the latus rectum of the parabola:
(i)x2=−8y (ii)x2=−18y (iii)3x2=−16yView SolutionFind the coordinates of the focus, axis of the parabola, the equation of the directrix and the length of the latus rectum.x2=6y
View SolutionThe length of the latus rectum of the parabola y2=8x is
View SolutionFind the coordinates of the focus, axis of the parabola, the equation of the directrix and the length of the latus rectum.y2=−8x
View Solution