Prove that the perimeter of a triangle is greater than the sum of its three medians.
Let AD, BE and CF be the three medians of a ΔABC.
We know that the sum of any two sides of a triangle is greater than twice the median drawn to the third side.
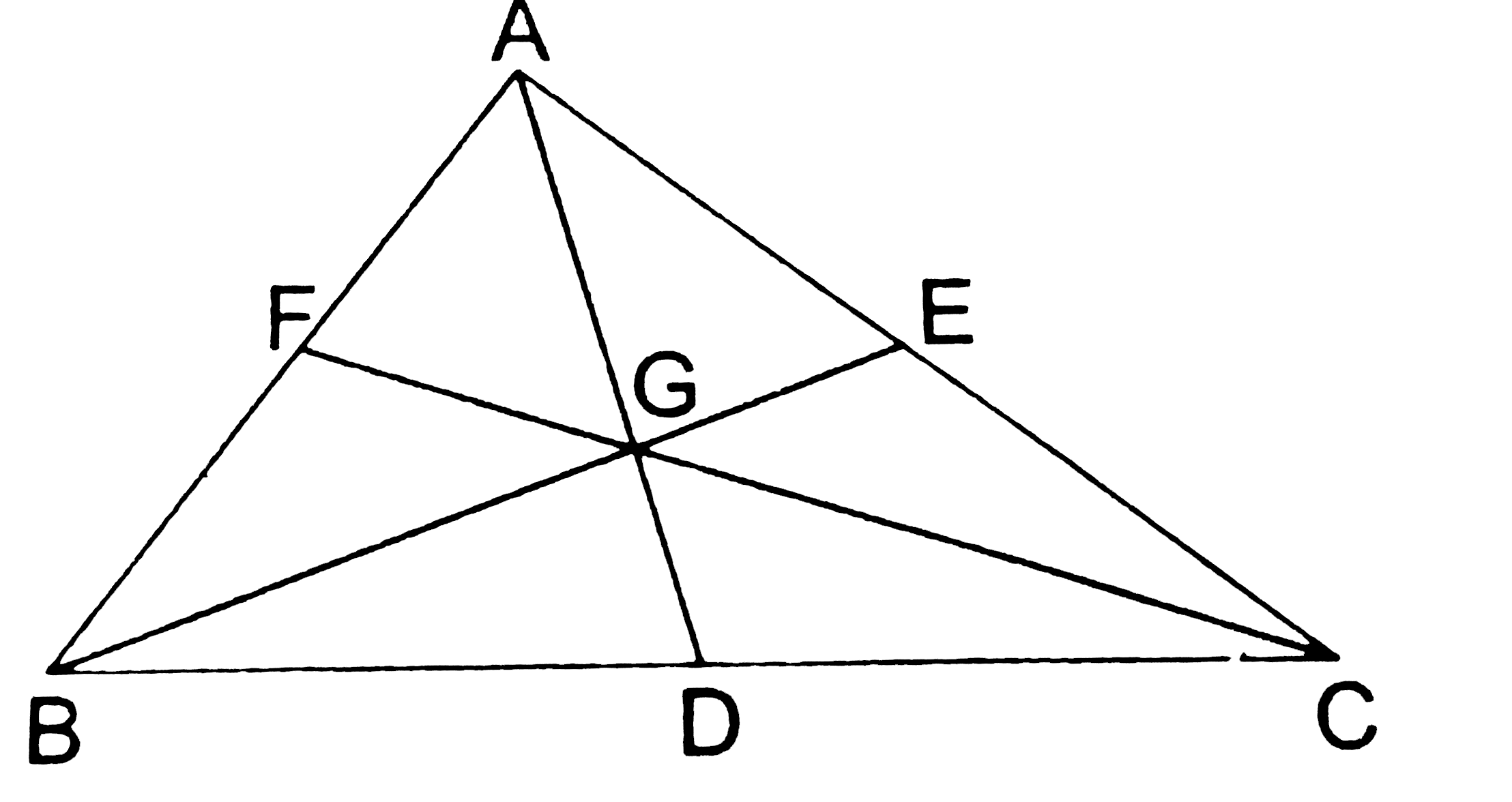
∴ AB+AC>2AD, ...(i)
AB+BC>2BE ...(ii)
and BC+AC>2CF. ...(iii)
Adding, the corresponding sides of (i), (ii) and (iii), we get
2(AB+BC+AC)>2(AD+BE+CF)
∴ (AB+BC+AC)>(AD+BE+CF).
Hence, the perimeter of a triangle is greater than the sum of its three medians.
Topper's Solved these Questions
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Exercise 9A
27 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Exercise 9B
16 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - MULTIPLE CHOICE QUESTION(MCQ)
37 Videos
- COORDINATE GEOMETRYBOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)
22 Videos
Similar Questions
Knowledge Check
Consider the following statements in respect of any triangle
I. The three medians of a triangle divide it into six triangles of equal area.
II. The perimeter of a triangle is greater than the sum of the lengths of its three medians.
Which of the statements given above is/are correct ?AI onlyBII onlyCBoth I and IIDNeither I nor II
Prove that the perimeter of any triangle is greater than the sum of three altitudes.
View SolutionConsider the following statements :
1. The perimeter of a triangle is greater than the sum of its three medians.
2. In any triangle ABC, if D is any point on BC, then AB + BC + CA > 2AD.
Which of the above statements is/are correct?
View SolutionProve that the sum of three altitudes drawn from the vertices to opposite sides of a triangle is less than the sum of three sides.
or
Prove that the perimeter of a triangle is greater than the sum of three altitudes drawn from the vertices to opposite of a triangle.
View SolutionProve that the sum of any two sides of a triangle is greater than two times the median of third side.
View SolutionProve that sum of any two medians of a triangle is greater than the third median.
View SolutionWhich of the following statements are true (T) and which are false (F)? Sum of the three sides of a triangle is less than the sum of its three altitudes. Sum of any two sides of a triangle is greater than twice the median drawn to the third side. Sum of any two sides of a triangle is greater than the third side. Difference of any two sides of a triangle is equal to the third side. If two angles of a triangle are unequal, then the greater angle has the larger side opposite to it. Of all the line segments that can be drawn from a point to a line not containing it, the perpendicular line segment is the shortest one.
View SolutionProve that sum of any two sides of a triangle is greater than twice the median with respect to the third side.
View Solution
