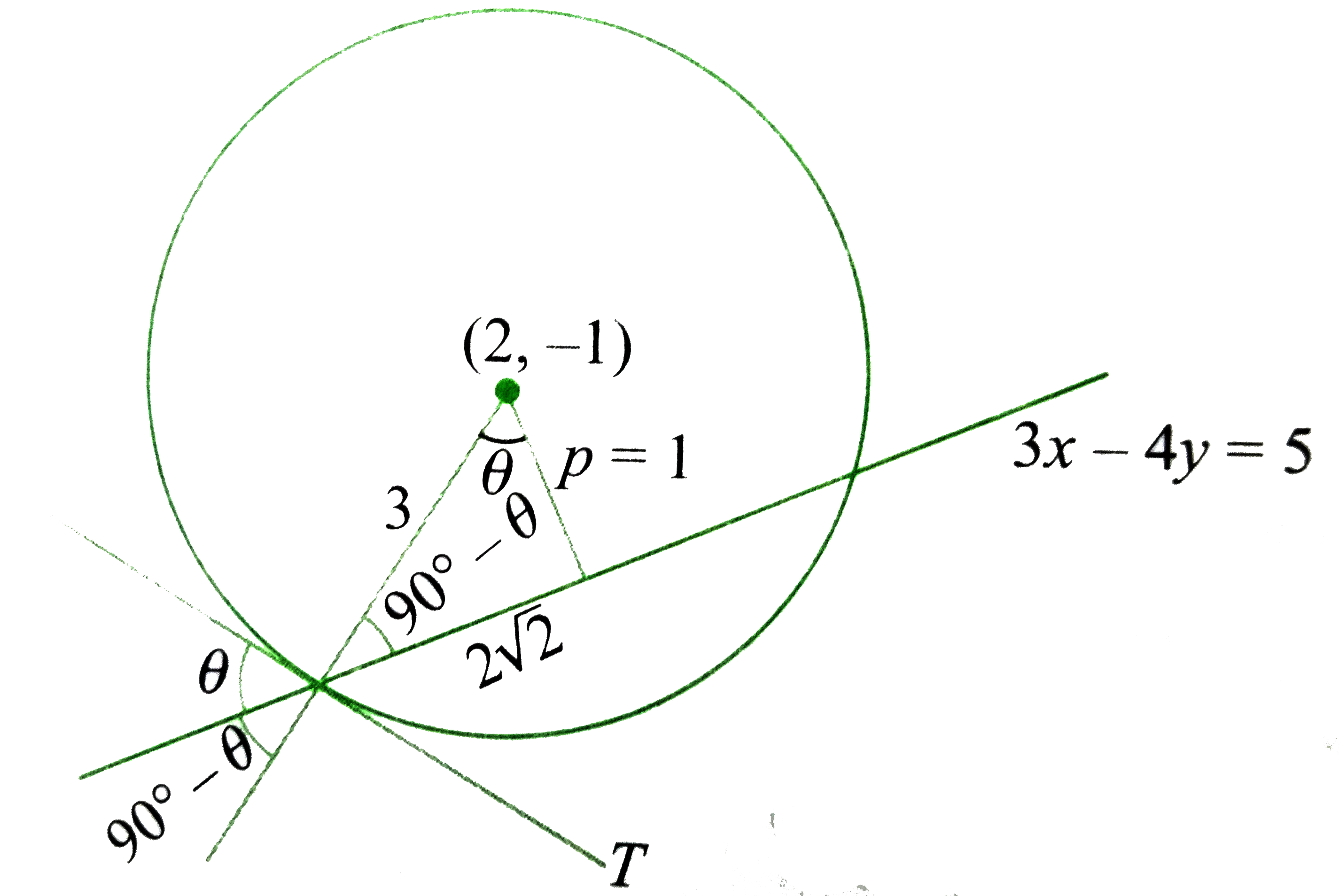
The acute angle between the line 3x-4y=5 and the circle x^2+y^2-4x+2y-

The acute angle between the curve $y = x e^{- x}$ and the straight line 3x + 2y = 0 is
A $\frac{π}{2} - {tan}^{- 1} \frac{3}{2}$
B $\frac{3 π}{4} - {tan}^{- 1} \frac{3}{2}$
C $π - {tan}^{- 1} \frac{3}{2}$
D ${tan}^{- 1} \frac{3}{2}$
The acute angle between the line represented by $2 x^{2} + x y - y^{2} + x + 4 y - 3 = 0$ is
A ${tan}^{- 1} 3$
B ${tan}^{- 1} 5$
C ${tan}^{- 1} (2 \sqrt{3})$
D ${tan}^{- 1} (2 \sqrt{2})$
The acute angle between the line represented by $x^{2} - 6 x y + 5 y^{2} + 10 x - 4 y + 9 = 0$ is
A ${tan}^{- 1} (\frac{3}{2})$
B ${tan}^{- 1} (\frac{3}{4})$
C ${tan}^{- 1} (\frac{2}{3})$
D ${tan}^{- 1} (\frac{4}{3})$

The acute angle between the line $3 x - 4 y = 5$ and the circle $x^{2} + y^{2} - 4 x + 2 y - 4 = 0$ is $θ$ . Then $9 cos θ =$