The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
The correct Answer is:9
3x+6y=k
or 3x+6yk=1 (1)
Also, 2x2+2xy+3y2−1=0 (2)
Now, homogenizing (2) with the helop of (1), we get
2x2+2xy+3y2−(3x+6yk)2=0
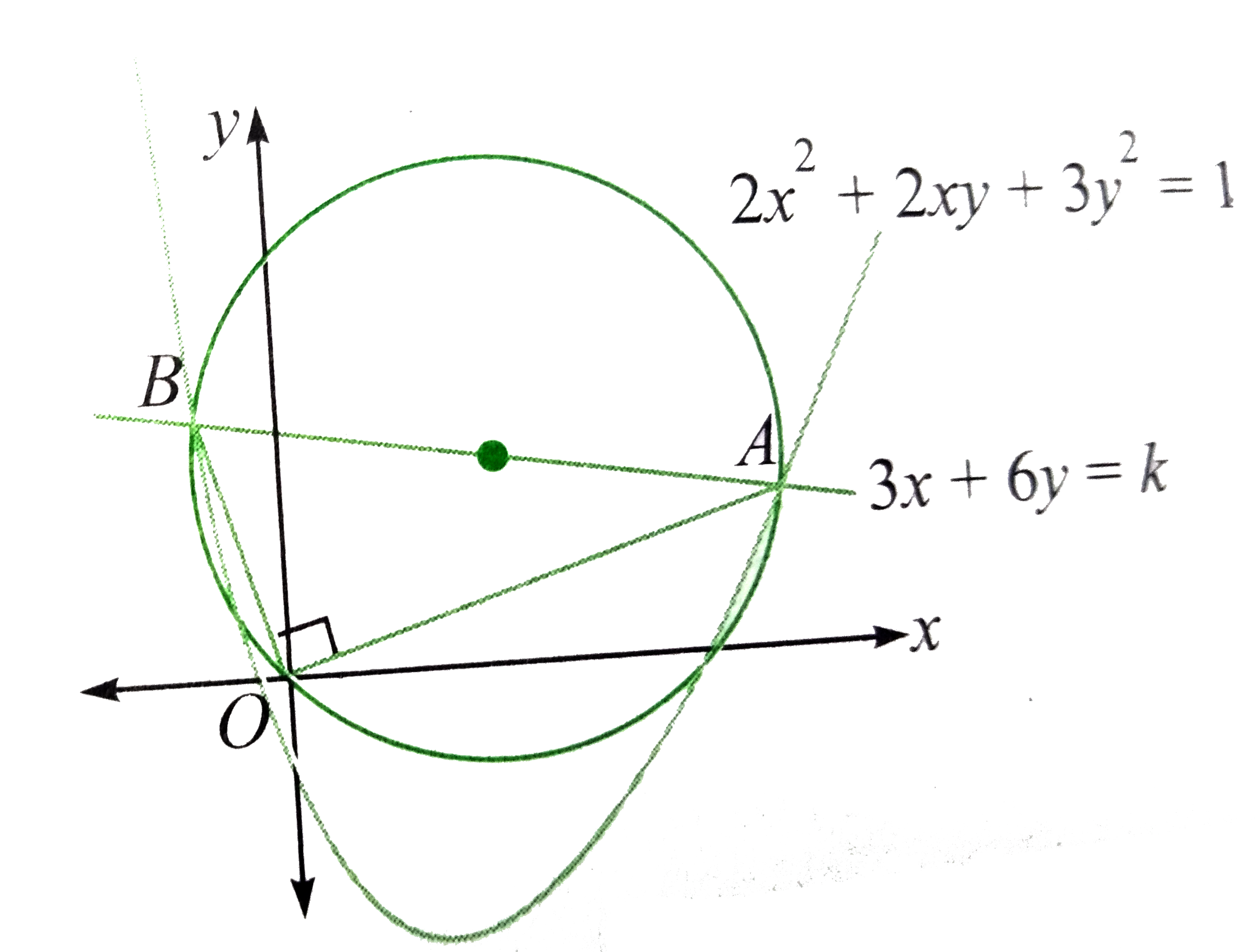
or k2(2x2+2xy+3y2)−(3x+6y)2=0 (3)
This is the equation of the pair of straight lines OA and OB. ltbr> Now, AB is diameter. Then OA and OB are perpendicular . So, Coefficient of x2+ Coefficient of y2=0
or (2k2−9)+(3k2−36)=0
or 5k2=45
or k2=9
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Main Previous Year
10 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)
14 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Matrix)
1 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
View SolutionLine 3x+by=k intersect the curve 2x2+3y2+2xy=1 at points A and B. Thecircle on AB as diameter passes through origin,then sum of all possible values of 'k' is
View Solution
Knowledge Check
The circle x2+y2=4 cuts the circle x2+y2−2x−4=0 at the points A and B. If the circle x2+y2−4x−k=0 passes through A and B then the value of k , is
A-4B0C-8D4The line 3x−2y=k meets the circle x2+y2=4r2 at only one point, if k2=
A20r2B52r2C529r2D209r2If the curve y=ax2+bx+c passes through the point (1, 2) and the line y = x touches it at the origin, then
Aa = 1, b = -1, c = 0Ba = 1, b = 1, c = 0Ca = -1, b = 1, c = 0Dnone of these
The line (a−1)x+ay=1 intersects the curve 3x2+4xy+2y2=1 at points A and B. The circle on AB as diameter passes through the origin, then find the sum of all possible value of 'a'.
View SolutionSuppose the ine x+2y=k intersects the conic 2x2+5xy+3y2=1 in two points A and B. If the circle with AB as diameter passes through the origin, then value of 14k2 is
View SolutionIf the line 2x-3y=k passing through the origin, then the value of k is
View SolutionIf the line 2x-3y=k passing through the origin, then the value of k is
View SolutionIf a line3x+4y−20=0 intersect the circle x2+y2=25at the point A,B then The radius of the circle which is orthogonal to the given circle and which pass through points A and B is
View Solution
