Find the length of the chord of contact with respect to the point on the director circle of circle x2+y2+2ax−2by+a2−b2=0 .
We have circle S1≡(x+a)2+(y−b)2=2b2
Equation of dirctor circle S2≡(x+a)2+(y−b)2=4b2.
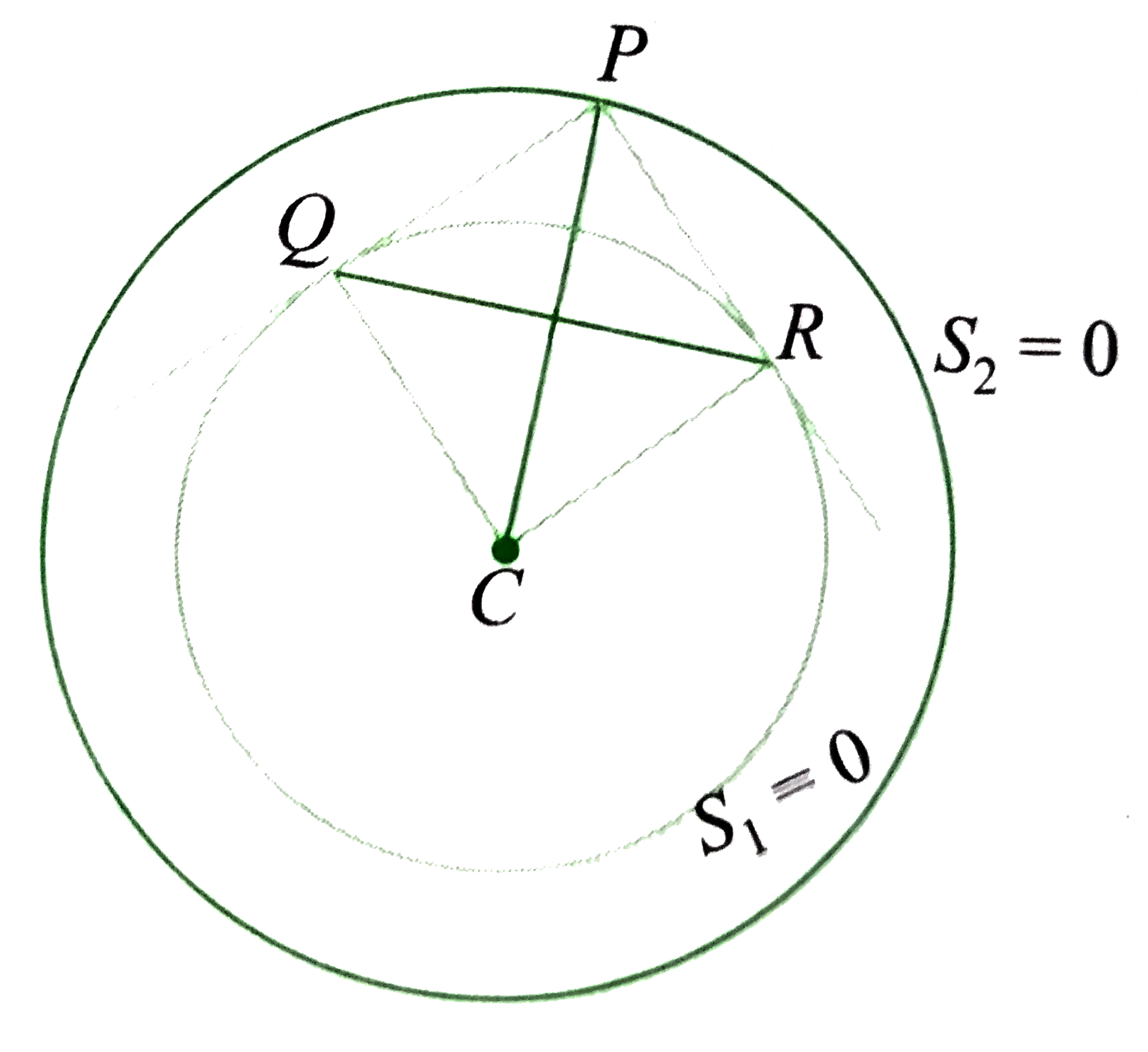
Tangents PQ and PR are drawn to circle S1=0 from a point P on the director circle S2=0.
So, QR is the chord of contact.
In the figure, PQCR is a square.
∴QR=CP= Radius of director circle =2b
Topper's Solved these Questions
Similar Questions
Find the length of the chord of contact with respect to the point on the director circle of circle x2+y2+2ax−2by+a2−b2=0 .
View SolutionFind the equation of the chord of contact of the point (1,2) with respect to the circle x2+y2+2x+3y+1=0
View SolutionThe length of chord of contact of the point (3,6) with respect to the circle x2+y2=10 is
View SolutionThe length of the chord of contact of the tangents drawn from the point (−2,3) to the circle x2+y2−4x−6y+12=0 is:
View SolutionThe equation of director circle to the circle x2+y2=8 is
View SolutionFind the equation to the chord of contact of the tangents drawn from an external point (−3,2) to the circle x2+y2+2x−3=0.
View SolutionLength of chord of contact of point (4,4) with respect to the circle x2+y2−2x−2y−7=0 is
View SolutionThe midpoint of the chord y=x−1 of the circle x2+y2−2x+2y−2=0 is
View SolutionFind the length of the chord cut-off by y=2x+1 from the circle x2+y2=2
View SolutionThe Equation of director circle of x2+y2+6x+2y+1=0 is
View Solution