A car moving at a constant velocity of 46 m/s passes a traffic cop who is readily sitting on his motorcycle. After a reaction time of 1.0s, the cop begins to chase the spoeding car with a constant acceleration of 4.0 m/s2. How much time does the cop then need to overtake the speeding car ?
The correct Answer is:23.96≈24s
To solve the problem step by step, we will analyze the motion of both the car and the cop.
Step 1: Calculate the distance traveled by the car during the cop's reaction time.
The car is moving at a constant velocity of 46 m/s. The cop has a reaction time of 1.0 seconds before he starts chasing the car.
Distance traveled by the car during the reaction time:
Distance=Velocity×Time=46m/s×1.0s=46m
Step 2: Set up the equations of motion.
After the reaction time, the cop starts chasing the car with a constant acceleration of 4.0 m/s².
- Distance traveled by the car after the reaction time (for time t):
dcar=46m+46m/s×t
This accounts for the initial distance of 46 m and the distance traveled at 46 m/s for time t.
- Distance traveled by the cop (starting from rest with acceleration):
dcop=12at2=12×4m/s2×t2=2t2
Step 3: Set the distances equal to find the time when the cop overtakes the car.
To find the time t when the cop overtakes the car, we set the distances equal:
46+46t=2t2
Step 4: Rearrange the equation.
Rearranging gives:
2t2−46t−46=0
Step 5: Solve the quadratic equation.
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=2, b=−46, and c=−46.
Calculating the discriminant:
b2−4ac=(−46)2−4×2×(−46)=2116+368=2484
Now, applying the quadratic formula:
t=46±√24844
Calculating √2484:
√2484≈49.84
Now substituting back:
t=46±49.844
Calculating the two possible values for t:
1. t=46+49.844≈95.844≈23.96s
2. t=46−49.844 gives a negative value, which we discard since time cannot be negative.
Final Answer:
The time needed for the cop to overtake the speeding car is approximately 24 seconds.
---
The car is moving at a constant velocity of 46 m/s. The cop has a reaction time of 1.0 seconds before he starts chasing the car.
Distance=Velocity×Time=46m/s×1.0s=46m
After the reaction time, the cop starts chasing the car with a constant acceleration of 4.0 m/s².
dcar=46m+46m/s×t
This accounts for the initial distance of 46 m and the distance traveled at 46 m/s for time t.
dcop=12at2=12×4m/s2×t2=2t2
To find the time t when the cop overtakes the car, we set the distances equal:
46+46t=2t2
Rearranging gives:
2t2−46t−46=0
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=2, b=−46, and c=−46.
b2−4ac=(−46)2−4×2×(−46)=2116+368=2484
t=46±√24844
√2484≈49.84
t=46±49.844
1. t=46+49.844≈95.844≈23.96s
2. t=46−49.844 gives a negative value, which we discard since time cannot be negative.
The time needed for the cop to overtake the speeding car is approximately 24 seconds.
Topper's Solved these Questions
- MOTION ALONG A STRAIGHT LINEBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( Single Correct Choice Type)
54 Videos
- MOTION ALONG A STRAIGHT LINEBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - PRACTICE QUESTIONS ( More than One Correct Choice Type )
6 Videos
- MOTION ALONG A STRAIGHT LINEBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION ALONG A STRAIGHT LINEEXERCISE - Checkpoints
5 Videos
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Practice Questions (Integer type )
7 Videos
- MOTION IN TWO AND THREE DIMENSIONSBOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MATRIX-MATCH)
10 Videos
Similar Questions
What will be the minimum stopping distance required by a car to stop if it is moving with a velocity of 40m/s with a deceleration of 20m/s3? How much time will it take to stop the car?
View SolutionThere are two cars on a straight road, marked as x axis. Car A is travelling at a constant speed of VA=9m/s. Let the position of the Car A, at time t=0, be the origin. Another car B is L=40m ahead of car A at t=0 and starts moving at a constant acceleration of a=1m/s2 (at t = 0). Consider the length of the two cars to be negligible and treat them as point objects.
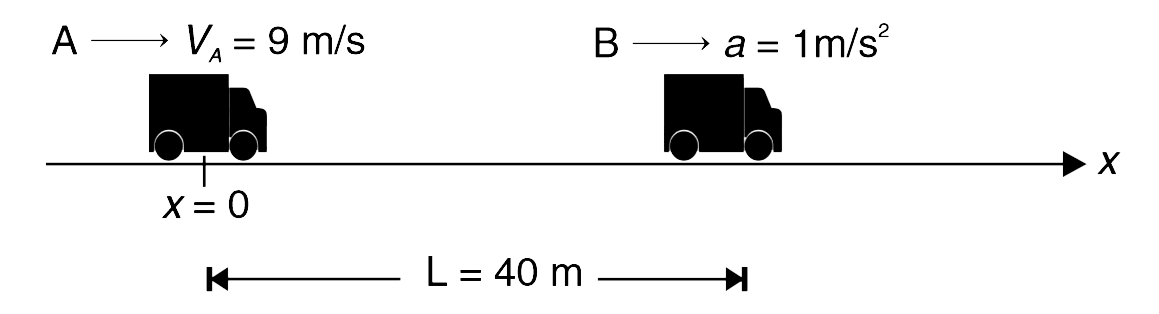
(a) Plot the position–time (x–t) graph for the two cars on the same graph. The two graphs intersect at two points. Draw conclusion from this.
(b) Determine the maximum lead that car A can have.
View Solution
Knowledge Check
In the previous problem if the car is moving with a constant acceleration of 2m/s2, the ball will fall behind the person at a distance
A32mB64mC96mD16mTwo cars A and B are at rest at the origin O. If A starts with a uniform velocity of20m/s and B starts in the same direction with a constant acceleration of 2m/s2, then the cars will meet after time
A10sB20sC30sD40sA car is moving eastward with velocity 10 m/s. In 20 sec, the velocity change to 10 m/s northwards. The averge acceleration in this time.
A1/√2m/s2 toward N-WB1/√2m/s2 towards N-EC1/√2m/s2 towards N-WD1/√2m/s2 towards N
A car is moving northwards at a constant speed of 5 m/s . It makes a right turn and continues to move with a constant speed of 5 m/s . Find the magnitude of change in velocity.
View SolutionTwo cars a and B starts motion with a velocity of 10m/s and 20m/s respectively. Car a moves with a constant acceleration of 2.5m/s2 and car B have constant acceleration of 0.5m/s2 as shown in figure. Find when car A will over take car B. Dimension of car is negligible as compare to distance. Find the time after which car A over takes car B.
View SolutionCar A starts from a point O and moves with constant velocity 9m/s. After 2s, another car B begins its journey from O and follows car A. If car B starts from rest and moves under constant acceleration 4m/s2, after how much time and at what distance from O the cars meet?
View SolutionA car moving with a velocity of 10m/s can be stopped by the application of a constant force F In a distance of 20m. If the velocity of the car is 30m/s. It can be stopped by this force in
View SolutionAt t=0 and x=0 , an initially stationary blue car begins to accelerate at a constant rate of 2.0m/s2 in the positive direction of the x -axis At =t = 2s a red car travelling in an adjecent lane and in the same direction passes x =0 with a speed of 8.0m/s and a constant accleration of 3.0m/s2 .the time when red car passes the blue car is :
View Solution
