If $cos θ = \frac{3}{5}, find the value of (\frac{5 cosec θ - 4 tan θ}{sec θ + cot θ}) .$

A

$\frac{24}{29}$

B

$\frac{1}{29}$

C

$\frac{8}{29}$

D

$\frac{11}{29}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

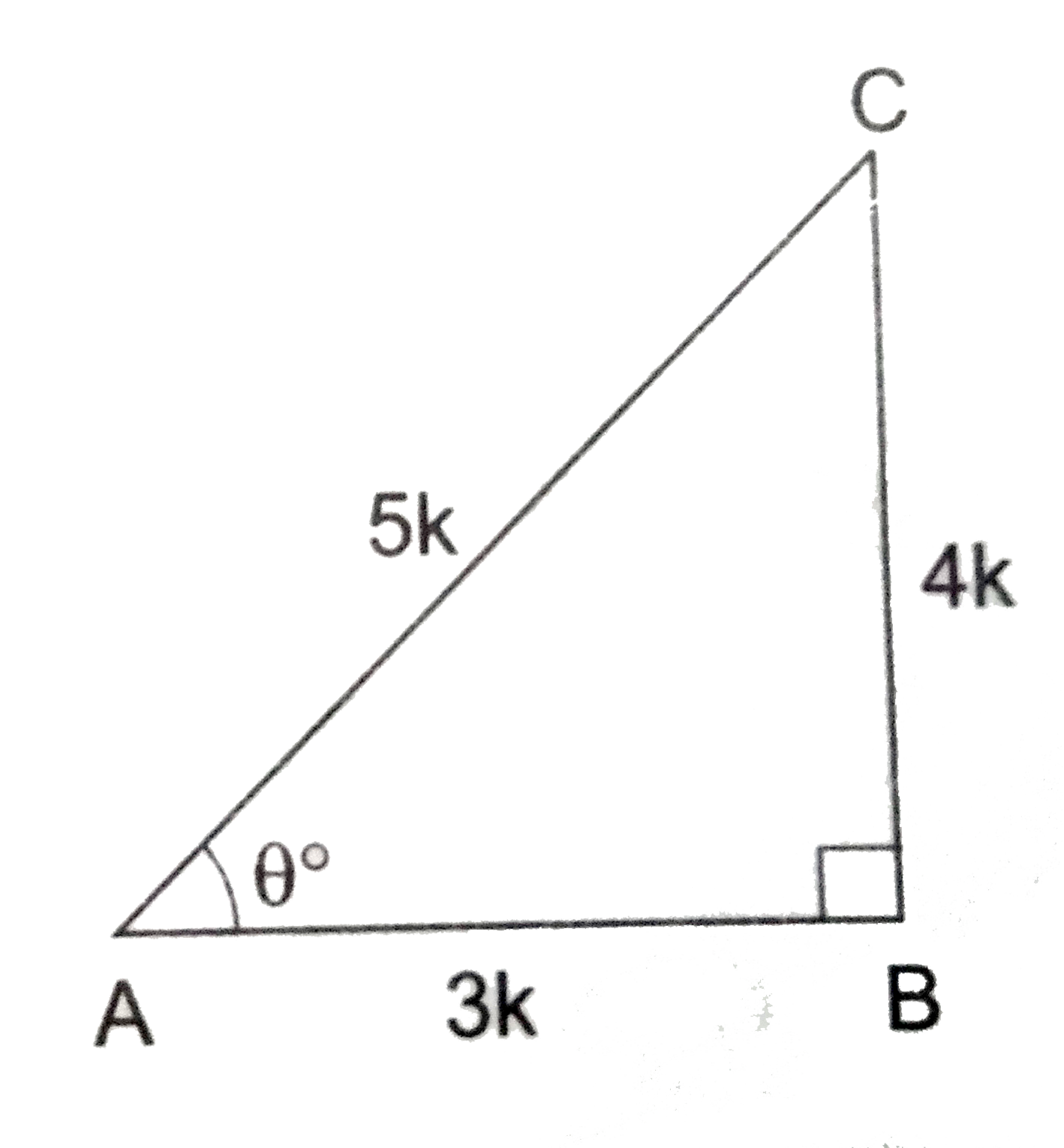
Let us draw a $Δ A B C in which ∠ B = 90^{\circ} .$
Let $∠ A = θ^{\circ} .$
then , $cos θ = \frac{A B}{A C} = \frac{3}{5} .$
Let $A B = 3 K and A c = 5 k$ , where k is positive .
By pythagoras' theorem , we have
$A C^{2} = A B^{2} + B C^{2}$
$\Rightarrow B C^{2} = A C^{2} - A B^{2} = {(5 k)}^{2} - {(3 k)}^{2} = 25 k^{2} - 9 k^{2} = 16 k^{2}$
$\Rightarrow B C = \sqrt{16 k^{2}} = 4 k .$
$∴ sec θ = \frac{1}{cos θ} = \frac{5}{3}, tan θ = \frac{B C}{A B} = \frac{4 k}{3 k} = \frac{4}{3},$
$cot θ = \frac{1}{tan θ} = \frac{3}{4}, and cosec θ = \frac{A C}{B C} = \frac{5 k}{4 k} = \frac{5}{4} .$
$∴ (\frac{5 cosec θ - 4 tan θ}{sec θ + cot θ}) = \frac{(5 \times \frac{5}{4} - 4 \times \frac{4}{3})}{(\frac{5}{3} + \frac{3}{4})} = \frac{(\frac{25}{4} - \frac{16}{3})}{(\frac{5}{3} + \frac{3}{4})}$
$= \frac{\frac{75 - 64}{12}}{\frac{20 + 9}{12}} = (\frac{11}{12} \times \frac{12}{29}) = \frac{11}{29} .$

Updated on:21/07/2023

|

Class 10 MATHS TRIGONOMETRIC RATIOS AND IDENTITIES

Topper's Solved these Questions

Similar Questions

Knowledge Check

If $sec θ = \frac{5}{4}, find the value of \frac{(2 cos θ - sin θ)}{(cot θ - tan θ)} = .$
A(12)/(7)
B(12)/(5)
C(1)/(7)
D(2)/(5)
If $5 cot θ = 3, find the value of (\frac{5 sin θ - 3 cos θ}{4 sin θ + 3 cos θ}) .$
A $\frac{11}{29}$
B $\frac{16}{19}$
C $\frac{16}{29}$
D $\frac{34}{29}$
If $cos θ = \frac{- 3}{5} and π < θ < \frac{3 π}{2}$ , then find the value of $\frac{sec θ - tan θ}{cos e c θ + cot θ}$ is-
A6
B8
C $- 2$
D $- 4$

RS AGGARWAL-TRIGONOMETRIC RATIOS-Exercise 10

Home
Ask Doubt
Profile