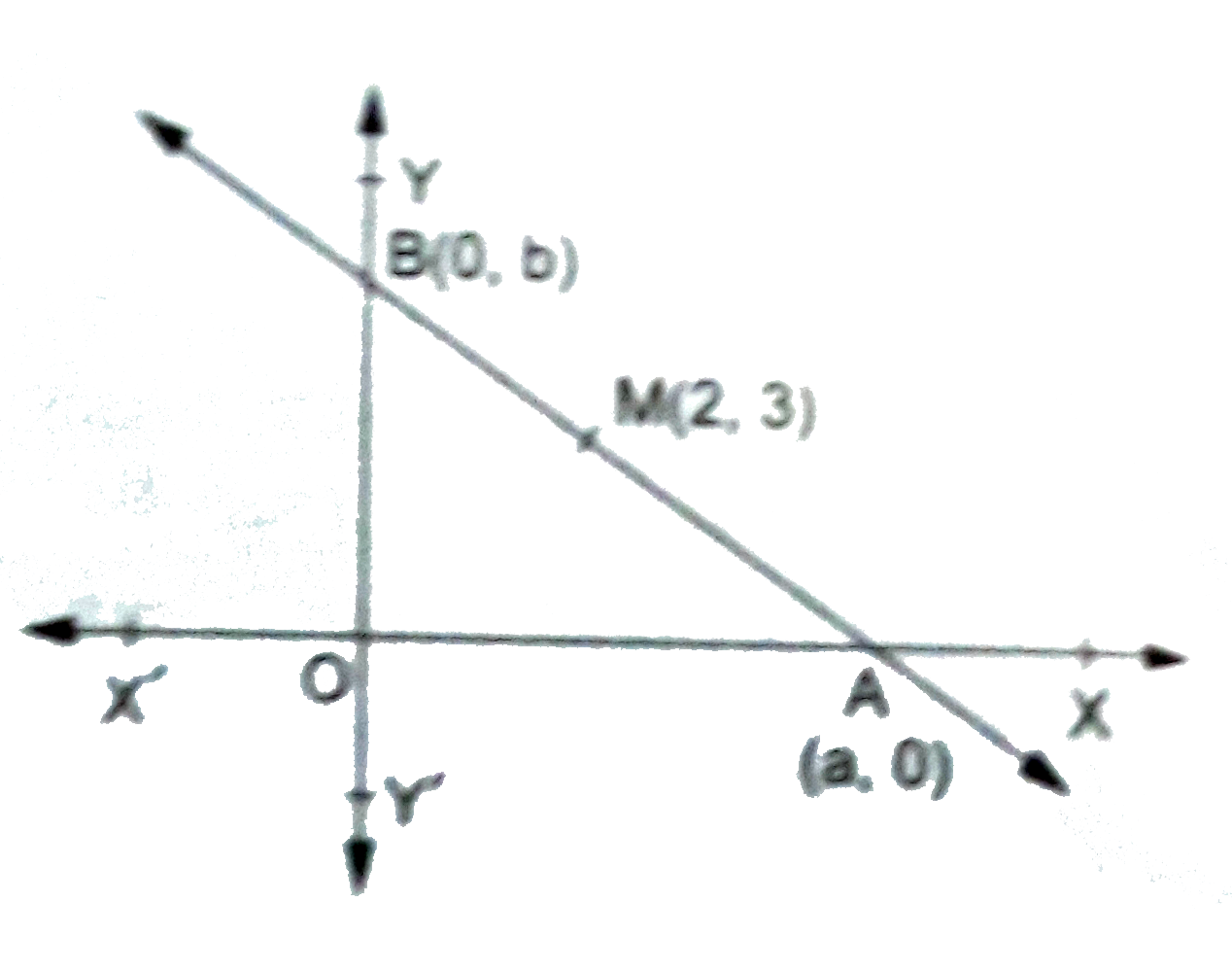
Find the equation of the line through (2,3) so that the segment of the line intercepted between the axes is bisected at this point.

Video Solution

Text Solution

Verified by Experts

Let AB be the given line segment making intercepts a and b on the x-axis and y-axis respectively. Then, the equation of line AB is $\frac{x}{a} + \frac{y}{b} = 1$
Morever, these points are A(a,0) and B(0,b). Let M(2,3) be the midpoint of AB. Then, $\frac{a + 0}{2} = 2 and \frac{0 + b}{2} = 3$
$\Rightarrow a = 4 and b = 6$
Hence, the required equation of the given line is
$\frac{x}{4} + \frac{y}{6} = 1 \Leftrightarrow 3 x + 2 y - 12 = 0$
Hence, the required equation is 3x+2y-12=0

Updated on:21/07/2023

The equation of the line through (2,3) so, that the segment of the line intercepted between the axes is bisected at the point is
A3x+2y=12
B2x+3y=12
C3x+2y=6
DNone of the above
What is the equation of the line through $(1, 2)$ so that the segment of the line intercepted between the axis is bisected at this point ?
A $2 x - y = 4$
B $2 x - y + 4 = 0$
C $2 x + y = 4$
D $2 x + y + 4 = 0$
The equation of the line through the origin which bisects the portion of the line 3x-y=12 intercepted between the axes is
A3x+y=0
By=3x
Cx=3y
Dnone of these