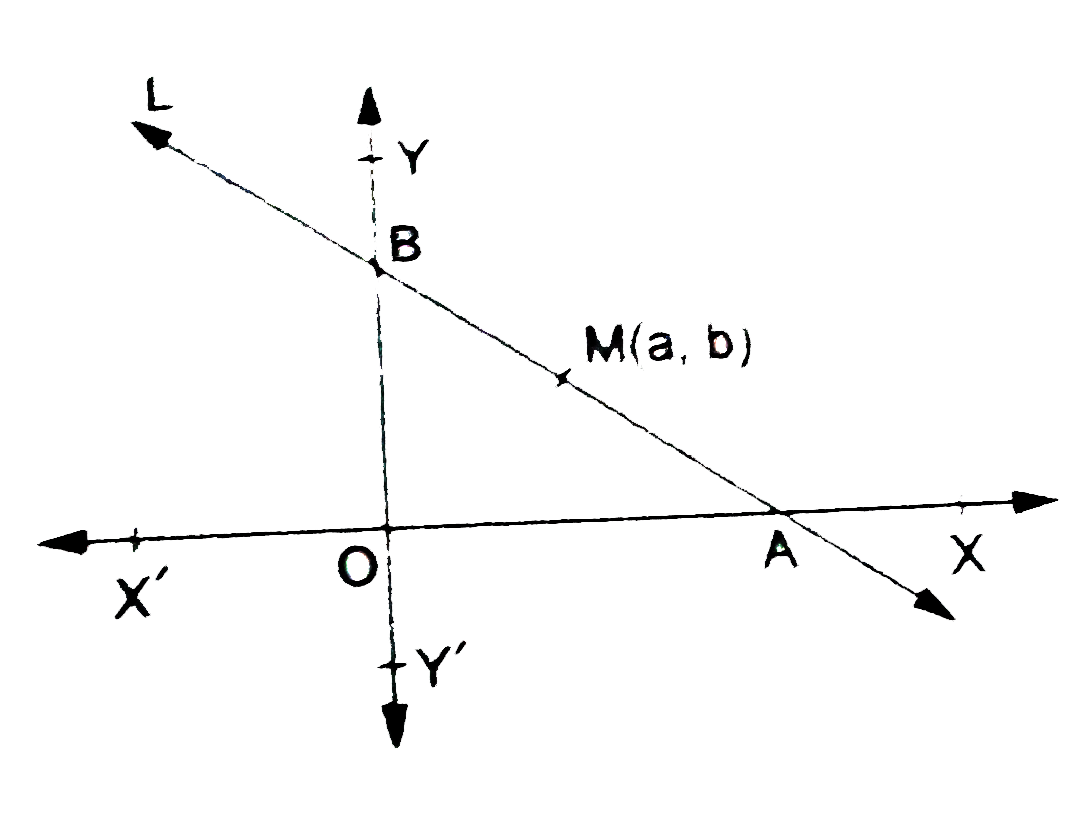
P (a, b) is the midpoint of a line segment between axes. Show that equation of the line is $\frac{x}{a} + \frac{y}{b} = 2 .$

Video Solution

Text Solution

Verified by Experts

Let the required equation of the line be $\frac{x}{c} + \frac{y}{d} = 1$
Then, it cuts the axis and y-axis at the points A(c,0) and B(0,d). Let M(a,b) be the midpoint of AB. Let M(a,b) be the midpoint of AB.
Then, $\frac{c + 0}{2} = a and \frac{0 + d}{2} = b$
$\Leftrightarrow c = 2 a and d = 2 b$
So, the required equation is
$\frac{x}{2 a} + \frac{y}{2 b} = 1 \Leftrightarrow \frac{x}{a} + \frac{y}{b} = 2$
Hence, the required equation is $\frac{x}{a} + \frac{y}{b} = 2$

|

Updated on:21/07/2023

Class 11 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $(\frac{3}{2}, \frac{5}{2})$ is the midpoint of line segment intercepted by a line between axes, the equation of the line is
A $5 x + 3 y + 15 = 0$
B $3 x + 5 y + 15 = 0$
C $5 x + 3 y - 15 = 0$
D $3 x + 5 y - 15 = 0$
Question 2 - Select One
If (2,3) is the midpoint of the portion of a line intercepted between the co-ordinate axes , then the equation of the line is
A2x+3y=12
B2x+3y+12=0
C3x+2y=12
Dnone of these
Question 3 - Select One
If the point (a, b) divides a line between the axes in the ratio 2 : 3, the equation of the line is
A $a x + b y = 5$
B $b x + a y = 5$
C $\frac{2 x}{a} + \frac{3 y}{b} = 5$
D $2 a x + 3 b y = 5$

RS AGGARWAL-STRAIGHT LINES -SOLVED-EXAMPLE

Home
Ask Doubt
Profile