A coil of cross-sectional area A having n turns is placed in uniform magnetic field B. When it is rotated with an angular velocity $ω$ , the maximum e.m.f. induced in the coil will be :

Video Solution

Text Solution

Verified by Experts

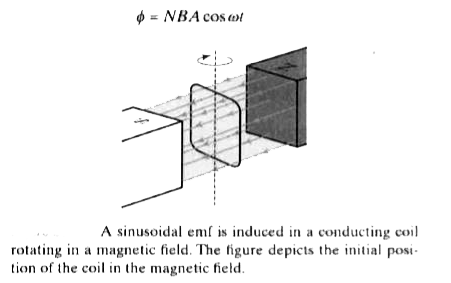
When the coil lies in the plane perpendicular to the magnetic field, the magnetic flux through the coil has its greatest possible value, NBA. As the coil rotates, the surface area vector associated with the coil is no longer perpendicular to the magnetic field, and thus changes with time. At any time, the angle between the surface area vector and the magnetic field is $ω$ t, so the flux through the coil is
$ϕ = N B A \cos ω t$
Therefore, the emf is
$e m f = - \frac{d}{d t} (N B A \cos ω t) = N B A ω \sin ω t$

Updated on:21/07/2023

A coil having an area $A_{0}$ is placed in a magnetic field which changes from $B_{0}$ to $4 B_{0}$ in a time interval t. The e.m.f. induced in the coil will be
A $\frac{3 B_{0}}{A_{0} t}$
B $\frac{4 A_{0} B_{0}}{t}$
C $\frac{4 B_{0}}{A_{0} t}$
D $\frac{3 A_{0} B_{0}}{t}$
A coil having an area $A_{0}$ is placed in a magnetic field which changes from $B_{0}$ to $4 B_{0}$ in a time interval $t$ . The e.m.f. induced in the coil will be
A $\frac{3 A_{0} B_{0}}{t}$
B $\frac{4 A_{0} B_{0}}{t}$
C $\frac{3 B_{0}}{A_{0} t}$
D $\frac{4 B_{0}}{A_{0} t}$
A rectangular coil of single trun, having area $A$ , rotates in a uniform magnetic field $B$ with an angular velocity $ω$ about an axis prependicular to the field. If initially the plane of the coil is perpendicular to the field, then the average induced emf when it has rotate through $90^{\circ}$ is
A $\frac{ω B A}{π}$
B $\frac{ω B A}{2 π}$
C $\frac{ω B A}{4 π}$
D $\frac{2 ω B A}{4 π}$