Question

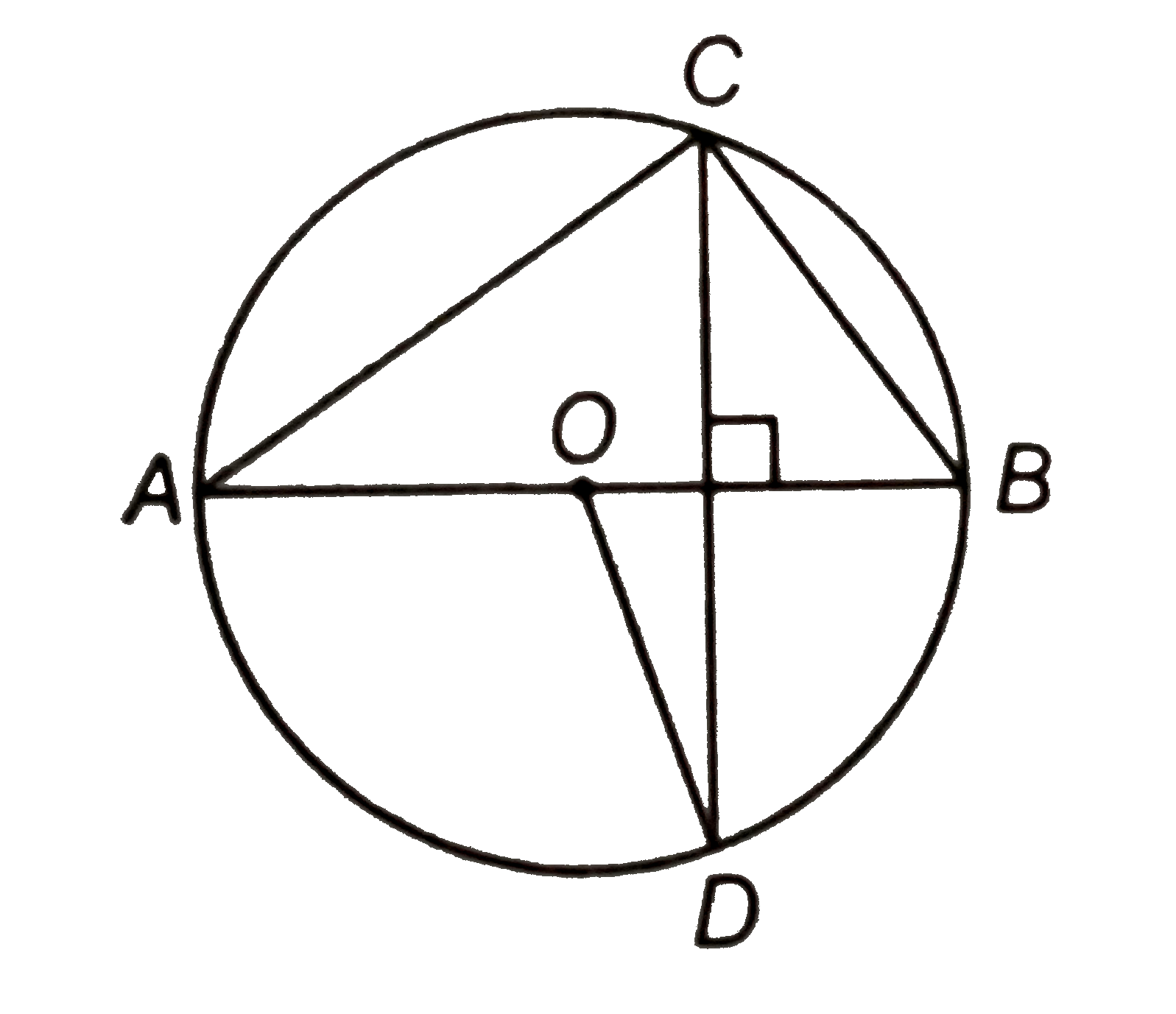
If O is the circumcentre of ${\displaystyle \Delta ABC}$ and OD ${\displaystyle \perp }$ BC, then ${\displaystyle \angle BOD}$ must be equal to

Options

A. `angleA`
B. `1/2 angle A`
C. `1/2 angleB`
D. `1/2 angleC`

Answer

A

Explanation

To solve the problem, we need to find the value of angle BOD given that O is the circumcenter of triangle ABC and OD is perpendicular to BC.

1. Understanding the Circumcenter:
The circumcenter O of triangle ABC is the point where the perpendicular bisectors of the sides of the triangle intersect. It is also the center of the circumcircle that passes through all three vertices A, B, and C.

Hint: Remember that the circumcenter is equidistant from all vertices of the triangle.

2. Drawing the Circumcircle:
Draw a circle with center O and points A, B, and C on the circumference. This circle represents the circumcircle of triangle ABC.

Hint: Visualize the triangle and its circumcircle to understand the relationships between angles.

3. Identifying the Perpendicular:
Since OD is perpendicular to BC, we know that angle ODB and angle ODC are both right angles (90 degrees).

Hint: Use the property of perpendicular lines to identify right angles in the triangle.

4. Analyzing Triangle OBD and Triangle OCD:
In triangles OBD and OCD:
- OB = OC (both are radii of the circumcircle)
- OD is common to both triangles.
- Angle ODB = Angle ODC = 90 degrees.

Hint: Use the criteria for congruence (Side-Angle-Side) to establish relationships between the triangles.

5. Establishing Congruence:
Since triangles OBD and OCD are congruent (by the criteria mentioned), we can conclude:
- Angle BOD = Angle COD.

Hint: Congruent triangles have equal corresponding angles.

6. Relating Angles BOC and BOD:
Since angle BOC is formed by angles BOD and COD, we can express it as:
- Angle BOC = Angle BOD + Angle COD = 2 * Angle BOD.

Hint: Remember that the angle at the center (BOC) is double the angle at the circumference (BOD).

7. Using the Inscribed Angle Theorem:
According to the inscribed angle theorem, the angle subtended by an arc at the center of the circle is double the angle subtended at any point on the remaining part of the circle. Therefore:
- Angle BOC = 2 * Angle A (where A is the angle at vertex A).

Hint: This theorem is crucial for relating angles in circles.

8. Final Calculation:
From the previous steps, we have:
- 2 * Angle BOD = 2 * Angle A.
- Dividing both sides by 2 gives us:
- Angle BOD = Angle A.

Hint: Simplifying equations can help you find the desired angle.

Conclusion:
The value of angle BOD must be equal to angle A.

Final Answer:
Angle BOD = Angle A.