A gun of mass M fires a bullet of mass m with a horizontal speed V. The gun is fitted with a concave mirror of focal length f facing towards the receding bullet. Find the speed of separation of the bullet and the image just after the gun was fired.
speed of recoil of gun then according to conservation of linear momentum we can write the following :
MV.=mV⇒V.=mV/M
Speed of bullet with respect to mirror fitted with gun
=V.+V=mVM+V=V(1+mM)
Mirror formula can be written as :
1v+1u=1f
⇒1u=1f−1u
⇒v=ufu−f
On differentiating the above result we get the following :
⇒dvdt=(u−f)×f(du)dt−fu×(dudt−0)(u−f)2
⇒dvdt=−f2(du)dt(u−f)2
Initially u=0 hence
dudt=dudt
Hence initial speed of image with respect to mirror is the same as speed of object with respect to mirror but in opposite direction . Hence both the speed are to be added to get relative speed . Speed of object with respect to mirror is calculated as V(1+mM) , hence relative speed of separation of bullet and its image will be 2V(1+mM)
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT FILE SOLVED (TEXT BOOK EXERCISES)
38 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT (EXemplar Problems Obejective Questions) (Multiple Choice Questions (Type-I)
10 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Conceptual Questions
40 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
A gun of mass M fires a bullet of mass m with a horizontal speed V. The gun is fitted with a concave mirror of focal length f facing towards the receding bullet. Find the speed of separation of the bullet and the image just after the gun was fired.
View SolutionA gun of mass 1kg fires a bullet of mass 10gm with a Kinetic energy 200J .The velocity of recoil of the gun is?
View Solution
Knowledge Check
A loaded spring gun of mass M fires a bullet of mass m with a velocity v at an angle of elevation θ. The gun is initially at rest on a horizontal smooth surface. After firing, the centre of mass of the gun and bullet system
A(a) moves with velocity vMmB(b) moves with velocity vmmcosθ in the horizontal directionC(c) does not move in horizontal directionD(d) moves with velocity v(M−m)M+m in the horizontal directionA gun of mass 20 kg has bullet of mass 0.1 kg in it. The gun is free to recoil and 804 J of recoil energy is released on firing the gun. The speed of bullet (in ms−1) is
A√804×2010B√2010804C√8042010D√804×4×103A man fires a bullet of mass 200 g at a speed of 5 m/s . The gun is of one kg mass. By what velocity the gun rebounds backwards
A0.1m/sB10m/sC1m/sD0.01m/s
A gun of mass 4 kg initially at rest fires a bullet of mass 4g with a speed of 50m/s as seen by an observer on ground. Find the impulse on bullet and recoil speed of the gun.
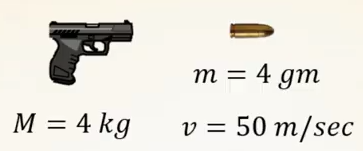
View SolutionA gun fires a bullet of mass 50 g with a velocity of 30 m s−1 because of which the gun recoils with a velocity 1 m s−1. Find the mass of the gun.
View SolutionA gun fires a bullet of mass 50 g with a velocity of 30 m s−1 because of which the gun recoils with a velocity 1 m s−1. Find the mass of the gun.
View SolutionA gun fires a bullet of mass 50g with a velociy of 30m/s. Due to this, the gun is pushed back with a velocity of 1m/s, then the mass of the gun is :
View SolutionA gun fires two bullets at 60∘and30∘ with the horizontal. The bullets strike at some horizontal distance. The ratio of maximum height for the two bullet is
View Solution
