In a ΔPQR, points M and N are on the sides PQ and PR respectively such that PM 0.6. PQ and NR=0.4PR. What percentage of the area of the triangle PQR does that of triangle PMN form?
A)60%
B)50%
C)36%
D)55%
The correct Answer is:C
To solve the problem, we need to find the percentage of the area of triangle PMN in relation to the area of triangle PQR. Let's break it down step by step.
Step 1: Understand the dimensions of the triangles
Given:
- PM = 0.6 * PQ
- NR = 0.4 * PR
Assuming PQ = PR = 10 cm (as per the example in the video).
Calculating the lengths:
- PM = 0.6 * 10 cm = 6 cm
- MQ = PQ - PM = 10 cm - 6 cm = 4 cm
- NR = 0.4 * 10 cm = 4 cm
- NR = PR - NR = 10 cm - 4 cm = 6 cm
Step 2: Calculate the area of triangle PQR
The area of triangle PQR can be calculated using the formula:
Area=12×base×height
For triangle PQR:
- Base = PQ = 10 cm
- Height = PR = 10 cm (assuming the height is the same as the length of PR for simplicity)
Thus, the area of triangle PQR is:
AreaPQR=12×10×10=50cm2
Step 3: Calculate the area of triangle PMN
For triangle PMN:
- Base = PM = 6 cm
- Height = PN (which is also 6 cm, as NR = 4 cm and the total height remains the same)
Thus, the area of triangle PMN is:
AreaPMN=12×6×6=18cm2
Step 4: Calculate the percentage of the area of triangle PMN to triangle PQR
To find the percentage:
Percentage=(AreaPMNAreaPQR)×100
Substituting the areas we calculated:
Percentage=(1850)×100=36%
Conclusion
The area of triangle PMN forms 36% of the area of triangle PQR.
---
Given:
- PM = 0.6 * PQ
- NR = 0.4 * PR
- PM = 0.6 * 10 cm = 6 cm
- MQ = PQ - PM = 10 cm - 6 cm = 4 cm
- NR = 0.4 * 10 cm = 4 cm
- NR = PR - NR = 10 cm - 4 cm = 6 cm
The area of triangle PQR can be calculated using the formula:
Area=12×base×height
- Base = PQ = 10 cm
- Height = PR = 10 cm (assuming the height is the same as the length of PR for simplicity)
AreaPQR=12×10×10=50cm2
For triangle PMN:
- Base = PM = 6 cm
- Height = PN (which is also 6 cm, as NR = 4 cm and the total height remains the same)
AreaPMN=12×6×6=18cm2
To find the percentage:
Percentage=(AreaPMNAreaPQR)×100
Percentage=(1850)×100=36%
The area of triangle PMN forms 36% of the area of triangle PQR.
Topper's Solved these Questions
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)
52 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.5
60 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
- HCF AND LCMBOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions
18 Videos
Similar Questions
Knowledge Check
In a ΔPQR, points M and N are on the sides PQ and PR respectively such that PM 0.6. PQ and NR=0.4PR. What percentage of the area of the triangle PQR does that of triangle PMN form?
AA)60%BB)50%CC)36%DD)55%In a ΔPQR, points M and N are on the sides PQ and PR respectively such that PM 0.6. PQ and NR=0.4PR. What percentage of the area of the triangle PQR does that of triangle PMN form?
A60%B50%C36%D55%In △PQR L and M are two points on the sides PQ and PR respectively such that LMII QR. If PL = 2cm, LQ = 6 cm and PM= 1.5 cm, then MR (in cm) is
A0.5B4.5C9D8
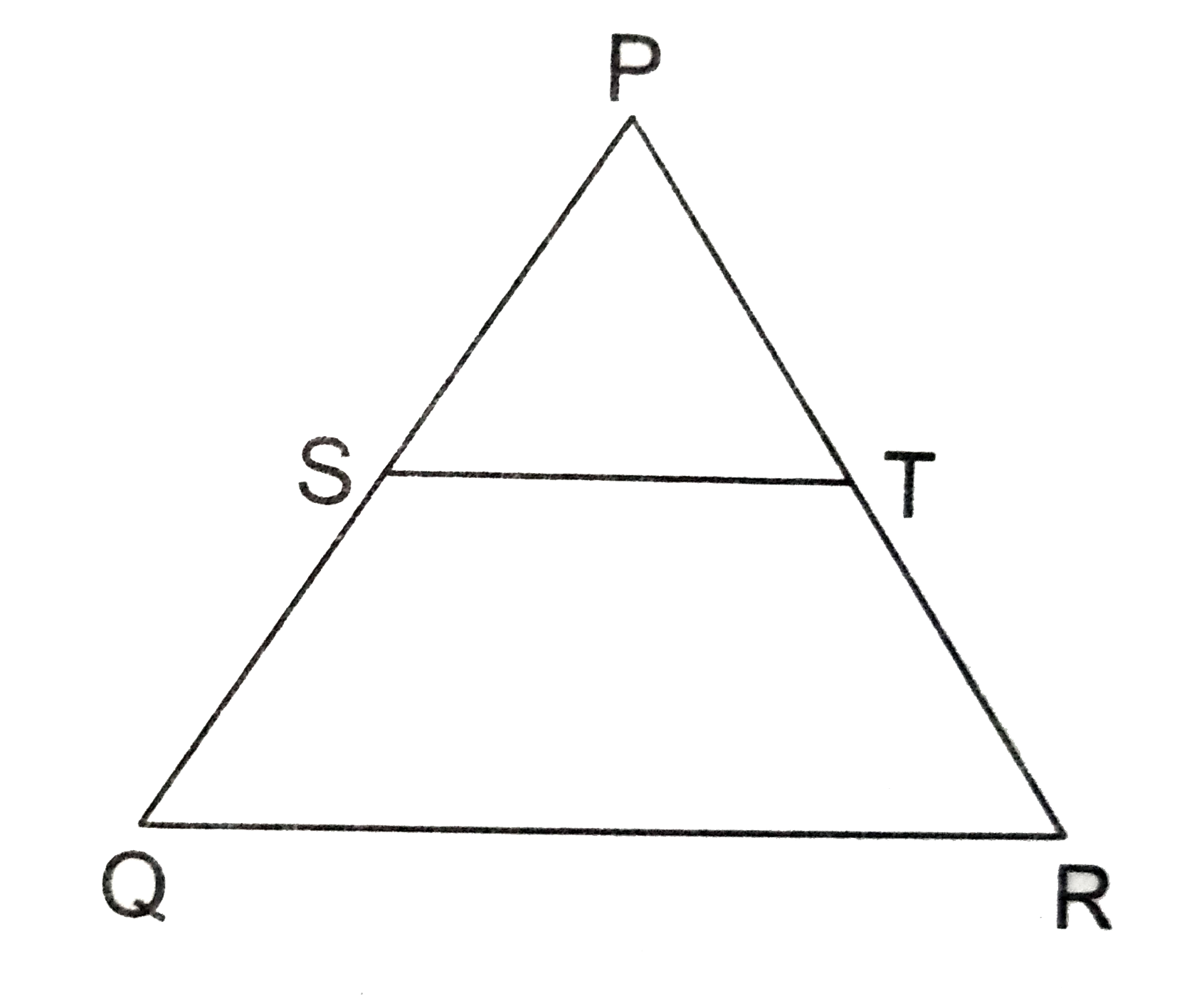
S and T are points on the sides PQ and PR, respectively of ΔPQR, such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ΔPST and ΔPQR.
View SolutionIn the adjoining figure, S and T are points on the sides PQ and PR respectively of ΔPQR such that PT=2cm,TR=4cmandST is parallel to QR. Find the ratio of the ares ofΔPSTandΔPQR.
View SolutionIn triangle PQR, points A,B, and C are taken on PQ, PR and QR respectively such that QC=AC and CR=CB . If ∠QPR=40∘ then ∠ACB is equal to :
View SolutionIn a triangle PQR, PQ=20 cm and PR =6 cm, the side QR is :
View SolutionIn ΔPQR,right-angled at Q,X,Y and Z are points on sides PR,RQandPQrespectively such that QX⊥PR,XY⊥RQandXZ⊥PQ . Find the value of XZ If PZ=4cmandZQ=9cm.
View Solution