Question

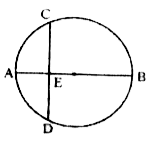
Suppose AB is a diameter of a circle, whose centre is at O and C be any point on the circle. If CD is perpendicular to AB and CD = 12 cm, AD = 16 cm, then BD is equal to

Options

A. 10 cm
B. 12 cm
C. 8 cm
D. 9 cm

Answer

D

Explanation

To solve the problem, we need to find the length of BD given the following information:

- CD is perpendicular to AB (the diameter of the circle).
- CD = 12 cm.
- AD = 16 cm.

Let’s denote the lengths as follows:
- Let BD = x cm.

1. Understand the Geometry:
- We have a circle with diameter AB, where O is the center of the circle.
- Point C is on the circumference of the circle, and CD is perpendicular to AB at point D.

2. Use the Pythagorean Theorem:
- In triangle ACD, we can apply the Pythagorean theorem since CD is perpendicular to AB.
- According to the theorem:
\( AC^2 = AD^2 + CD^2 \)

3. Substitute the Known Values:
- We know AD = 16 cm and CD = 12 cm.
- Substitute these values into the equation:
\( AC^2 = 16^2 + 12^2 \)

4. Calculate the Squares:
- Calculate \(16^2\) and \(12^2\):
\( 16^2 = 256 \)
\( 12^2 = 144 \)

5. Add the Squares:
- Now add these two results:
\( AC^2 = 256 + 144 = 400 \)

6. Find AC:
- Take the square root to find AC:
\( AC = \sqrt{400} = 20 \text{ cm} \)

7. Use the Length of AB:
- Since AB is the diameter, and we know that AD + BD = AB, we can express AB as:
\( AB = AD + BD = 16 + x \)

8. Relate AC and AB:
- Since AC is the radius, and AB is the diameter:
\( AB = 2 \times AC = 2 \times 20 = 40 \text{ cm} \)

9. Set Up the Equation:
- Now we can set up the equation:
\( 16 + x = 40 \)

10. Solve for x (BD):
- Rearranging gives:
\( x = 40 - 16 = 24 \text{ cm} \)

11. Find BD:
- Since BD is the remaining part of the diameter:
\( BD = 24 \text{ cm} \)

Final Answer:
BD = 24 cm.