Consider the following statements in respect of an equilateral ΔABC.
I. There is a point P inside the ΔABC, such that each of its sides subtends an angle of 120∘ at P. II. There is a point P inside ΔABC, such that the ΔPBC, iis obtuse angled and A is the orthocentre of ΔPBC. Which of the above statements is/are correct ?
Only I
Only II
Both I and II
Neither I nor II
The correct Answer is:A
To solve the problem, we need to analyze the two statements regarding the equilateral triangle ΔABC.
Step 1: Analyze Statement I
The first statement claims that there exists a point P inside the triangle ΔABC such that each side of the triangle subtends an angle of 120∘ at point P.
- Understanding the Geometry: In an equilateral triangle, all angles are equal to 60∘. When we consider point P inside the triangle, the angles subtended by the sides at point P can be calculated using the property of angles in a triangle.
- Finding Angles: If point P is positioned such that it subtends 120∘ at each side, we can visualize this. The angles around point P must sum to 360∘. If each side subtends 120∘, then the total subtended angle would be 3×120∘=360∘, which is valid.
- Conclusion for Statement I: Therefore, Statement I is correct.
Step 2: Analyze Statement II
The second statement claims that there exists a point P inside ΔABC such that triangle ΔPBC is obtuse-angled and point A is the orthocenter of triangle ΔPBC.
- Understanding Orthocenter: The orthocenter of a triangle is the point where the altitudes intersect. In an equilateral triangle, the orthocenter coincides with the centroid and the circumcenter.
- Conditions for Obtuseness: For triangle ΔPBC to be obtuse, one of its angles must be greater than 90∘. If A is the orthocenter of triangle ΔPBC, then the altitude from A to side BC must intersect at a point within the triangle.
- Geometric Impossibility: However, since A is a vertex of the equilateral triangle, it cannot serve as the orthocenter of triangle ΔPBC while maintaining the obtuse condition. The orthocenter of triangle PBC cannot be at vertex A because it would contradict the properties of the triangle.
- Conclusion for Statement II: Therefore, Statement II is incorrect.
Final Conclusion
Only Statement I is correct, while Statement II is incorrect.
Summary of the Solution
- Statement I is correct: There exists a point P inside ΔABC such that each side subtends an angle of 120∘ at P.
- Statement II is incorrect: There cannot be a point P such that A is the orthocenter of triangle PBC while PBC is obtuse.
Final Answer
Only Statement I is correct.
---
The first statement claims that there exists a point P inside the triangle ΔABC such that each side of the triangle subtends an angle of 120∘ at point P.
- Finding Angles: If point P is positioned such that it subtends 120∘ at each side, we can visualize this. The angles around point P must sum to 360∘. If each side subtends 120∘, then the total subtended angle would be 3×120∘=360∘, which is valid.
- Conclusion for Statement I: Therefore, Statement I is correct.
The second statement claims that there exists a point P inside ΔABC such that triangle ΔPBC is obtuse-angled and point A is the orthocenter of triangle ΔPBC.
- Conditions for Obtuseness: For triangle ΔPBC to be obtuse, one of its angles must be greater than 90∘. If A is the orthocenter of triangle ΔPBC, then the altitude from A to side BC must intersect at a point within the triangle.
- Geometric Impossibility: However, since A is a vertex of the equilateral triangle, it cannot serve as the orthocenter of triangle ΔPBC while maintaining the obtuse condition. The orthocenter of triangle PBC cannot be at vertex A because it would contradict the properties of the triangle.
- Conclusion for Statement II: Therefore, Statement II is incorrect.
Only Statement I is correct, while Statement II is incorrect.
- Statement I is correct: There exists a point P inside ΔABC such that each side subtends an angle of 120∘ at P.
- Statement II is incorrect: There cannot be a point P such that A is the orthocenter of triangle PBC while PBC is obtuse.
Only Statement I is correct.
Topper's Solved these Questions
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.1
45 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - INTRODUCTORY EXERCISE - 12.2
100 Videos
- GEOMETRYBOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)
52 Videos
- FUNDAMENTALS BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 2
40 Videos
- HCF AND LCMBOOK - ARIHANT SSCCHAPTER - HCF AND LCMEXERCISE - Exercise Higher Skill Level Questions
18 Videos
Similar Questions
P is a point on the side AB of ΔABC , such that 5AP =2AB.
View SolutionConsider the following statements :
1. The orthocentre of a triangle always lies inside the triangle.
2. The centroid of a triangle always lies inside the triangle.
3. The orthocentre of a right angled triangle lies on the triangle.
4.The centroid of a right angled triangle lies on the triangle.
Which of the above statements are correct ?
View Solution
Knowledge Check
Let O be a point inside ΔABC such that
∠OAB=∠OBC=∠OCA=θ
Area of ΔABC is equal toA(a2+b2+c24)tanθB(a2+b2+c24)cotθC(a2+b2+c22)tanθD(a2+b2+c22)cotθLet O be a point inside ΔABC such that
∠OAB=∠OBC=∠OCA=θ
cotA+cotB+cotC is equal toAtan2θBcot2θCtanθDcotθConsider the following statements
I. If G is the centroid of ΔABC, then
GA = GB = GC.II. If H is the orthocentre of ΔABC, then HA = HB = HC.
Which of the statements given above is/are correct ?AOnly IBOnly IICBoth I and IIDNeither I nor II
P is a point on the side BC off the ΔABC and Q is a point such that PQ is the resultant of AP,PB and PC. Then, ABQC is a
View SolutionEach side of an equilateral triangle subtends an angle of 60∘ at the top of a tower hm high located at the centre of the triangle. It a is the length of each side of the triangle, then prove that 2a2=3h2.
View SolutionConsider the following statements.
I. Melting point of a solid.
II. Melting point is used for the identification of type of solids.
Which of the above two statement(s) is/are correct?
View SolutionA straight wire of finite length carrying current I subtends an angle of 60∘ at point P as shown. The magnetic field at P is
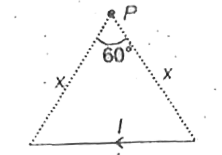
View SolutionConsider the following statements:
I. There exists no ΔABC for which SinA+SinB=SinC
II. If the angles of a triangle are in the ratio 1:2:3, then its sides will be in the ratio 1:√3:2
Which of the above statement(s) is/are correct?
View Solution
