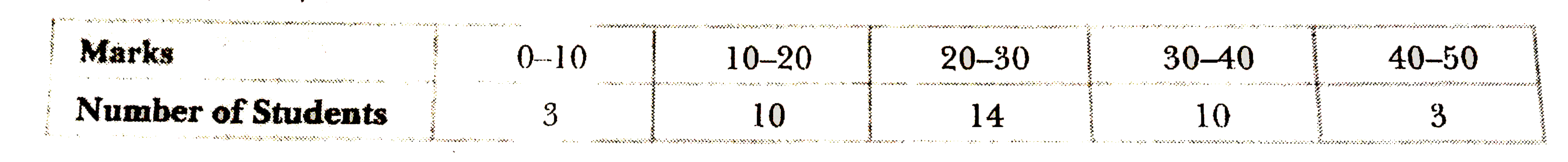The frequency distribution of the marks obtained by 28 students in a test carrying 40 marks is given below :

If the mean of the above data is 20 , then find the difference between x and y .
A
3
B
2
C
1
D
0
Video Solution
Text Solution
The correct Answer is:d
(i) Find the mid-values (xi) of class intervals .
(ii) Find ∑fx∑f
(iii) Equate ∑fx∑f with mean , and find the values of x and y .
|
Topper's Solved these Questions
- STATISTICS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - STATISTICS EXERCISE - Level 3
14 Videos
- STATISTICS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - STATISTICS EXERCISE - Level 1
28 Videos
- SHARES AND DIVIDENDSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SHARES AND DIVIDENDSEXERCISE - Level 3
15 Videos
- TAXATION BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TAXATION EXERCISE - LEVEL 3
10 Videos
Similar Questions
Knowledge Check
The marks obtained by 60 students in a certain test are given below :
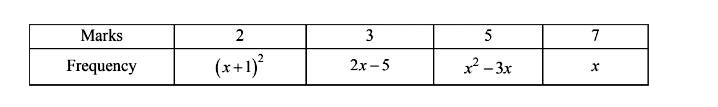
Median of the above data isA68.33B70C68.11DNone of theseIf for somes x∈R the frequency distribution of marks obtained by 20 students in a test is:

Then the mean of the marks is:A2.5B3.0C2.8D3.2The frequency distribution of marks obtained by 28 students in a test carrying 40 marks is given below:
Marks:0−1010−2020−3030−40Number of students:6xy6
If the mean of the above data is 20, then the difference between x and y isA3B2C1D0
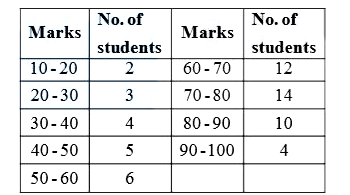
The marks obtained by 110 students in an examination are given below:
Find the mean marks of the studens.
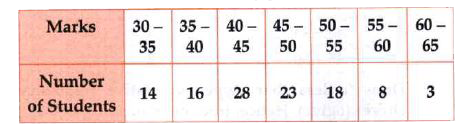
View SolutionFollowing data gives the marks obtained by 100 students in a test. Find the median
View SolutionThere are 60 students in a class. The following is the frequency distribution of the marks obtained by the students in a test:

Where "p" is positive integer. Determine the mean and standard deviation of the marks.
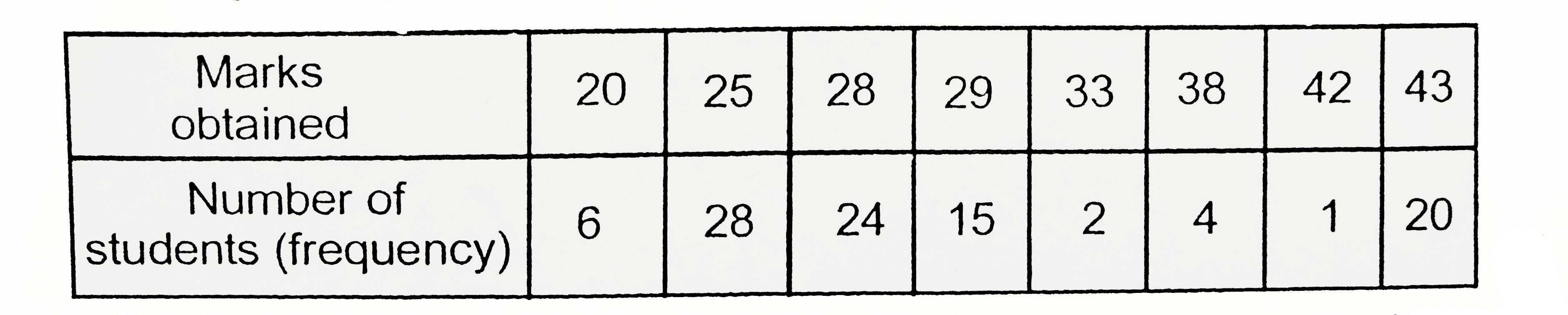
View SolutionThe marks (maximum marks 100) obtained by 20 students in a test are given below :
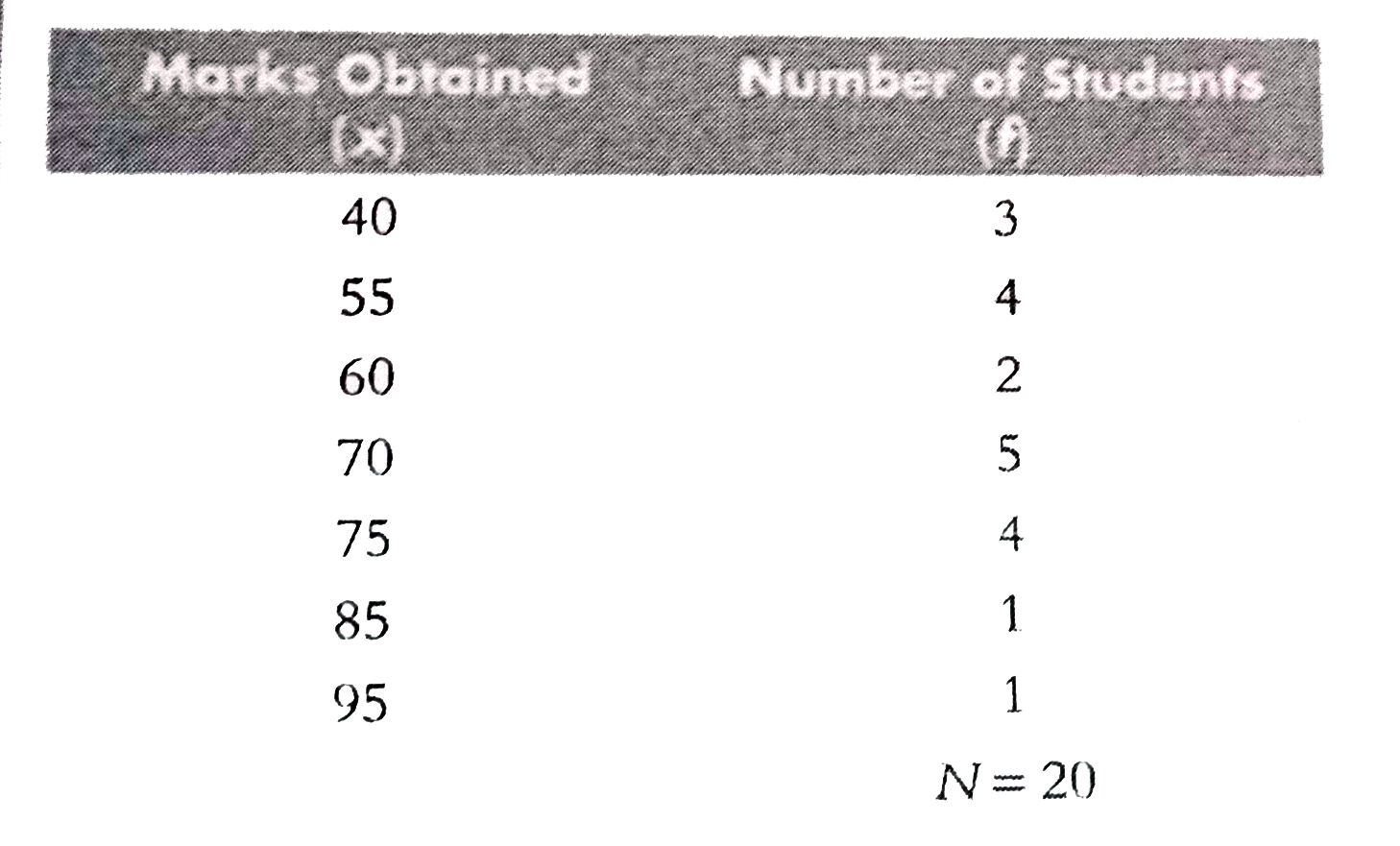
Find the mean marks of the 20 students.
View SolutionIn the given table, frequency distribution of marks obtained by the stubents in a question paper of mathematics are given below

Then, median of marks is
View Solution