If for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is: 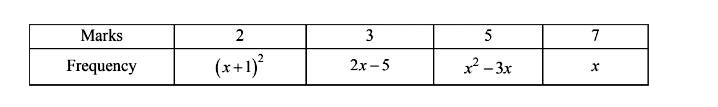
Then the mean of the marks is:
3.2
2.8
3
2.5
The correct Answer is:B
Total number of students are 20
So, (x+1)2+(2x−5)+(x2−3x)+x=20
x2+2x+1+2x−5+x2−3x+x=20
2x2+2x−24=0
x2+x−12=0
x=−4,x=3
But x is positive so, x=3
Now average marks (mean of the marks) =16×2+1×3+0×5+7×320=5620=2.8
Similar Questions
The frequency distribution of the marks obtained by 28 students in a test carrying 40 marks is given below :

If the mean of the above data is 20 , then find the difference between x and y .
View SolutionThere are 60 students in a class. The following is the frequency distribution of the marks obtained by the students in a test:

Where "p" is positive integer. Determine the mean and standard deviation of the marks.
View Solution
Knowledge Check
If for somes x∈R the frequency distribution of marks obtained by 20 students in a test is:

Then the mean of the marks is:A2.5B3.0C2.8D3.2The table shows the marks obtained by 25 students in a class test. Find the mean of the marks obtained
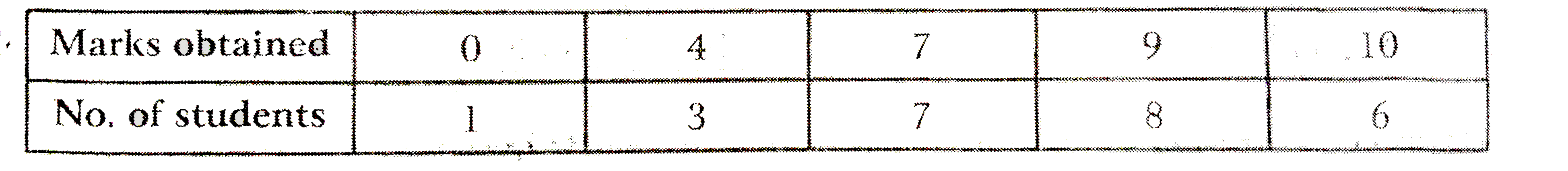 A7.72B7.65C7.4D7
A7.72B7.65C7.4D7If for some x∈R the frequency distribution of the marks obtained by 20 statement in a test is :
Marks2357Frequency(x+12)(2x−5)(x2−3x)x then the mean of the marks is :A3.2B3C2.5D2.8
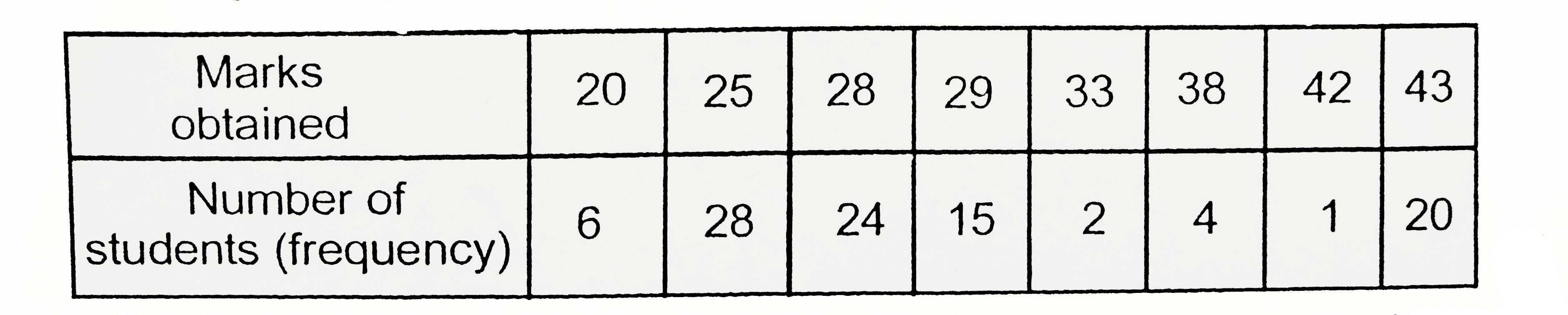
Following data gives the marks obtained by 100 students in a test. Find the median
View SolutionThe frequency distribution of marks obtained by students in a class test is given below .
Draw frequency polygon and ogive.
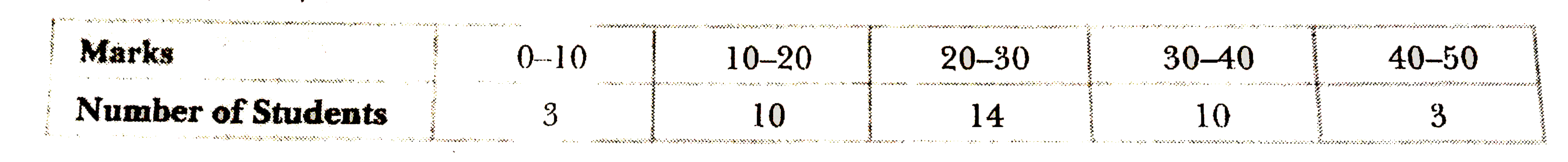
View SolutionThere are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks:, 0, 1, 2, 3, 4, 5 Frequency , x−2 , x , x2 , (x+1)2 , 2x , x+1. Determine mean and standard deviation of the marks.
View SolutionThe following table shows frequency distribution of marks secured by 100 students in a particular examination. Find the mean of the marks.
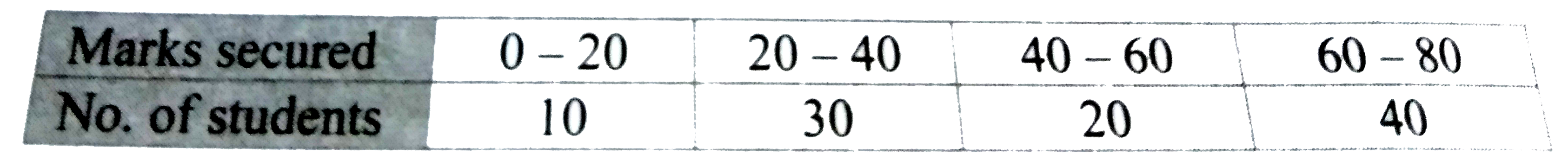
View SolutionIf the standard deviation for the marks obtained by a student in monthly tests is 36 then the variance is
View Solution
