Find the area bounded by $y = ∣ ∣ ∣ sin x - \frac{1}{2} ∣ ∣ ∣ and y = 1 for x \in [0, π]$

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $(\frac{π}{2} + 2)$ sq. units

$y = ∣ ∣ ∣ sin x - \frac{1}{2} ∣ ∣ ∣ = ⎧ ⎪ ⎪ ⎪ ⎪ ⎨ ⎪ ⎪ ⎪ ⎪ ⎩ \begin{matrix} \frac{1}{2} - sin x, & 0 \leq x \leq \frac{π}{6} sin x - \frac{1}{2}, & \frac{π}{6} < x < \frac{5 π}{6} \frac{1}{2} - sin x, & \frac{5 π}{6} \leq x \leq π \end{matrix}$

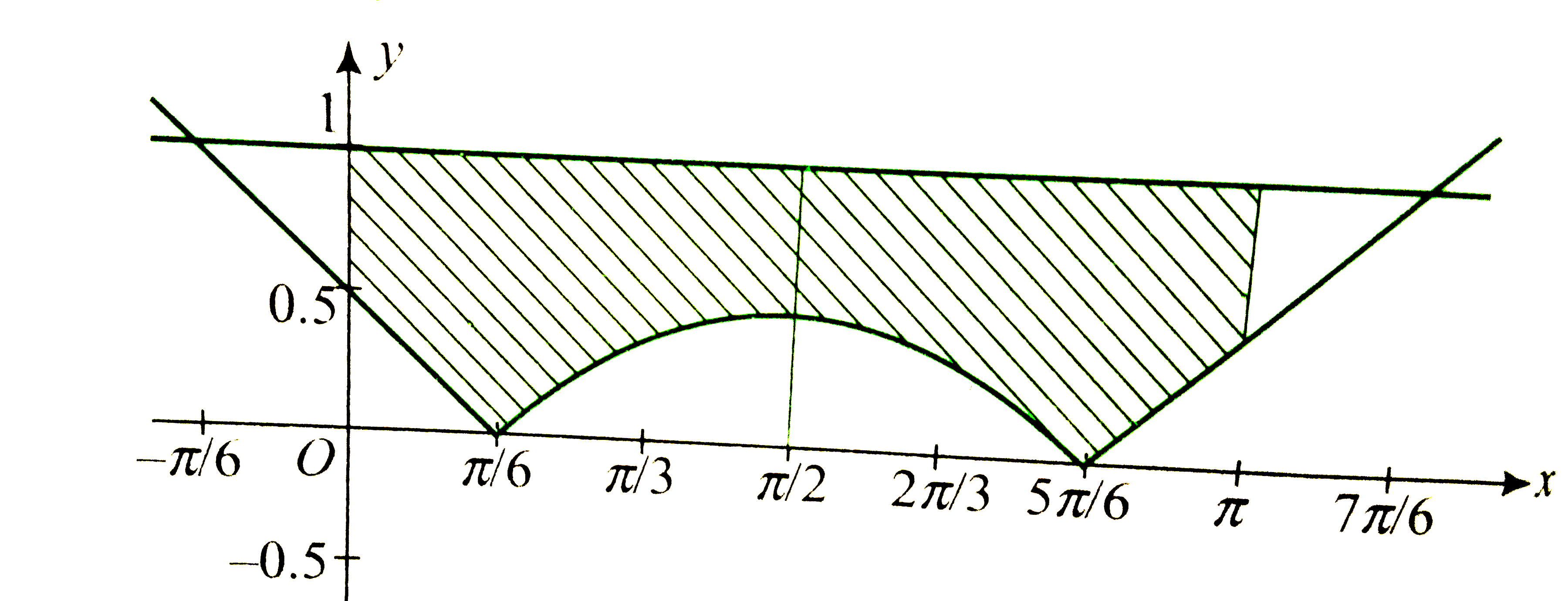
$∴ Required area is$
$A = π - 2 ⎛ ⎜ ⎜ ⎜ ⎝ \frac{π}{2} \int 0 (\frac{1}{2} - sin x) d x + \frac{π}{2} \int 0 (sin x - \frac{1}{2}) d x ⎞ ⎟ ⎟ ⎟ ⎠$
$π - 2 {(\frac{1}{2} x + cos x)}_{0}^{π / 6} + 2 {(- cos x - \frac{1}{2})}_{π / 6}^{π / 2}$
$= \frac{π}{2} + 2$

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

The area bounded by $| x | = 1 - y^{2} and | x | + | y | = 1$ is:
A $\frac{1}{3}$
B $\frac{1}{2}$
C $\frac{2}{3}$
D $1$

CENGAGE-AREA-Exercise 9.1

Home
Ask Doubt
Profile