Question

Find the area of the region bounded by the x-axis and the curves defined by $y=\tan x$,
(where $\frac{-\pi }{3}\le x\le \frac{\pi }{3}$) and $y=\cot x$.(where $\frac{\pi }{6}\le x\le \frac{2\pi }{3}$)

Answer

`log""(3)/(2)`sq. units

Explanation

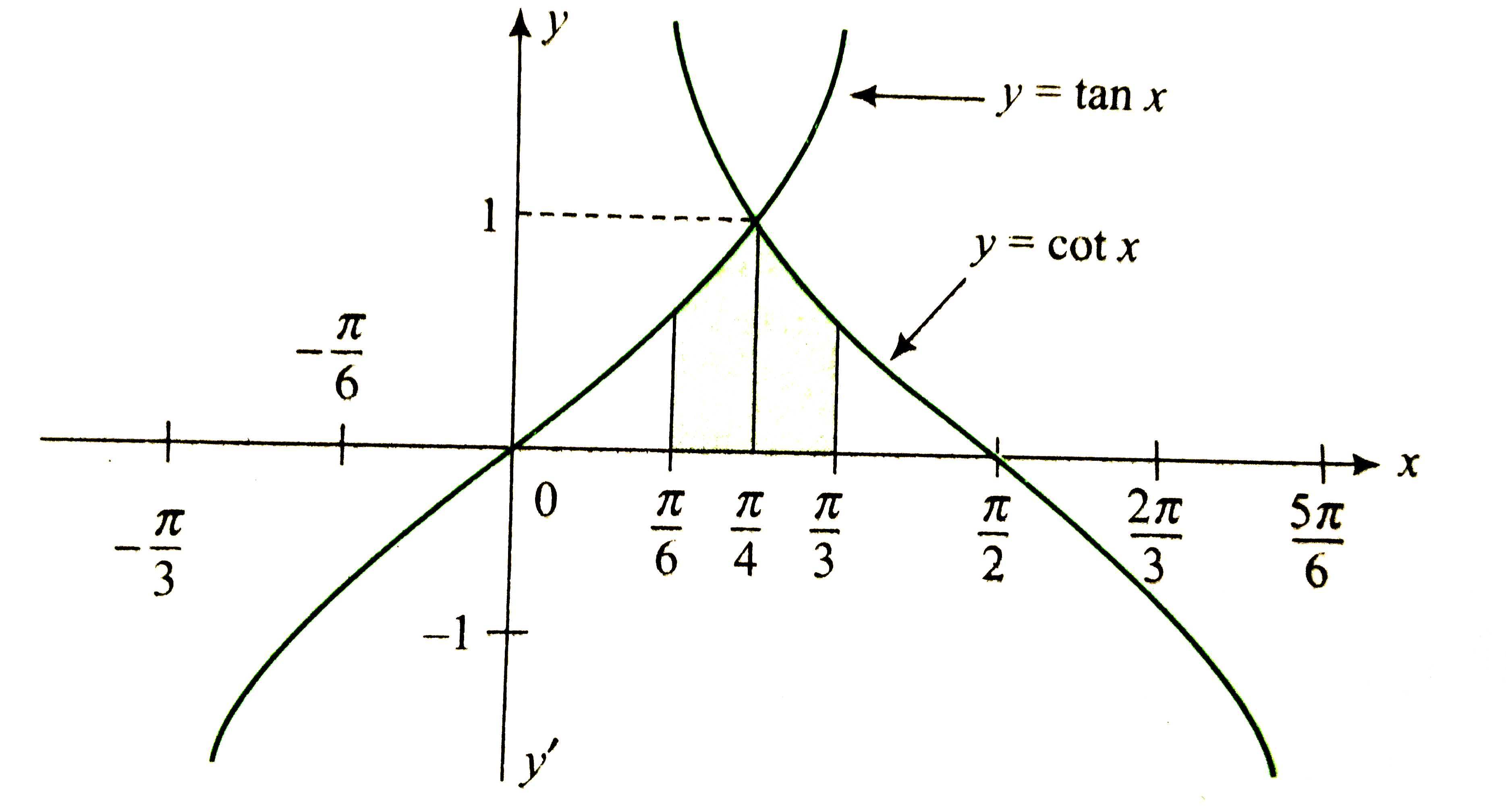
The two curves are
`y=tan x,"where "-pi//3lexpi//3" (1)"`
`y=cot x,"where "pi//6lexle3pi//2" (2)"`
At the point of intersection of the two curves
`tan x= cot x or tan^(2)x=1 or tan x = pm 1, x= pm pi//4`
Thus, the curves interset at `x=pi//4`
The required area is the shaded area. Therefore,
`A=int_(pi//6)^(pi//4)tan x dx +int_(pi//4)^(pi//3)cot x dx`
`=[ log sec x]_(pi//6)^(pi//4)+[log sin x]_(pi//4)^(pi//3)`
`=(logsqrt(2)-log""(2)/(sqrt(3)))+(log""(sqrt(3))/(2)-log""(1)/(sqrt(2)))`
`=logsqrt(2)+log""(sqrt(3))/(2)+log""(sqrt(3))/(2)+log sqrt(2)`
`=2(log sqrt(2)(sqrt(3))/(2))`
`=2log sqrt((3)/(2))=log 3//2` sq. units.