A series LCR circuit with L=0.12H,C=480nF, and R=23Ω is connected to a 230V variable frequency supply.
(a) What is the source frequency for which current amplitude is maximum? Find this maximum value.
(b) What is the source frequency for which average power absorbed by the circuit is maximum? Obtain the value of maximum power.
(c ) For which frequencies of the source is the power transferred to the circuit half the power at resonant frequency?
(d) What is the Q-factor of the circuit?
Given, inductance, L = 0.12 H,
Capacitance, C = 480 ×10−9F
Resistance, r = 23 Ω
rms voltage, Erms=230V
Peak voltage, E0=√2×230 v
I0 Is maximum at resonance frequency, ω0=1√LC
ω0=1√0.12×480×10−9=4167rads−1
f0=ω02π= 663 Hz,
Preak value of current is
I0=E0R=√2×23023 = 14.14 A
(b) power absorbed is also maximum at the resonating frequency
f0=663 Hz
When I0−14.14 A
so, |Pav|max=12l20R=12×(14.14)2×23 = 2300 W
(c) At resonating frequency, average power is given by
Pav=E2rmsR
For half the power of Pav we have
P = E2rmsZcosϕ
Also, cosϕ=RZandP=Pav/2
E2rmsZ.RZ=E2rms2R
⇒ Z2=2R2
R2+(ωL−1ωC)2=2R2
⇒ ωL−1ωC=R
⇒LCω2−RCω−1=0
⇒ ω=RC±√R2C2+4LC2LC
=R2L±√R2C24L2C2+4LC4L2C2
⇒ ω=R2L±√(R2L)2+1LC
=R2L±√(R2L)2+ω20
R2L<<ω0
ω=RL±ω0=95.8±4167
⇒ 4262.8 rad /s and 4071.2 rad/s
At these frequencies, current amplitude is (1√2) times the maximum current amplitude (at half the peak power points ) is
I0√2=14.14√2 = 10 A
(d) Q = ωLR=4167×0.1223=21.74
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - NCERT FILE EXEMPLAR PROBLEMS Subjective Question (VERY SHORT ANSWER TYPE QUESTIONS)
13 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - HIGHER ORDER THINKING SKILLS & ADVANCED LEVEL
7 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - NCERT FILE (TEXTBOOK EXERCISES )
11 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
Similar Questions
A series LCR circuit with L = 0.12 H, C = 480 nF, R = 232 is connected to a 230 V variable
frequency supply.
(a) What is the source frequency for which current amplitude is maximum ? Obtain this maximum value.
(b) What is the source frequency for which average power absorbed by the circuit is maximum ? Obtain the value of this maximum power.
(c) For which frequencies of the source is the power transferred to the circuit half the power at resonant frequency ? What is the current amplitude at these frequencies? (d) What is the Q-factor of the given circuit ?
View SolutionA series LCR circuit with L=0.125/πH,C=500/πnF,R=23Ω is connected to a 230V variable frequency supply. (a)What is the source frequency for which current amplitudes is maximum? Obtain this maximum value. (b)What is the source frequency for which average power absorbed by the circuit is maximum ?Obtain the value of this maximum power.(c )For what reactangle of the circuit, the power transferred to the circuit is half the power at resonance?What is the current amplitude at this reactangle ? (d)if ω is the angular frequency at which the power consumed in the circuit is half the power at resonance, write an expression for ω (e)What is the Q-factor (Quality factor) of the given circuit?
View Solution
Knowledge Check
Figure shows a series LCR circuit connected to a variable frequency 230 V source.
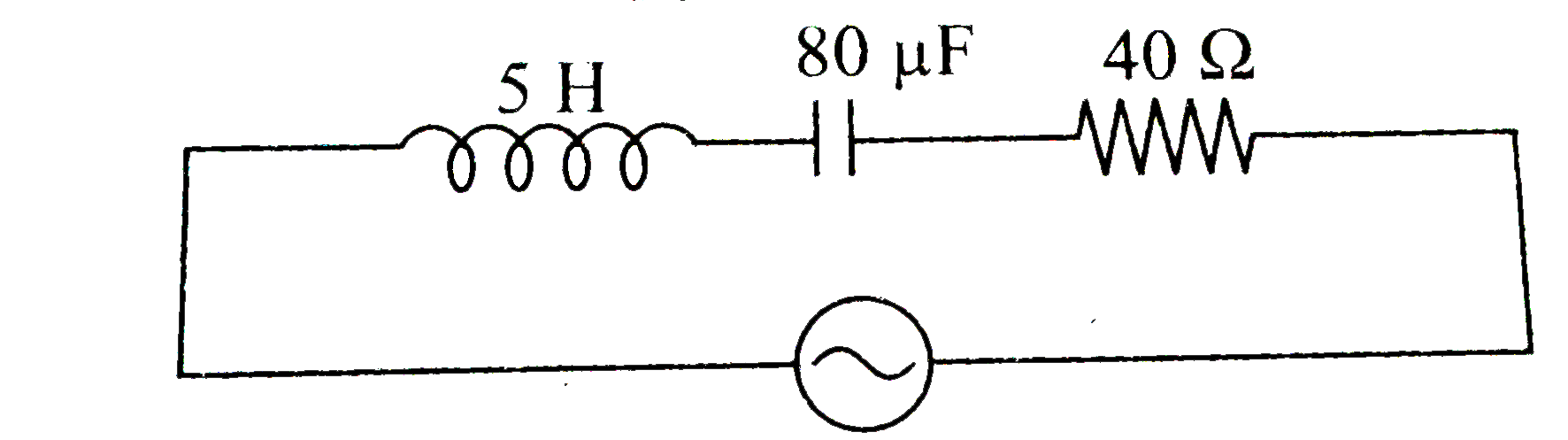
The source frequency which drives the circuit the circuit in resonance isA4 HzB5 HzC6 HzD8 Hz
A series LCR- circuit with L=0.12HC=4.8×10−7F,R=23Ω is connected to a variable frequency supply At what frequency is the current maximum ?
View SolutionA series L-C-R circuit with R=44Ω,C=8μFandL=50H is connected to a variable frequency 220 V ac suppy. Calculate angular frequency, impedance and current at resonance condition.
View SolutionA series LCR circuit with L=80mH,C=50μF and R=60ohm is connected to a variable frequency 220 V source. Determine (i) the source frequency which drives the circuitin resonance (iii) the quality factor Q of the circuit.
View SolutionA series LCR circuit having L=10mH, C=(400/π2)μF and R = 55 ohm is connected to 220 v variable frequency a.c. supply.
(i) Find frequency of source, for which average power absorbed by the circuit is maximum
(ii) Calculate the amplitude of current.
View SolutionA series LCR circuit with L=4.0h,C=100μF and R=60Ω is connected to a varialbe frequency 240 V source. Calcalate
(i) angular frequency of the source which drives the circuit in resonace,
(ii) current at the resonating frequency,
(iii) rms potential drop across the inductro at resonance.
View SolutionA series L-C-R circuit with R = 20Ω, L = 1.5 H and C =35μF is connected to a variable frequency 200 V, AC supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transfered to the circuit, what is the average power transfered to the circuit in one complete cycle ?
View SolutionA series LCR circuit with R=20Ω,L=1.5H and C=35μF is connected to a variable frequency 200V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power in Kw transferred to the circuit in one complete cycle?
View Solution
