Two identical charges of magnitude 0.4 mC are placed on diagonally opposite vertices of a square of side 10 cm. Calculate the amount of work done in slowly moving one of the charges from diagonally opposite vertex to adjacent vertex.
To solve the problem of calculating the work done in moving one charge from a diagonally opposite vertex to an adjacent vertex in a square, we can follow these steps:
1. Identify the Charges and Positions:
- We have two identical charges, Q=0.4mC=0.4×10−3C.
- They are placed at diagonally opposite vertices of a square with side length a=10cm=0.1m.
2. Calculate the Initial Distance:
- The distance between the two charges when they are at diagonally opposite corners is the diagonal of the square, given by:
r1=√2×a=√2×0.1m=0.1√2m
3. Calculate the Initial Potential Energy (E1):
- The potential energy E1 when the charges are at the diagonal corners is given by:
E1=kQ2r1=k(0.4×10−3)20.1√2
- Where k=14πϵ0≈9×109N m2/C2.
4. Calculate the Final Distance:
- When one charge is moved to an adjacent vertex, the distance between the two charges becomes:
r2=a=0.1m
5. Calculate the Final Potential Energy (E2):
- The potential energy E2 when one charge is moved to an adjacent corner is given by:
E2=kQ2r2=k(0.4×10−3)20.1
6. Calculate the Work Done (W):
- The work done in moving the charge is the change in potential energy:
W=E2−E1
- Substitute the expressions for E1 and E2:
W=kQ20.1−kQ20.1√2
- Factor out common terms:
W=kQ2(10.1−10.1√2)=kQ2(10−10/√2)
- Simplifying gives:
W=kQ2(10(1−1√2))
7. Substituting Values:
- Now substitute k, Q, and calculate:
W=9×109×(0.4×10−3)2×10(1−1√2)
- Calculate 1−1√2≈0.2929:
W≈9×109×0.16×10−6×10×0.2929
- Final calculation:
W≈4219.2J
Final Answer:
The amount of work done in moving one of the charges from a diagonally opposite vertex to an adjacent vertex is approximately 4219.2 J.
- We have two identical charges, Q=0.4mC=0.4×10−3C.
- They are placed at diagonally opposite vertices of a square with side length a=10cm=0.1m.
- The distance between the two charges when they are at diagonally opposite corners is the diagonal of the square, given by:
r1=√2×a=√2×0.1m=0.1√2m
- The potential energy E1 when the charges are at the diagonal corners is given by:
E1=kQ2r1=k(0.4×10−3)20.1√2
- Where k=14πϵ0≈9×109N m2/C2.
- When one charge is moved to an adjacent vertex, the distance between the two charges becomes:
r2=a=0.1m
- The potential energy E2 when one charge is moved to an adjacent corner is given by:
E2=kQ2r2=k(0.4×10−3)20.1
- The work done in moving the charge is the change in potential energy:
W=E2−E1
- Substitute the expressions for E1 and E2:
W=kQ20.1−kQ20.1√2
- Factor out common terms:
W=kQ2(10.1−10.1√2)=kQ2(10−10/√2)
- Simplifying gives:
W=kQ2(10(1−1√2))
- Now substitute k, Q, and calculate:
W=9×109×(0.4×10−3)2×10(1−1√2)
- Calculate 1−1√2≈0.2929:
W≈9×109×0.16×10−6×10×0.2929
- Final calculation:
W≈4219.2J
The amount of work done in moving one of the charges from a diagonally opposite vertex to an adjacent vertex is approximately 4219.2 J.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:MODERN PUBLICATIONChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:PRACTICE PROBLEMS 2
Explore 21 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:MODERN PUBLICATIONChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:PRACTICE PROBLEMS 3
Explore 3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:MODERN PUBLICATIONChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:Chapter Practice Test
Explore 9 Videos
- ELECTROMAGNETIC WAVESBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC WAVESExercise:CHAPTER PRACTICE TEST
Explore 14 Videos
- MAGNETISM AND MATTERBook:MODERN PUBLICATIONChapter:MAGNETISM AND MATTERExercise:CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
Explore 16 Videos
Similar Questions
Four identical charges q are kept on each vertices of a square of side a. Calculate the extra amount of work done in bringing a Q charge from infinity to the centre of the square.
View SolutionTwo point charges −0.1μCand0.2μC are placed on diagonally opposite vertices of a square of side 15 cm. Locate a point P on the diagonal, where electric potential is zero. Consider the potential infinity to be zero.
View SolutionTwo identical charge of magnitude +2 μCare kept on two diagonally opposite vertices of a square of side a. Both the charges are joined using an insulated massless chord. What will be the tension in the chord? Neglect the gravitational forces involved.
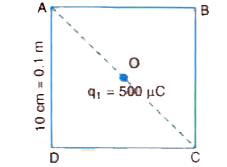
View SolutionA500μC charge is at the centre of a square of side 10 cm. Find the work done in moving a charge of 10μC between two diagonally opposite points on the square.
View SolutionThree identical point charges q are kept on each vertices of an equilateral triangle of side a. Calculate the work done in slowly moving the charges to the mid points of each side, covering the shortest possible distance.
View SolutionA 500 μ C charge is at the centre of a square of side 10 cm. Find the work done in moving a charge of 10 μ C between two diagonally opposite points on the square.
View SolutionTwo similar particles of mass m and charge q are kept on the vertices of an equilateral triangle of side l. Calcualte the work done in moving another particle of charge Q from the third vertex to the centroid of the given triangle.
View SolutionA charge of 5μC is placed at the center of a square ABCD of side 10cm. Find the work done (inμJ) in moving a charge of 1μC from A to B.
View SolutionTwo charges of 5μC and 20μC are placed 20 cm apart. Calculate the work done in bringing the charges closer to 15 cm apart.
View SolutionTwo charges +0.4μC and−0.2μC are placed on adjacent vertices of a square of side 10 cm. Another charge of 1.2μC is placed at the centre af the square Calculate the force and its direction in the charge kept in the centre .
View Solution