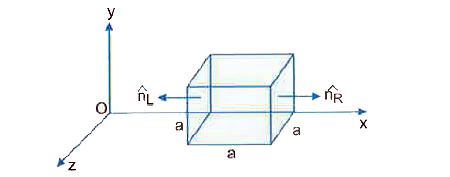
In Fig, electric field is directed along +X direction and is given by Ex=5Ax+2B, where E is in NC−1 and x is in meter, A and B are constants having dimensions. Taking A=10NC−1m−1andB=5NC−1, calculate (i) the electric flux through the cube and (ii) net charge enclosed within the cube.
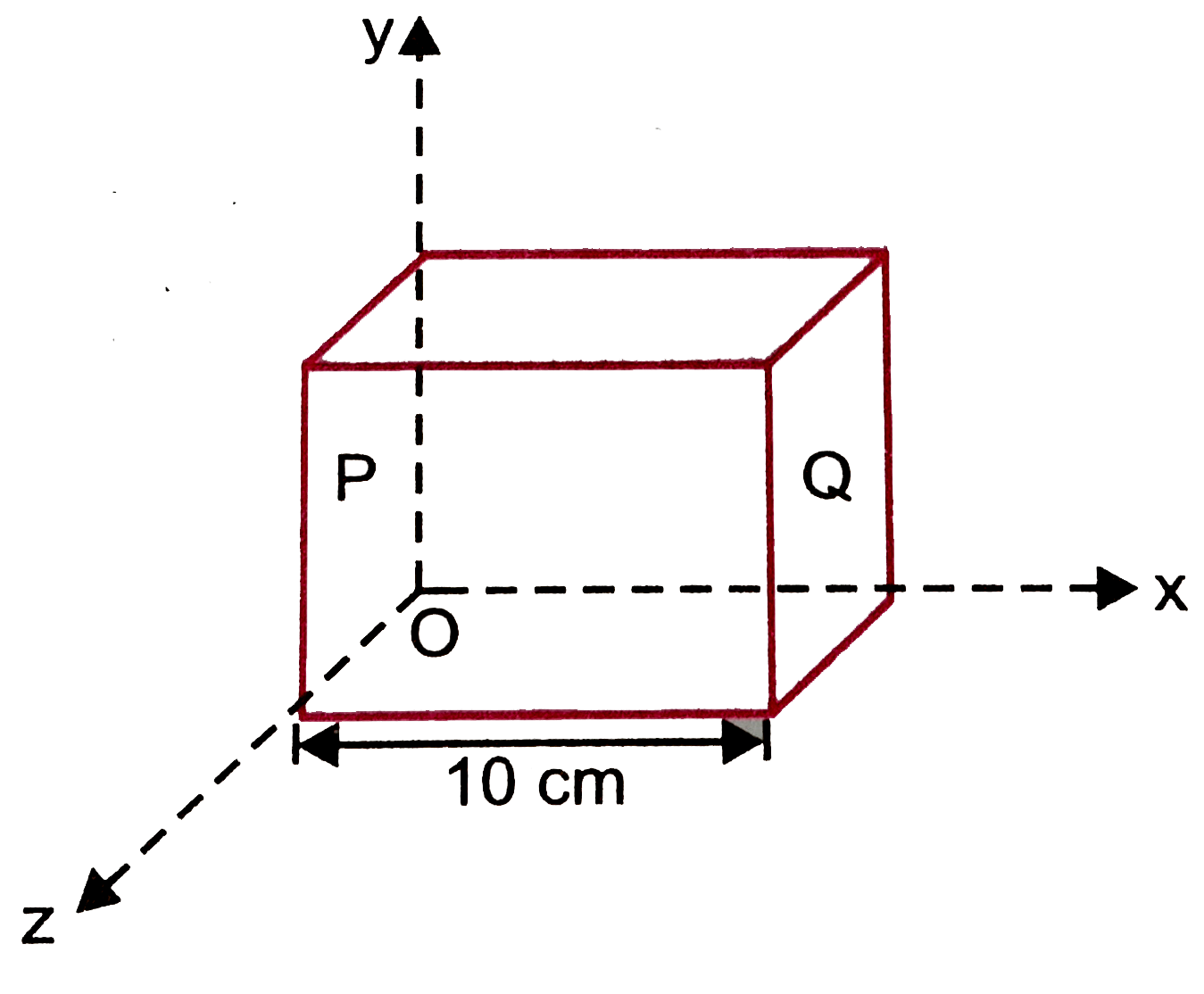
(i)The electric field is along X-axis, thus electric flux through all the surfaces except M and N will be zero
Electric field through surface M
EM=5Ax+2B
⇒EM=5×10×0+10=10NC−1
Electric flux through surface M
ϕM=EMAcos180∘=10×(0.1)2×(−1)
=−0.1Nm2C−1
Electric field through surface N
EN=5Ax+2B
⇒EN=5×10×0.1+10=15NC−1
Electric flux through surface N
ϕN=ENAcos0∘=15×(0.1)2×(1)
=0.15Nm2C−1
Net flux through the cube is 0.15 -0.1
=0.05Nm2C−1
(ii) Net charge enclosed within the cube is
q=ϕε0
⇒q=0.05×8.85×10−12C=0.4425×10−12 C
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Revision Exercises (Numerical Problems )
7 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - COMPETITION FILE ( Objective Question (A.MCQ) )
56 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Revision Exercises (Short Answer )
38 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
Similar Questions
In Fig, electric field is dirceted along +X direction and is given by Ex=5Ax+2B, where E is in NC−1 and x is in meter, A and B are constants having dimenstions. Taking A=10NC−1m−1andB=5NC−1, calculate (i) the electric flux through the cube and (ii) net charge enclosed within the cube.

View SolutionGiven the electric field in the region →E=2ξ, find the net electric flux through the cube and enclosed by it.

View Solution
Knowledge Check
In the given electric field →E=[α(d+x)ˆi+E0ˆj]NC−1, where α=1NC−1 hypothetical closed surface is taken as shown in figure .
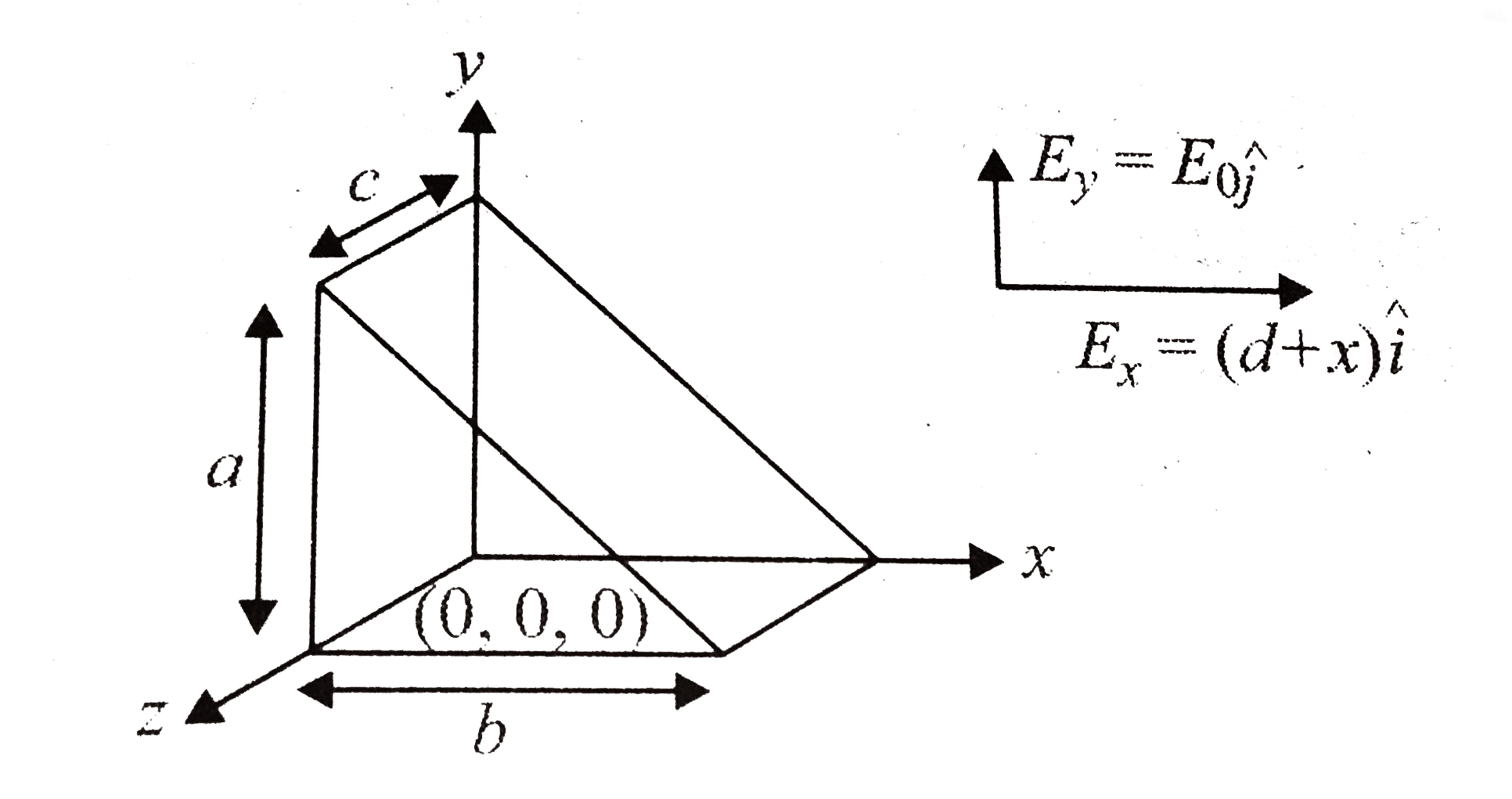
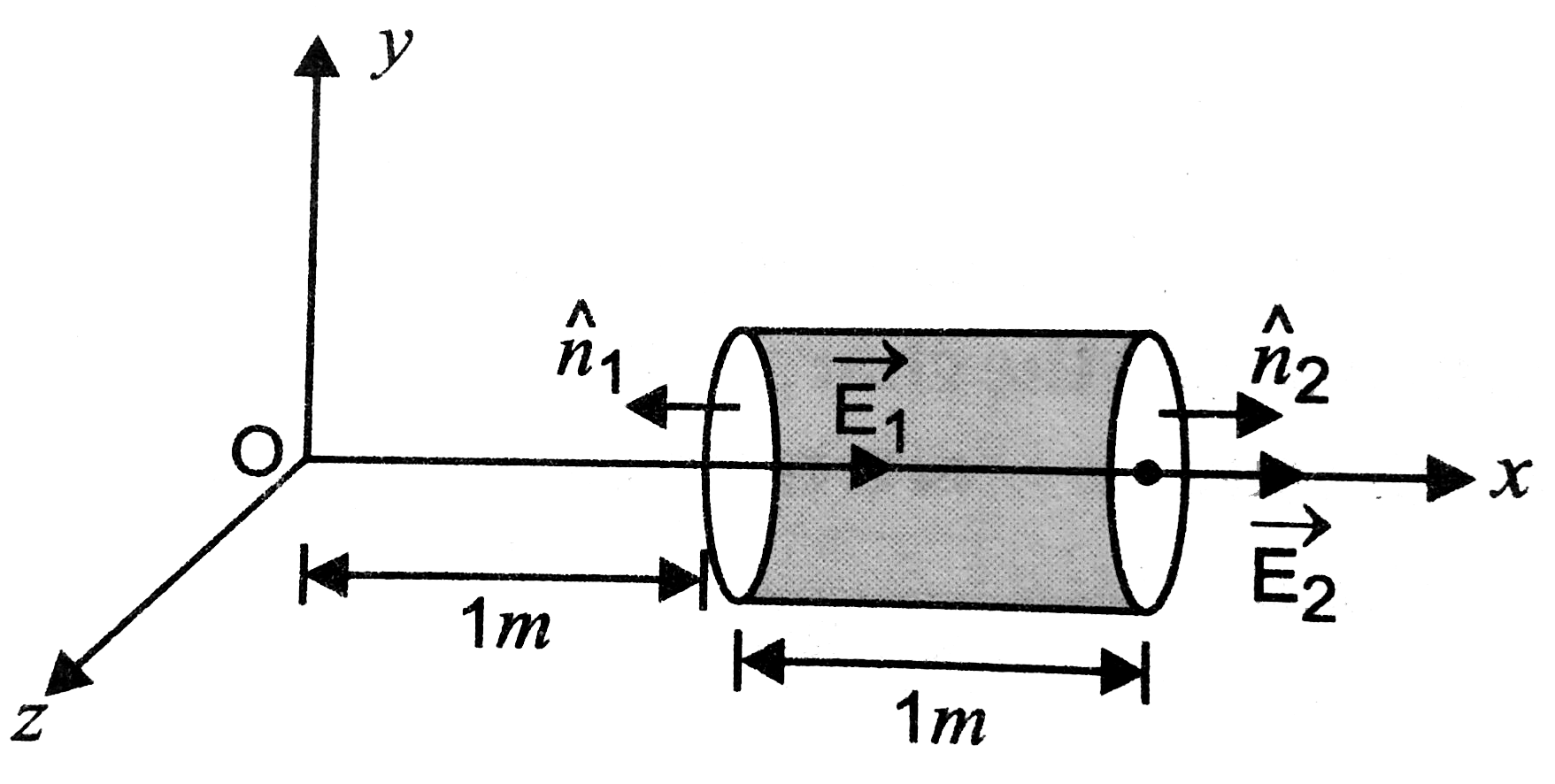
The total charge enclosed within the close surface isAabcε02Bacdε02Cabdε02Dnone of theseA hollow cylindrical box of length 1m and area of cross section 25cm2 is placed in a three dimensional co-ordinate system as shown in Fig, The electric field in the
region is given by →E=50xˆi , where E is in NC−1 and x is in meter. Find
(i) Net flux through the cylinder
(ii) Charge enclosed by the cylinder.
 A(i) 0.625Nm2/C , (ii) 3.506×10−12CB(i) 0.125Nm2/C , (ii) 1.106×10−12CC(i) 0.330Nm2/C , (ii) 2.550×10−12CD(i) 2.125Nm2/C , (ii) 6.106×10−12C
A(i) 0.625Nm2/C , (ii) 3.506×10−12CB(i) 0.125Nm2/C , (ii) 1.106×10−12CC(i) 0.330Nm2/C , (ii) 2.550×10−12CD(i) 2.125Nm2/C , (ii) 6.106×10−12C
If the electric field is given by →E=8ˆi+4ˆj+3ˆkNC−1, calculate the electric flux through a surface of area 100m2 lying in X-Y plane.
View SolutionThe electric field componets due to a charge inside the cube of side 0.1m are Ex=αx, where α=500(N/C)m−1,
Ey=0,Ez=0. Calculate the flux through the cube and the charge inside the cube.
View SolutionUsing Gauss’s law, derive expression for intensity of electric field at any point near the infinitely long straight uniformly charged wire.
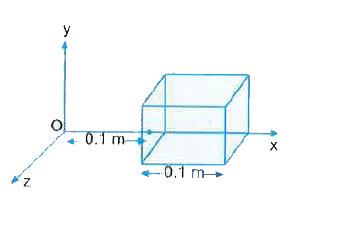
The electric field components in the following figure are Ex=αx,Ey=0,Ez=0, in which α=400N/C m. Calculate (i) the electric flux through the cube, and (ii) the charge within the cube assume that a = 0.1m.
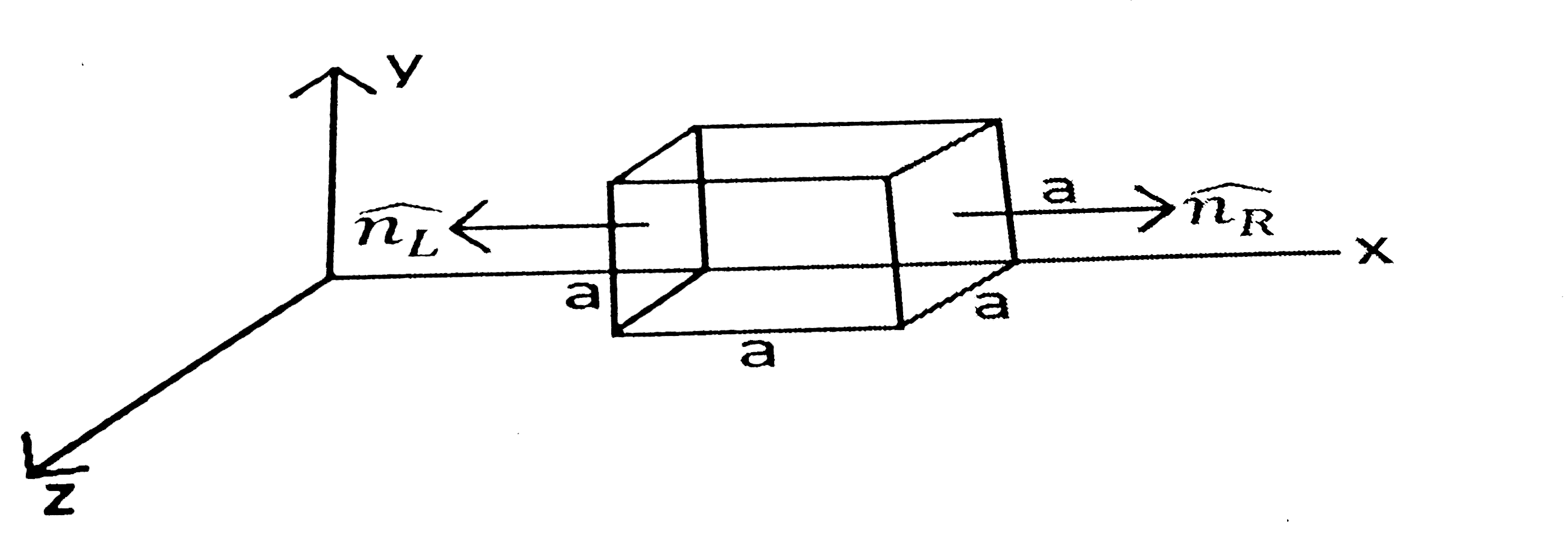
View Solution(a) Using Gauss's law, derive expression for intensity of electric field at any point near the infinitely long straight uniformly charged wire.
(b) The electric field components in the following figure are Ex=αx,Ey=0,Ez=0, in which α=400N/Cm. Calculate (i) the electric flux through the cube, and (ii) the charge within the cube assume that a=0.1m.
View SolutionDefine electric flux. Write its SI units.
(b) The electric field components due to a charge inside the cube of side 0.1m area as shown in Ex=αx, where α=500N/Cm,EY=0andEZ=0.
Calculate (i) the flux through the cube , and
(ii) the charges inside the cube.
View SolutionThe electric field in an electromagnetic wave is given by: E=(50NC−1)sinω(t−xc)F∈dthe∫ensity(∈W/m^2 ) of the wave.
View Solution
