A positive charge Q is fixed at a point in space. There is another charge -q, which moves in a circular path of radius r around the given fixed charge . Assume that the electrostatic force between them is the only force acting on them. Calculate time period of revolution of charge .
To find the time period of revolution of the charge -q around the fixed charge Q, we can follow these steps:
Step 1: Identify the Forces Acting on the Charge
The only force acting on the charge -q is the electrostatic force due to the charge Q. This force can be described by Coulomb's Law:
F=k⋅|Q⋅(−q)|r2
where k is Coulomb's constant, Q is the positive charge, q is the negative charge, and r is the distance between the charges.
Step 2: Set Up the Centripetal Force Equation
Since the charge -q is moving in a circular path, the electrostatic force will provide the necessary centripetal force to keep it in circular motion. The centripetal force can be expressed as:
Fc=mv2r
where m is the mass of the charge -q and v is its tangential velocity.
Step 3: Equate the Electrostatic Force and Centripetal Force
Setting the electrostatic force equal to the centripetal force, we have:
k⋅|Q⋅(−q)|r2=mv2r
This simplifies to:
k⋅Q⋅qr2=mv2r
Step 4: Solve for Velocity
Rearranging the equation to solve for v2:
k⋅Q⋅q=mv2⋅r
v2=k⋅Q⋅qm⋅r
Step 5: Relate Velocity to Angular Velocity
The tangential velocity v can be related to the angular velocity ω by the equation:
v=ωr
Substituting this into our equation gives:
(ωr)2=k⋅Q⋅qm⋅r
This simplifies to:
ω2r2=k⋅Q⋅qm⋅r
Step 6: Solve for Angular Velocity
Rearranging for ω2:
ω2=k⋅Q⋅qm⋅r3
Taking the square root:
ω=√k⋅Q⋅qm⋅r3
Step 7: Find the Time Period
The time period T is related to angular velocity by the formula:
T=2πω
Substituting for ω:
T=2π√m⋅r3k⋅Q⋅q
Final Result
Thus, the time period of revolution of the charge -q around the fixed charge Q is:
T=2π√m⋅r3k⋅Q⋅q
The only force acting on the charge -q is the electrostatic force due to the charge Q. This force can be described by Coulomb's Law:
F=k⋅|Q⋅(−q)|r2
where k is Coulomb's constant, Q is the positive charge, q is the negative charge, and r is the distance between the charges.
Since the charge -q is moving in a circular path, the electrostatic force will provide the necessary centripetal force to keep it in circular motion. The centripetal force can be expressed as:
Fc=mv2r
where m is the mass of the charge -q and v is its tangential velocity.
Setting the electrostatic force equal to the centripetal force, we have:
k⋅|Q⋅(−q)|r2=mv2r
This simplifies to:
k⋅Q⋅qr2=mv2r
Rearranging the equation to solve for v2:
k⋅Q⋅q=mv2⋅r
v2=k⋅Q⋅qm⋅r
The tangential velocity v can be related to the angular velocity ω by the equation:
v=ωr
Substituting this into our equation gives:
(ωr)2=k⋅Q⋅qm⋅r
This simplifies to:
ω2r2=k⋅Q⋅qm⋅r
Rearranging for ω2:
ω2=k⋅Q⋅qm⋅r3
Taking the square root:
ω=√k⋅Q⋅qm⋅r3
The time period T is related to angular velocity by the formula:
T=2πω
Substituting for ω:
T=2π√m⋅r3k⋅Q⋅q
Thus, the time period of revolution of the charge -q around the fixed charge Q is:
T=2π√m⋅r3k⋅Q⋅q
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS Book:MODERN PUBLICATIONChapter:ELECTRIC CHARGES AND FIELDS Exercise:Practice Problems
Explore 88 Videos
- ELECTRIC CHARGES AND FIELDS Book:MODERN PUBLICATIONChapter:ELECTRIC CHARGES AND FIELDS Exercise:Conceptual Questions
Explore 51 Videos
- DUAL NATURE OF RADIATION AND MATTER Book:MODERN PUBLICATIONChapter:DUAL NATURE OF RADIATION AND MATTER Exercise:CHAPTER PRACTICE TEST
Explore 15 Videos
- ELECTROMAGNETIC INDUCTIONBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC INDUCTIONExercise:CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
Explore 14 Videos
Similar Questions
Knowledge Check
A charge moves with a speed v in a circular path of radius r around a long uniformly charged conductor.
Av∝rBv∝1rCv∝1√rDv∝r0A particle of charge −q and mass m moves in a circular orbits of radius r about a fixed charge +Q.The relation between the radius of the orbit r and the time period T is
Ar=Qq16π2ε0MT3Br3=Qq16π2ε0MT2Cr2=Qq16π3ε0mT3Dr2=Qq16π3ε0mT3A point charge Q is moved along a ciruclar path around another fixed point charge. The work done is zero
Aonly if Q returns to its starting pointBonly if the twoi charges have the same magnitudeConly if the two charges have the same magnitude and opposite signsDin all case
A charged metallic sphere A having charge qA is brought in contact with an uncharged metallic sphere of same radius and then separated by a distance d. What is the electrostatic force between them
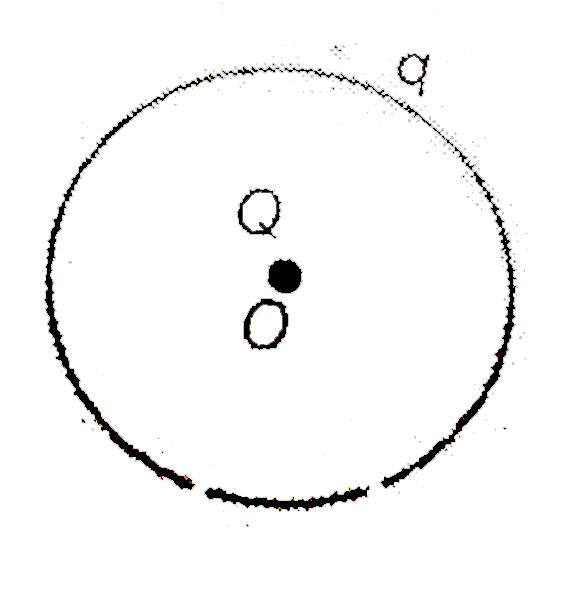
View SolutionA point charge Q is placed at the centre of a circular wire of radius R having charge q. Find the force of electrostatic interaction between point charge and the wire.
View SolutionA positive charge 'Q' is fixed at a point A negatively charged particle of mass ' 'm' and charge 'q' is revolving in a circular path of radius r1 ,with Q' as the centre.The change in potential energy to change the radius of the circular path from r1 to r2 in joule is
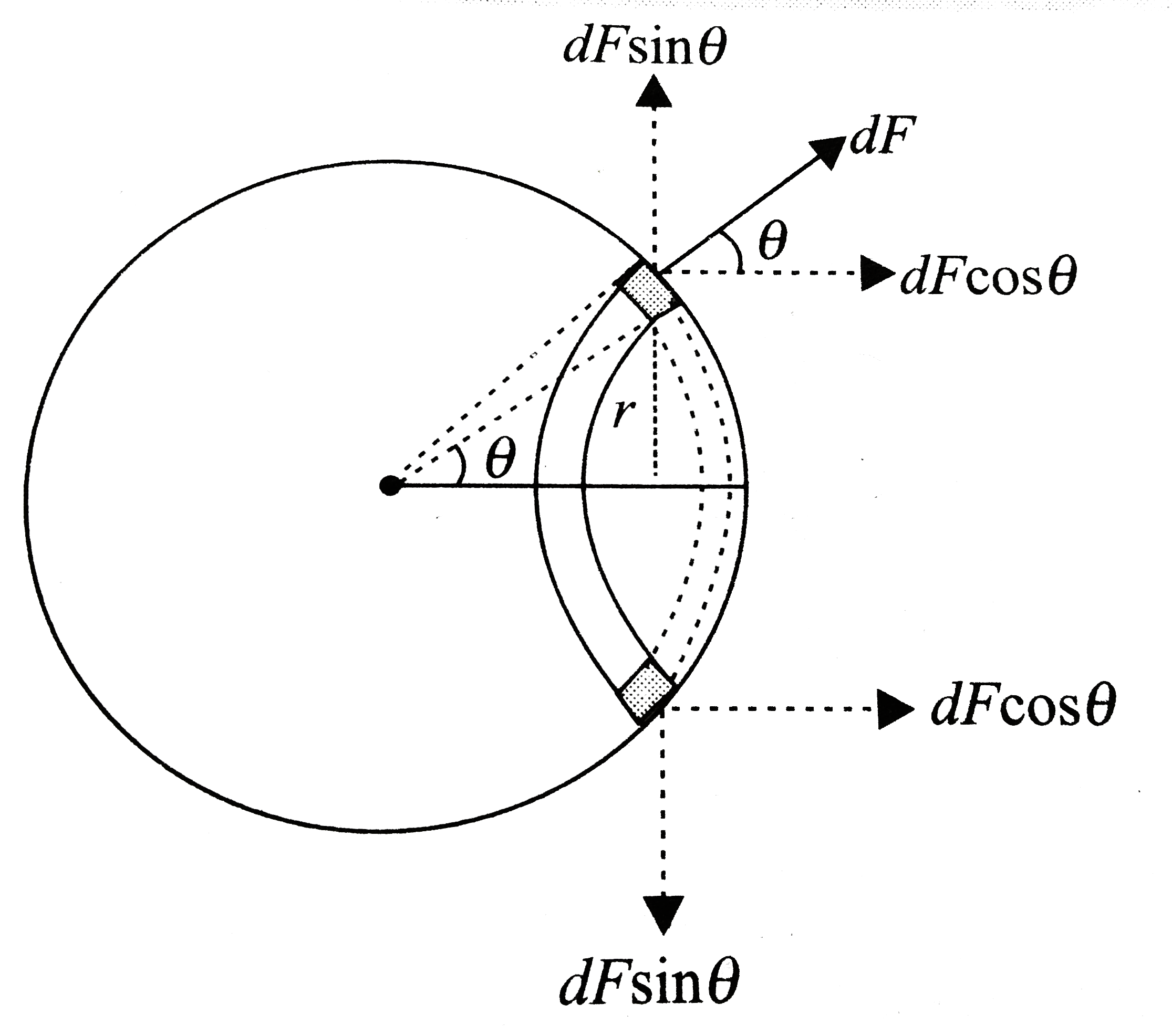
View SolutionFind the electrostatic force of interaction between two halves of a spherical conductor of radius R carrying a charge Q.
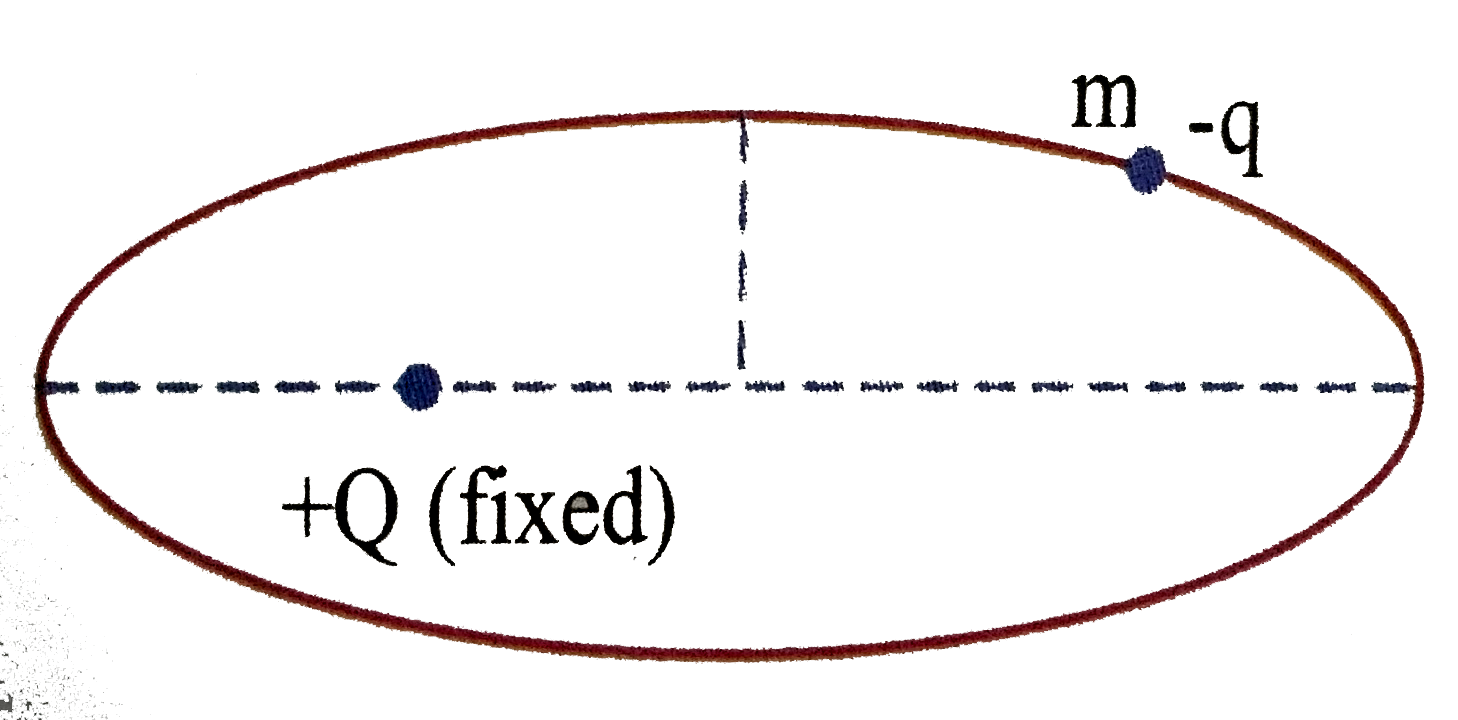
View SolutionA positive point charge +Q is fixed in space .A negative point charge −q of mass m revolves around a fixed charge in elliptical orbits .The fixed charge +Qis at one focus of the ellipse.The only force acting on negative charge is the electrostatic force due to positive charge is the electrostatic force due ot positive charge.Then which of the following statement is true
View Solution
