A positive point charge +Q is fixed in space .A negative point charge −q of mass m revolves around a fixed charge in elliptical orbits .The fixed charge +Qis at one focus of the ellipse.The only force acting on negative charge is the electrostatic force due to positive charge is the electrostatic force due ot positive charge.Then which of the following statement is true
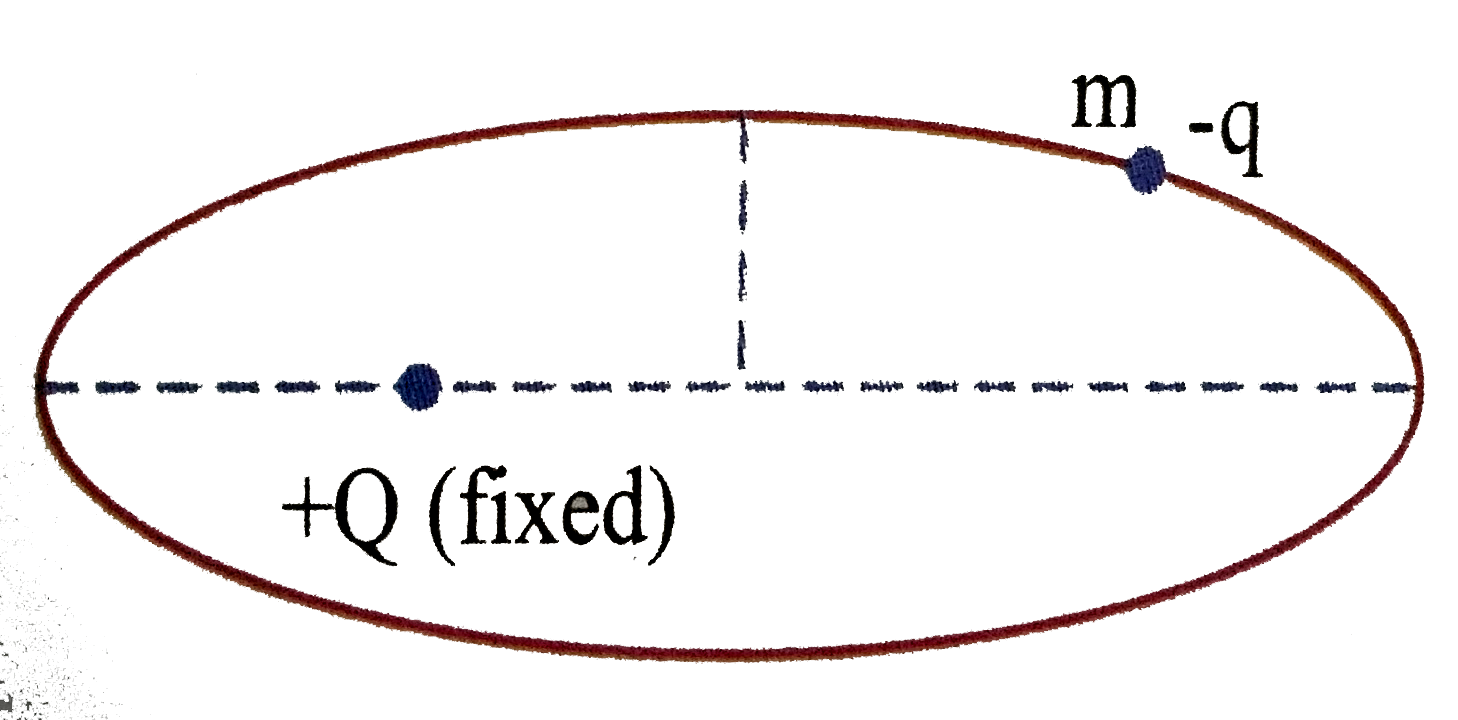
Linear momentum of negative point charge is conserved
Angular momentum of negative point charge about fixed positve charge is conserved
Total Kinetic energy of negative point charge is conserved.
Electrostatic potential energy of system of both point charge is conserved.
The correct Answer is:B
Only angular momentum x & total energy are conserved but not kinetic energy.
Topper's Solved these Questions
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Passage I
3 Videos
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Passage II
3 Videos
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - NCERT based Question
14 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - ADDITIONAL PROBLEMS
14 Videos
- EXPERIMENTAL PHYSICSBOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Comprehension type
6 Videos
Similar Questions
A positive charge Q is fixed at a point in space. There is another charge -q, which moves in a circular path of radius r around the given fixed charge . Assume that the electrostatic force between them is the only force acting on them. Calculate time period of revolution of charge .
View SolutionState superposition for electrostatic force on a charge due to a number of charges.
View Solution
Knowledge Check
A charge +q is fixed to each of three corners of a square .On the empty corner a charge Q is placed such that there is no net electrostatic force acting on the diagonally opposite charge then
AQ=−2qBQ=−2√2qCQ=−√2qDQ=−4qNegative charge is revolving around is fixed positive charge in a circular orbit If the classical idea of an accelerating charge rediating energy is valid, then the negative charge will:
Aspiral towards the positive charge, with increasing kinetic energyBspiral towards the positive charge with potential energy decreasing at a faster rate then increase in its kinetic energy.Cspiral away from the positive charge and finally escape from the binding of the positive chargesDrevolve around the positive charge with increasing frequency of revolution.Two equal negative charges are fixed at points and on y-axis. A positive charge Q is released from rest at the point on the x-axis. The charge Q will:
Aexecute simple harmonic motion about the originBmove to the origin and remain at restCmove to infinityDsame as at point 25 cm aways form the surface
Two positive point charges A and B have charge +q and +2q; mass m and 2m respectively. Both the charges are released from rest when they are at a distance l0 apart. Neglect gravity and also assume the only force acting on either charge is the electrostatic force due to each other. The speed of charge A at the instant separation between both charges is 2l0
View SolutionA positive point charge q1 is placed at (a,0) and another positive point charge q2 is placed at (0,a) . Find the force acting on q1.
View SolutionPositive point charges are placed at the vertices of a star shape as shown in the figure. Direction of the electrostatic force on a negative point charge at the centre O of the star is
View SolutionTwo point charges of same magnitude and opposite sign are fixed at point a and B. A third small point charge is to be balanced at point P by the electrostatic force due to these two charges. The point P :

View SolutionA point charge Q is moved along a ciruclar path around another fixed point charge. The work done is zero
View Solution
